The moment of inertia of an elliptical disc of uniform mass distribution of mass 'm' major axis 'r', minor axis 'd' about its axis is :
=mr22
=md22
>mr22
<mr22
The correct Answer is:D
To find the moment of inertia of an elliptical disc of uniform mass distribution with mass 'm', major axis 'R', and minor axis 'd' about its axis, we can follow these steps:
1. Understanding the Geometry:
- An elliptical disc has a major axis (R) and a minor axis (d). The semi-major axis is a=R2 and the semi-minor axis is b=d2.
2. Moment of Inertia of a Circular Disc:
- The moment of inertia I of a circular disc about its central axis is given by the formula:
I=12mr2
- Here, r is the radius of the circular disc.
3. Relating Elliptical Disc to Circular Disc:
- To find the moment of inertia of the elliptical disc, we can compare it to a circular disc.
- The moment of inertia of an elliptical disc can be derived by considering it as a collection of infinitesimally thin circular discs stacked along the minor axis.
4. Calculating Moment of Inertia for the Elliptical Disc:
- The moment of inertia about the major axis (R) can be expressed as:
Imajor=12ma2
- The moment of inertia about the minor axis (d) can be expressed as:
Iminor=12mb2
5. Final Expression for Moment of Inertia:
- For the elliptical disc, the moment of inertia about its axis can be approximated as:
Iellipse=14m(R2+d2)
- This formula accounts for the distribution of mass in both axes.
6. Conclusion:
- Therefore, the moment of inertia of an elliptical disc of uniform mass distribution about its axis is:
I=14m(R2+d2)
- An elliptical disc has a major axis (R) and a minor axis (d). The semi-major axis is a=R2 and the semi-minor axis is b=d2.
- The moment of inertia I of a circular disc about its central axis is given by the formula:
I=12mr2
- Here, r is the radius of the circular disc.
- To find the moment of inertia of the elliptical disc, we can compare it to a circular disc.
- The moment of inertia of an elliptical disc can be derived by considering it as a collection of infinitesimally thin circular discs stacked along the minor axis.
- The moment of inertia about the major axis (R) can be expressed as:
Imajor=12ma2
- The moment of inertia about the minor axis (d) can be expressed as:
Iminor=12mb2
- For the elliptical disc, the moment of inertia about its axis can be approximated as:
Iellipse=14m(R2+d2)
- This formula accounts for the distribution of mass in both axes.
- Therefore, the moment of inertia of an elliptical disc of uniform mass distribution about its axis is:
I=14m(R2+d2)
Topper's Solved these Questions
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE C
10 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE D
10 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE A
10 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question
46 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - IMPECCABLE
56 Videos
Similar Questions
Find the out the moment of inertia of a ring having uniform mass distribution of mass M and radius R about an axis which is tangent ot the ring and a in the plane of the ring b. perpendicular to the plane of the ring.
View SolutionThe moment of inertia of a ring of mass M and radius R about PQ axis will be

View SolutionWhat is the moment of inertia of a
(i) uniform circular ring of mass 2 kg about its 4 m diameter ?
(ii) a thin disc about an axis coinciding with a diameter ?
View SolutionThe moment of inertia of a disc of mass M and radius R about a tangent to its rim in its plane is
View SolutionThe moment of inertia of a thing uniform rod of length L and mass M, about axis CD (as shown in figure) is

View SolutionCalculate the moment of inertia of a ring having mass M, radius Rand having uniform mass distribution about an axis passing through the centre of the ring and perpendicular to the plane of the ring?
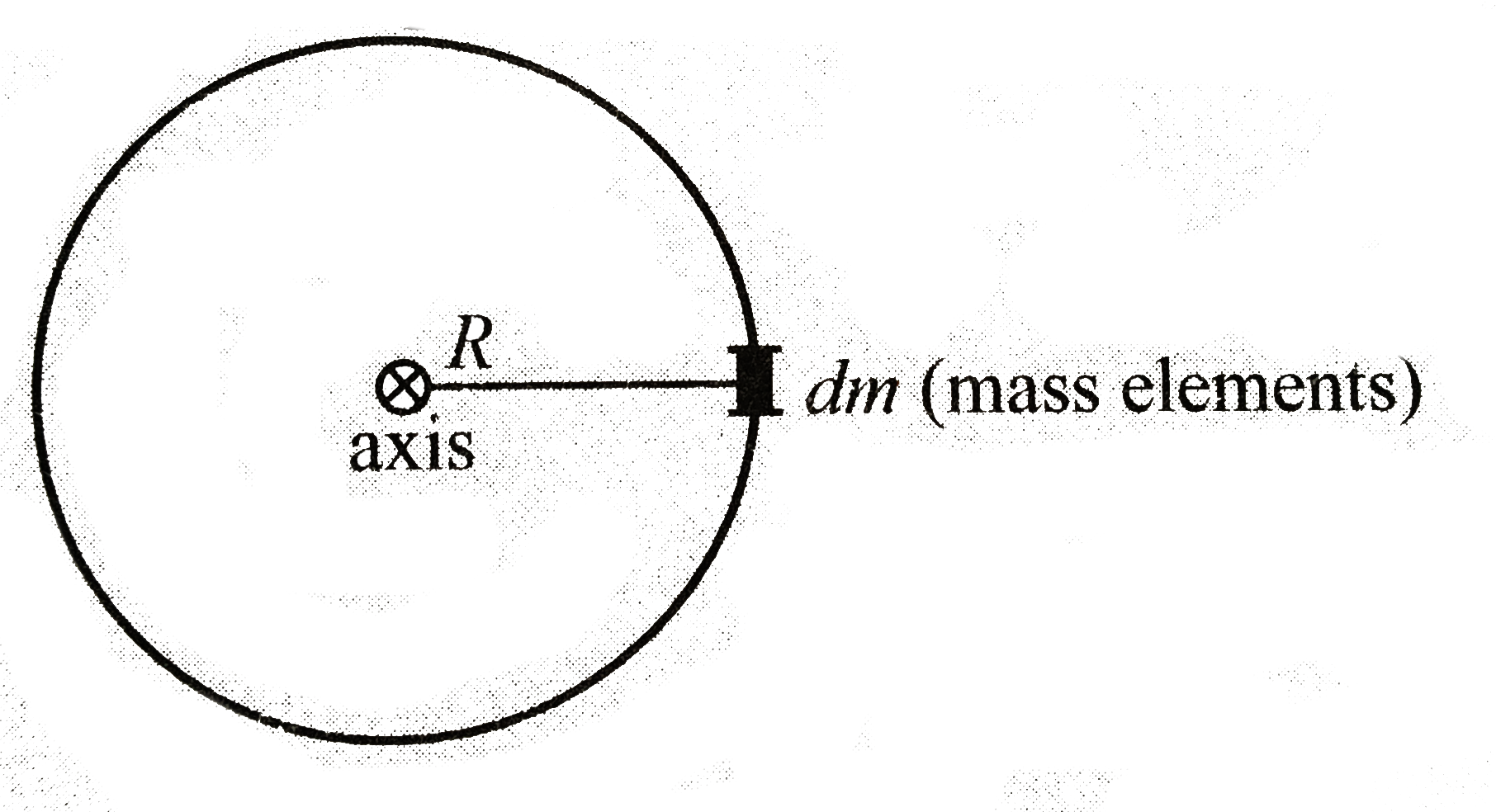
View SolutionThe moment of inertia of a uniform semicircular wire of mass m and radius r, about an axis passing through its centre of mass and perpendicular to its plane is mr2(−kπ2). Find the value of k.
View SolutionMoment of inertia of an ellipse shaped wire of mass 'm', semimajor axis 'a' about an axis passing through the centre and perpendicular to the plane of wire can be :
View SolutionThe moment of inertia of thin uniform circular disc about one of the diameter is I. Its moment of inertia about an axis perpendicular to the circular surface and passing through its centre is
View SolutionCalculate the moment of inertia of a rectangular frame formed by uniform rods having mass m each as shown in about an axis passing through its centre and perpendicular to the plane of frame. Also find moment of inertia about an axis passing through PQ ?

View Solution
