A bar magnet having centre O has a length of 4cm. Point P1 is in the broad side-on and P2 is in the end side-on position with OP1=OP2=10metres. The ratio of magnetic intensities H at P1 and P2 is
H1:H2=16:100
H1:H2=2:1
H1:H2=1:2
H1:H2=100:16
The correct Answer is:2
To find the ratio of magnetic intensities H at points P1 (broad side-on position) and P2 (end side-on position) of a bar magnet, we can use the formulas for magnetic field intensity in both positions.
1. Identify the Positions:
- Point P1 is in the broad side-on position (equatorial position).
- Point P2 is in the end side-on position (axial position).
- The distance from the center of the magnet O to both points is OP1=OP2=10m.
2. Length of the Magnet:
- The length of the bar magnet is given as 4cm which is 0.04m.
3. Magnetic Field Intensity Formulas:
- For a bar magnet, the magnetic field intensity H at a point in the axial position (end side) is given by:
Hend=μ0⋅2⋅mr3
- For a point in the equatorial position (broad side), the magnetic field intensity is:
Hbroad=μ0⋅mr3
Where μ0 is the permeability of free space and m is the pole strength of the magnet.
4. Calculate the Magnetic Intensities:
- For P1 (broad side):
HP1=μ0⋅m(10)3=μ0⋅m1000
- For P2 (end side):
HP2=μ0⋅2⋅m(10)3=2μ0⋅m1000
5. Find the Ratio:
- The ratio of magnetic intensities HP1HP2 is:
HP1HP2=μ0⋅m10002μ0⋅m1000=12
6. Final Result:
- Therefore, the ratio of magnetic intensities at points P1 and P2 is:
HP1HP2=12
Conclusion:
The ratio of magnetic intensities H at P1 and P2 is 12.
- Point P1 is in the broad side-on position (equatorial position).
- Point P2 is in the end side-on position (axial position).
- The distance from the center of the magnet O to both points is OP1=OP2=10m.
- The length of the bar magnet is given as 4cm which is 0.04m.
- For a bar magnet, the magnetic field intensity H at a point in the axial position (end side) is given by:
Hend=μ0⋅2⋅mr3
- For a point in the equatorial position (broad side), the magnetic field intensity is:
Hbroad=μ0⋅mr3
Where μ0 is the permeability of free space and m is the pole strength of the magnet.
- For P1 (broad side):
HP1=μ0⋅m(10)3=μ0⋅m1000
- For P2 (end side):
HP2=μ0⋅2⋅m(10)3=2μ0⋅m1000
- The ratio of magnetic intensities HP1HP2 is:
HP1HP2=μ0⋅m10002μ0⋅m1000=12
- Therefore, the ratio of magnetic intensities at points P1 and P2 is:
HP1HP2=12
The ratio of magnetic intensities H at P1 and P2 is 12.
Topper's Solved these Questions
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-H
10 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-I
10 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-F
10 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
- PROPERTIES OF MATTERBOOK - VMC MODULES ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced (Archive) Level - 2 (MATRIX MATCH TYPE)
1 Videos
Similar Questions
A short bar magnet has a magnetic moment of 0.48JT−1. Give the direction and magnitude of the magnetic field produced by the magnet at a distance of 10cm form the center of magnet on (i) the axis (ii) the equatorial line (normal bisector) of the magnet.
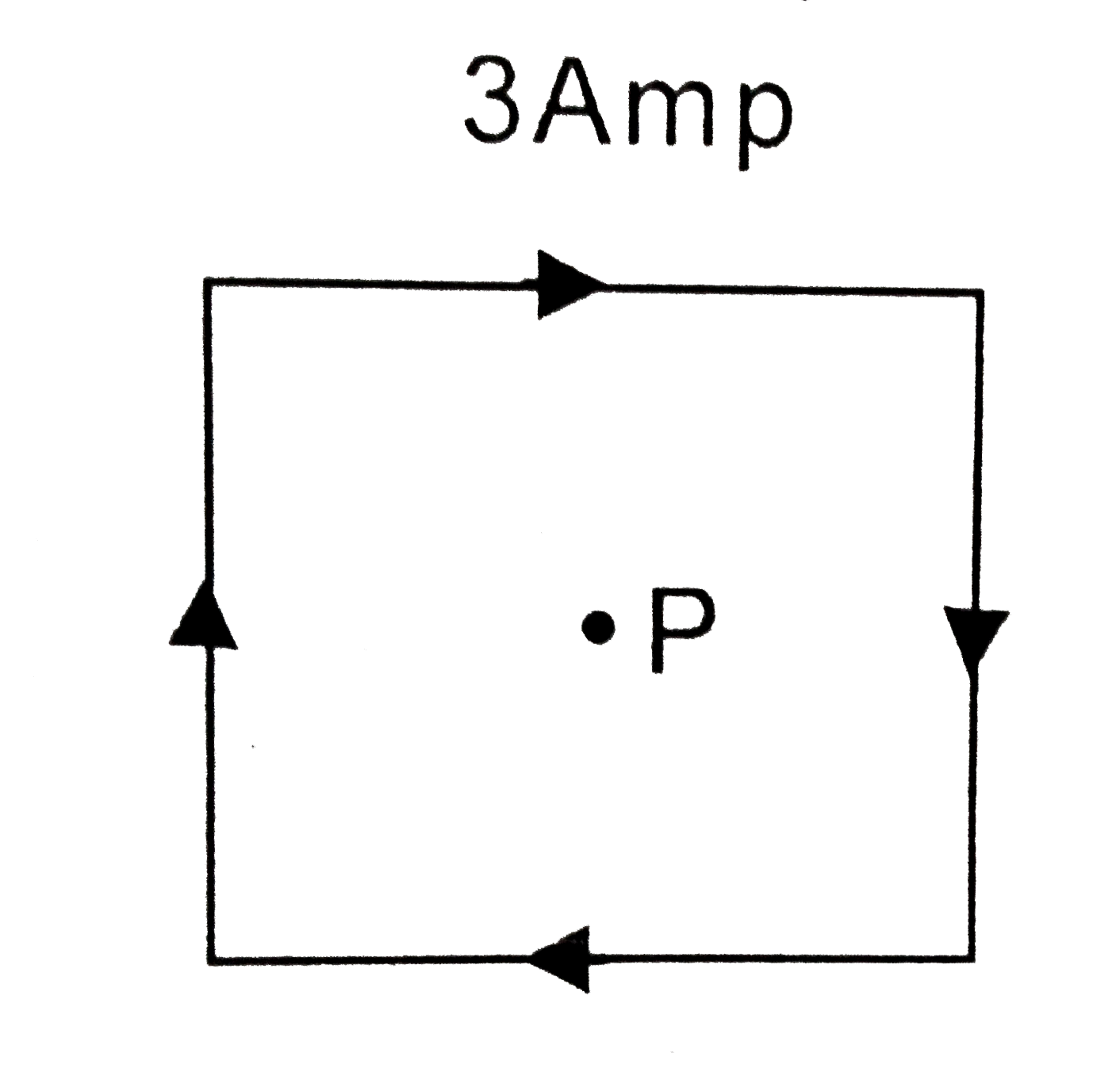
View SolutionFind magnetic field at centre P if length of side of square loop is 20 cm.
View SolutionAP is tangent to circle O at point P, What is the length of OP?
View SolutionThe ratio of magnetic fields due to a bar magnet at the two axial points P1andP2 which are separated from each other by 10 cm is 25 :2 . Point P1 is situated at 10 cm from the centre of the magnet. Magnetic length of the bar magnet is (Points P1andP2 are on the same side of magnet and distance of P2 from the centre is greater than distance of P1 from the centre of magnet)
View SolutionDue to a small magnet intensity at a distance x in the end on position is 9 Gauss. What will be the intensity at a distance x2 on broad side on position?
View SolutionConsider a bar magnet having pole strength 2A-m, magnetic length 4cm and area of cros-section 1cm2 Find
(I) the magnetisation I
(II) the magneic intensity H and
(III) the magnetic field at the centre of magnet
View SolutionWrite an expression (derivation not required) for intensity of electric field in :
(1) Axial position.
(2) Broad side position of an electric dipole, in terms of its length (2a) dipole moment (p) and distance (r).
(ii) What is the ratio of these two intensities i.e., E1:E2, for a short electric dipole ?
View SolutionAn organ pipe P1 open at one end vibrating in its first harmonic and another pipe P2 open at ends vibrating in its third harmonic are in resonance with a given tuning fork. The ratio of the length of P1 to that P2 is
View Solution(a) Using the phenomenon of polarization, show, how, transverse nature of light can be demonstrated.
(b) Two polaroids P1andP2 are placed with their pass axes perpendicular to each other. Unpolarised light of intensity 10 is incident on P1. A third polaroid P3 is kept in between P1andP2 such that its pass axis makes an angle of 30∘ with that of P1. Determine the intensity transmitted through P1,P2andP3.
View SolutionTwo wires W1andW2 have the same radius r and respective densities p1andp2 such that p2=4p1. They are joined together at the point O, as shown in the figure. The combination is used as a sonometer wire and kept udner tension T. The point O is midway between the two bridges. When a stationary waves is set up in the composite wire, the joint is found to be a node. The ratio of the number of antinodes formed in W1toW2 is-

View Solution
