Starting from origin at t=0, with initial velocity 5ˆjm/s, a particle moves in the x−y plane with a constant acceleration of (10ˆi−5ˆj)m/s2. Find the coordinates of the particle at the moment its y-coordinate is maximum.
(5m, 5m)
(2.5m, 2.5m)
(5m, 2.5m)
(2.5m, 5m)
The correct Answer is:C
To solve the problem step by step, we will use the equations of motion to find the coordinates of the particle when its y-coordinate is maximum.
Step 1: Identify the initial conditions and acceleration
- The initial position of the particle is at the origin:
→r0=0^i+0^j m
- The initial velocity is:
→u=0^i+5^j m/s
- The acceleration is:
→a=10^i−5^j m/s2
Step 2: Write the equations of motion
Using the second equation of motion, the displacement vector →s can be expressed as:
→s=→ut+12→at2
Substituting the values of →u and →a:
→s=(0^i+5^j)t+12(10^i−5^j)t2
This simplifies to:
→s=(0+5t)^j+(5t2)^i−(52t2)^j
Combining the terms, we have:
→s=5t2^i+(5t−52t2)^j
Step 3: Identify the coordinates
From the displacement vector, we can identify the x and y coordinates:
- xf=5t2
- yf=5t−52t2
Step 4: Find the time when y-coordinate is maximum
To find the maximum y-coordinate, we differentiate yf with respect to time t:
dyfdt=5−5t
Setting the derivative equal to zero to find the critical points:
5−5t=0⟹t=1 s
Step 5: Verify that it is a maximum
To confirm that this point is a maximum, we differentiate dyfdt again:
d2yfdt2=−5
Since d2yfdt2<0, this indicates that yf is indeed at a maximum when t=1 s.
Step 6: Calculate the coordinates at t=1 s
Substituting t=1 s into the equations for xf and yf:
- For xf:
xf=5(1)2=5 m
- For yf:
yf=5(1)−52(1)2=5−2.5=2.5 m
Final Coordinates
The coordinates of the particle when its y-coordinate is maximum are:
(xf,yf)=(5 m,2.5 m)
- The initial position of the particle is at the origin:
→r0=0^i+0^j m
- The initial velocity is:
→u=0^i+5^j m/s
- The acceleration is:
→a=10^i−5^j m/s2
Using the second equation of motion, the displacement vector →s can be expressed as:
→s=→ut+12→at2
Substituting the values of →u and →a:
→s=(0^i+5^j)t+12(10^i−5^j)t2
This simplifies to:
→s=(0+5t)^j+(5t2)^i−(52t2)^j
Combining the terms, we have:
→s=5t2^i+(5t−52t2)^j
From the displacement vector, we can identify the x and y coordinates:
- xf=5t2
- yf=5t−52t2
To find the maximum y-coordinate, we differentiate yf with respect to time t:
dyfdt=5−5t
Setting the derivative equal to zero to find the critical points:
5−5t=0⟹t=1 s
To confirm that this point is a maximum, we differentiate dyfdt again:
d2yfdt2=−5
Since d2yfdt2<0, this indicates that yf is indeed at a maximum when t=1 s.
Substituting t=1 s into the equations for xf and yf:
- For xf:
xf=5(1)2=5 m
- For yf:
yf=5(1)−52(1)2=5−2.5=2.5 m
The coordinates of the particle when its y-coordinate is maximum are:
(xf,yf)=(5 m,2.5 m)
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 3EXERCISE - PART I : PHYSICS (SECTION-2)10videos
MOCK TEST 3
- BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 2EXERCISE - PART I : PHYSICS (SECTION - 2)10videos
MOCK TEST 2
- BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 4EXERCISE - PHYSICS (SECTION 2)5videos
MOCK TEST 4
Similar Questions
A particle starts from the origin at t=0 with a velocity of 10.0ˆjm/s and moves in the x-y plane with a constant acceleration of (8.0ˆi+2.0ˆj)ms−2 . (a) At what time is the x-coordinate of the particle 16m ? What is the y-coordinate of the particle at that time ? (b) What is the speed of the particle at that time ?
View SolutionA particle starts from the origin at t=0 with a velocity of 10.0ˆjm/s and moves in the X-y plane with a constant accleration of (8.0ˆI+2.0ˆj)ms−2 . (a) At wht time is the x-coordinate of the particle 16m ? What is the y-coordinate of the particle at that time ? ( b) What is the speed of the particle at that time ?
View SolutionA particle starts from the origin at t = 0 s with a velocity of 10.0ˆj m/s and moves in plane with a constant acceleration of (8ˆi+2ˆj)ms−2. The y-coordinate of the particle in 2 sec is
View SolutionStarting from the origin at time t = 0 , with initial velocity 5ˆjms−1 , a particle moves in the x - y plane with a constant acceleration of (10ˆi+4ˆj)ms−2 . At time t , its coordinates are (20m,y0m) . The value of t and y0 are , respectively :
View SolutionA particle starts from the origin at t=0 with a velocity of 8.0ˆjm/s and moves in the x-y plane with a constant acceleration of (4.0ˆi+2.0ˆj)m/s2. At the instant the particle's x-coordinate is 29 m, what are
(a) its y-coordinate and
(b) its speed ?View SolutionA paarticle starts from origin at t=0 with a velocity 5.0ˆim/s and moves in x-y plane under action of a force which produces a constant acceleration of (3.0ˆi+2.0j)m/s2.
(a) What is the y-cordinate of the particle at the instant its x-coordinate is 84 m ? (b) What is the speed of the particle at this time?View SolutionA particle leaves the origin with initial velocity →v0=11ˆi+14ˆjm/s. It undergoes a constant acceleration given by →a=−225ˆi+215ˆjm/s2.
When does the particle cross the y-axis ?View SolutionA particle moves with a tangential acceleration at=→a.ˆv where →a=(5ˆi)m/s2. If the speed of the particle is zero at x=0, then find v (in m/s) at x=4.9m.
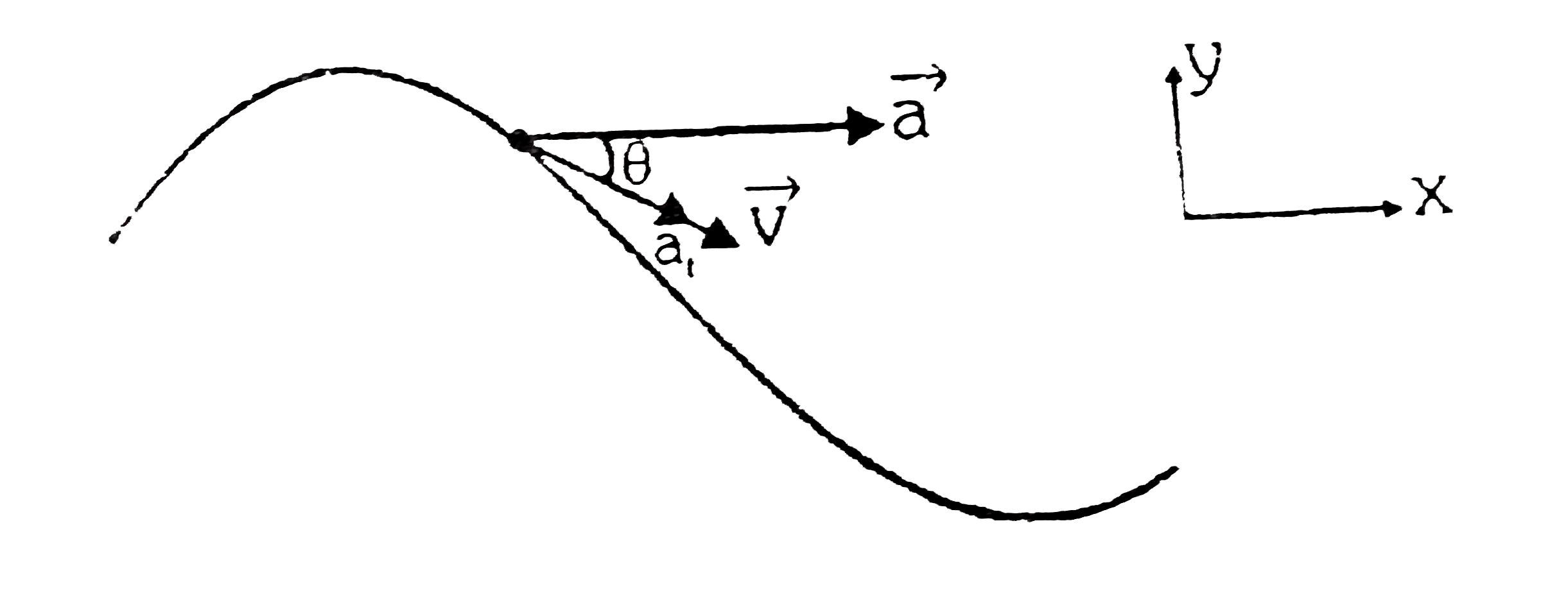 View Solution
View SolutionA particle is moving in x-y plane. At an instant, it has velocity (4ˆi+4ˆj)m/s and acceleration (3ˆi+5ˆj)m/s2 At that instant, the radius of curvature of its path will be :
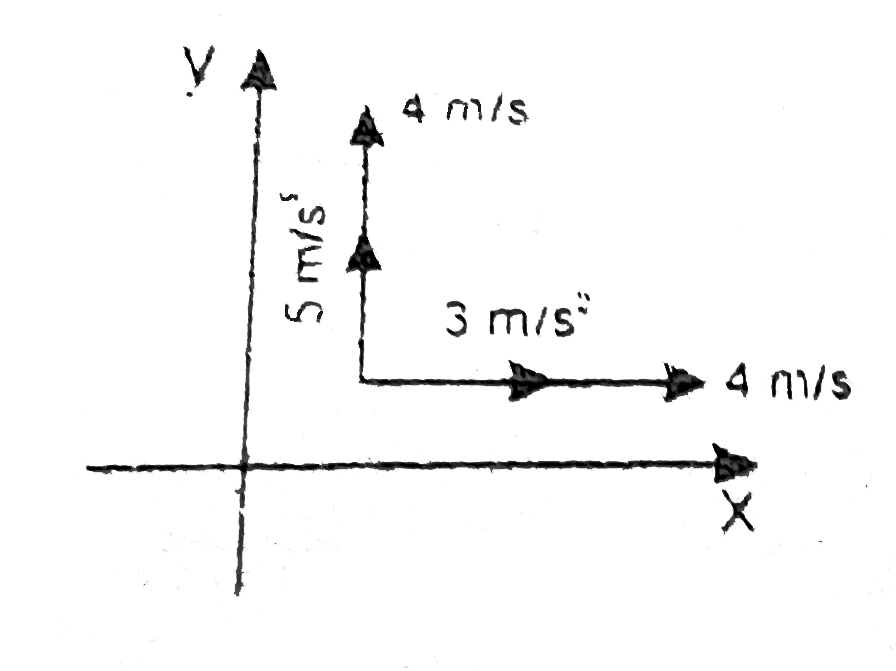 View Solution
View SolutionA particle has initial velocity of (3ˆi+4ˆj)m/s and a acceleration of (4ˆi−3ˆj)m/s2. Its speed after one second will be equal to :-
View Solution

