Question

Number of solutions $2^{\sin \left(\left|x\right|\right)}=4^{\left|\cos x\right|}\in [-\pi ,\pi ]$ is equal to

Options

A. 2
B. 4
C. 6
D. None of these

Answer

B

Explanation

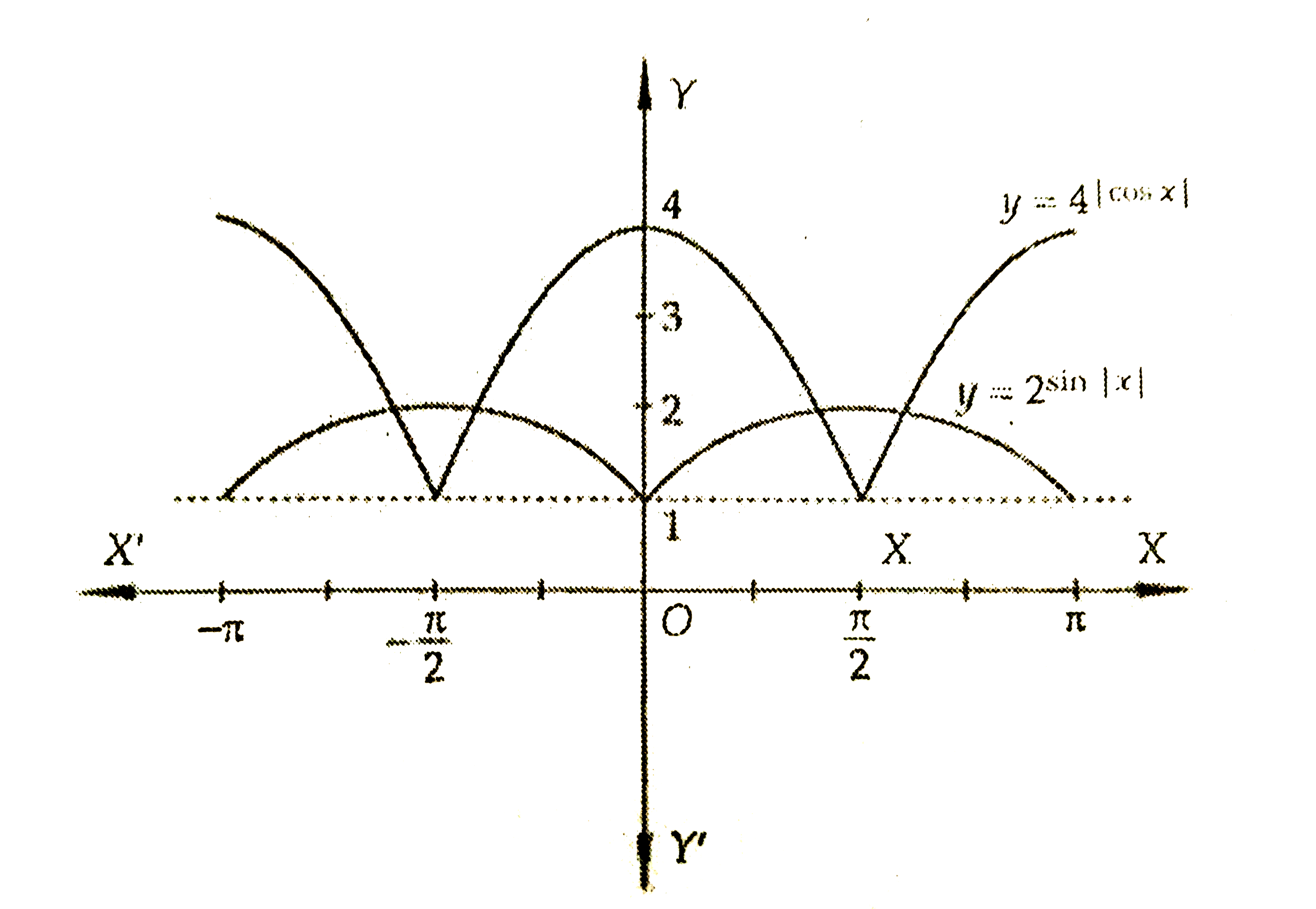
The total number of solutions of the given equation is equal to the number of points of intersection of curves `y=4^(|cosx|)` and `y=2^(sin|x|)`. Clearly, these two curves intersect a four points. So, there are four solutions of the given equation in the interval `[-pi,pi]`.