The number of solutions of the equation
e|sinx|=secx for all x∈(−π/2,π/2) is/are
1
3
5
2
The correct Answer is:B
Clearly, curves y=e|sinx| and y=sec x intersect at three points for all x∈(−π/2,π/2).
Hence, the given equation has three solutions in (−π/2,π/2).
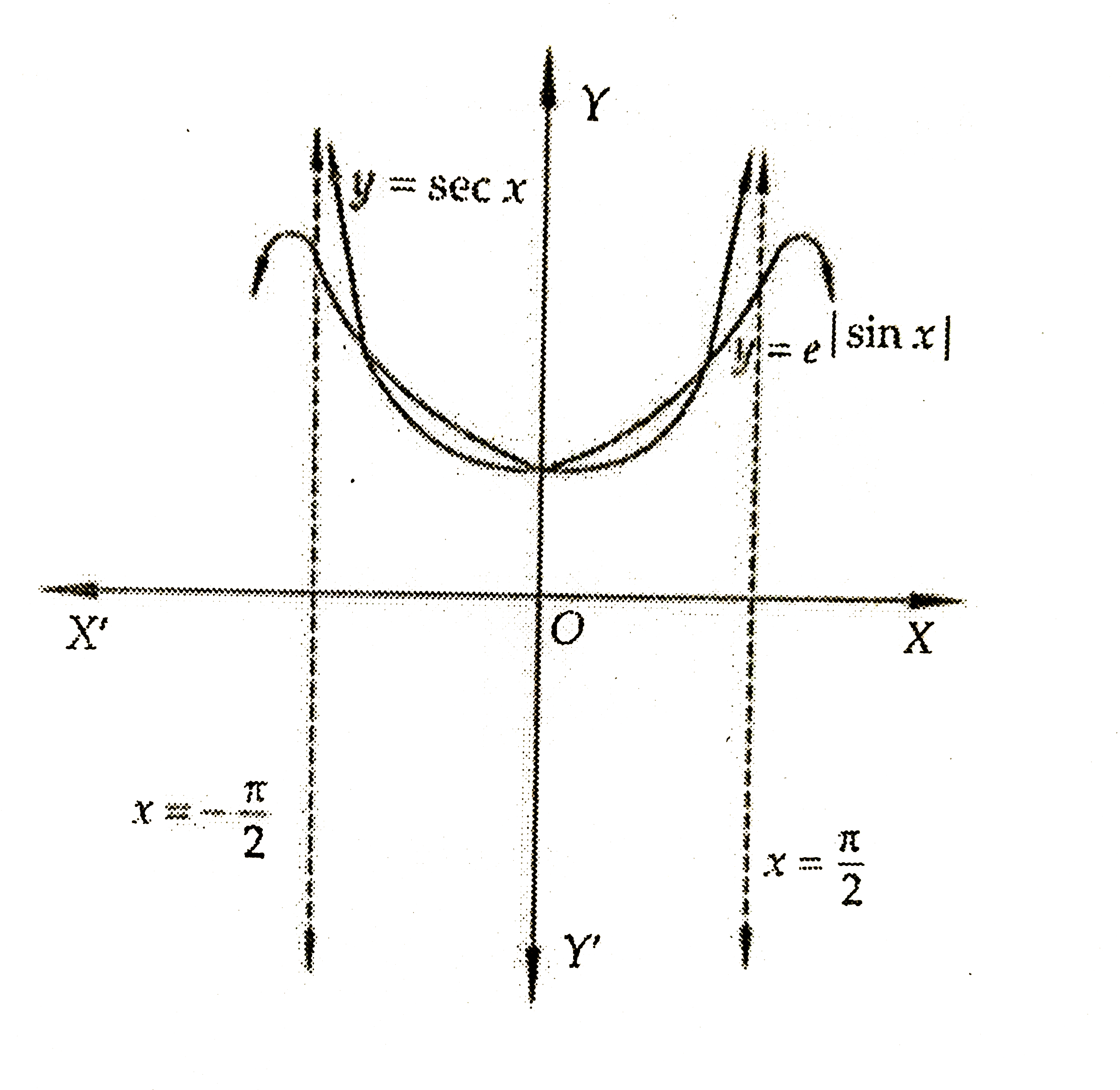
Topper's Solved these Questions
- MISCELLANEOUS EQUATIONS AND INEQUATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Exercise
30 Videos
- MISCELLANEOUS EQUATIONS AND INEQUATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Chapter Test
29 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
- PLANE AND STRAIGHT LINE IN SPACE BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PLANE AND STRAIGHT LINE IN SPACE EXERCISE - Chapter Test
31 Videos
Similar Questions
The total number of solutions of the equation sinxtan4x=cosx for all x∈(0,π) are
View SolutionThe number of solutions of equations |tan2x|=sinx in[0,π]
View SolutionNumber of solutions of the equation e−sin2x=tan2x in [0,10π] is
View SolutionThe number of solution of the equation |sinx|=|cos3x| in [−2π,2π] is
View SolutionThe number of solutions of the equation 1+sin4x=cos23x,x∈[−5π2,5π2] is
View SolutionThe total number of solution of the equation max(sinx,cosx)=12 for x∈(−2π,5π) is equal to (A) 3 (B) 6 (C) 7 (D) 8
View SolutionThe number of solutions of the equation sinx.Sin2x.Sin3x=1 in [0,2π] is
View SolutionThe number of solutions of the equation |y|=cosxandy=cot−1(cotx) in (−3π2,5π2) is
View SolutionThe number of solutions of the equation sinx. Sin2x. Sin3x = 1 in [0, 2pi]
View SolutionThe number of solutions of the equation x3+x2+4x+2sinx=0 in 0≤x≤2π is
View Solution
