Let Pn(x)=1+2x+3x2+............+(n+1)xn be a polynomial such that n is even.The number of real roots of Pn(x)=0 is
0
n
1
n+1
The correct Answer is:C
To solve the problem, we need to analyze the polynomial Pn(x)=1+2x+3x2+…+(n+1)xn where n is even, and determine the number of real roots of the equation Pn(x)=0.
1. Understanding the Polynomial:
The polynomial Pn(x) is given by:
Pn(x)=1+2x+3x2+…+(n+1)xn
Here, n is even, which means n=2k for some integer k.
2. Evaluating at Specific Points:
Let's evaluate Pn(x) at x=0:
Pn(0)=1+2(0)+3(0)2+…+(n+1)(0)n=1
This shows that Pn(0)=1, which is positive.
3. Behavior as x→∞:
As x approaches infinity, the term (n+1)xn dominates the polynomial since it has the highest degree. Therefore:
Pn(x)→∞asx→∞
4. Behavior as x→−∞:
As x approaches negative infinity, since n is even, the leading term (n+1)xn will also approach infinity:
Pn(x)→∞asx→−∞
5. Finding Critical Points:
To find the critical points, we can compute the derivative P′n(x):
P′n(x)=2+3⋅2x+4⋅3x2+…+(n+1)⋅nxn−1
Since all coefficients are positive, P′n(x)>0 for all x. This indicates that Pn(x) is a strictly increasing function.
6. Conclusion on Roots:
Since Pn(x) is strictly increasing, and we have established that Pn(0)=1>0 and Pn(x)→∞ as x→∞ and x→−∞, it follows that Pn(x) does not cross the x-axis. Therefore, the polynomial Pn(x)=0 has no real roots.
Final Answer:
The number of real roots of Pn(x)=0 is 0.
The polynomial Pn(x) is given by:
Pn(x)=1+2x+3x2+…+(n+1)xn
Here, n is even, which means n=2k for some integer k.
Let's evaluate Pn(x) at x=0:
Pn(0)=1+2(0)+3(0)2+…+(n+1)(0)n=1
This shows that Pn(0)=1, which is positive.
As x approaches infinity, the term (n+1)xn dominates the polynomial since it has the highest degree. Therefore:
Pn(x)→∞asx→∞
As x approaches negative infinity, since n is even, the leading term (n+1)xn will also approach infinity:
Pn(x)→∞asx→−∞
To find the critical points, we can compute the derivative P′n(x):
P′n(x)=2+3⋅2x+4⋅3x2+…+(n+1)⋅nxn−1
Since all coefficients are positive, P′n(x)>0 for all x. This indicates that Pn(x) is a strictly increasing function.
Since Pn(x) is strictly increasing, and we have established that Pn(0)=1>0 and Pn(x)→∞ as x→∞ and x→−∞, it follows that Pn(x) does not cross the x-axis. Therefore, the polynomial Pn(x)=0 has no real roots.
The number of real roots of Pn(x)=0 is 0.
Topper's Solved these Questions
- MISCELLANEOUS EQUATIONS AND INEQUATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MISCELLANEOUS EQUATIONS AND INEQUATIONS EXERCISE - Exercise
30 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
- PLANE AND STRAIGHT LINE IN SPACE BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - PLANE AND STRAIGHT LINE IN SPACE EXERCISE - Chapter Test
31 Videos
Similar Questions
Let Pn(ix)=1+2x+3x2+............+(n+1)xn be a polynomial such that n is even.The number of real roots of Pn(x)=0 is
View SolutionLet Pn(ix)=1+2x+3x2+............+(n+1)xn be a polynomial such that n is even.The number of real roots of Pn(x)=0 is
View SolutionThe maximum number of real roots of the equation x2n−1=0, is
View SolutionLet p(x) = a0+a1x+a2x2+.............+anxn be a non zero polynomial with integer coefficient . if p(√2+√3+√6)=0 , the smallest possible value of n . is
View SolutionIn the given figure graph of :
y=p(x)=xn+a1xn−1+a2xn−2+…..+an is given.
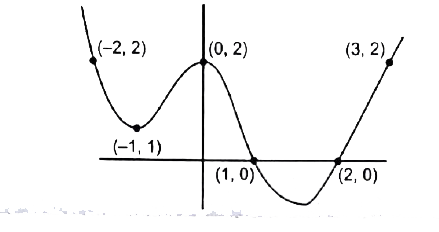
The minimum number of real roots of equation (p'(x))2+p(x)p''(x)=0 are:
View SolutionIn the given figure graph of :
y=p(x)=xn+a1xn−1+a2xn−2+…..+an is given.
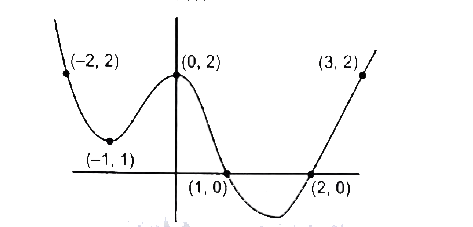
The product of all imaginary roots of p(x)=0 is:
View SolutionLet n be a positive integer with f(n)=1!+2!+3!+.........+n!andp(x),Q(x) be polynomial in x such that f(n+2)=P(n)f(n+1)+Q(n)f(n) for all n≥, Then
View SolutionLet f(x)=(x+1)(x+2)(x+3)…..(x+100)andg(x)=f(x)f''(x)−f'(x)2. Let n be the numbers of real roots of g(x)=0, then:
View SolutionLet Pn(u) be a polynomial is u of degree n. Then, for every positive integern, sin2nx is expressible is
View SolutionIf the polynomial equation anxn+an−1xn−1+an−2xn−2+.......+a0=0,n being a positive integer, has two different real roots a and b. then between a and b the equation nanxn−1+(n−1)an−1xn−2+.......+a1=0 has
View Solution
