The image of [-1,3] under f is not the interval
Statement-2: f is not an injective map.
1
2
3
4
The correct Answer is:A
To solve the problem, we need to analyze the function f(x)=12x2−12 and determine the image of the interval (−1,3) under this function. We will also check if the statement that the image is not the interval (f(−1),f(3)) implies that f is not an injective map.
Step 1: Calculate f(−1) and f(3)
First, we will evaluate the function at the endpoints of the interval:
f(−1)=12(−1)2−12=12(1)−12=0
f(3)=12(3)2−12=12(9)−12=108−12=96
Step 2: Determine the image of the interval (−1,3)
Next, we need to find the behavior of the function on the interval (−1,3). To do this, we will differentiate the function to find critical points.
Step 3: Differentiate f(x)
The derivative of f(x) is:
f′(x)=ddx(12x2−12)=24x
Step 4: Find critical points
Set the derivative equal to zero to find critical points:
24x=0⟹x=0
Step 5: Analyze the function's behavior
Now we will evaluate f(x) at the critical point x=0:
f(0)=12(0)2−12=−12
Now we have the values of f at the critical points and endpoints:
- f(−1)=0
- f(0)=−12
- f(3)=96
Step 6: Determine the image of (−1,3)
The function decreases from f(−1)=0 to f(0)=−12 and then increases from f(0)=−12 to f(3)=96. Therefore, the image of the interval (−1,3) is:
(−12,96)
Step 7: Compare with (f(−1),f(3))
The interval (f(−1),f(3)) is:
(0,96)
Since the image of (−1,3) is (−12,96), which is not equal to (0,96), we conclude that the image of (−1,3) under f is indeed not the interval (f(−1),f(3)).
Step 8: Determine if f is injective
Since the function decreases and then increases, it takes the same value at two different points (for example, f(−1)=0 and f(1)=0). Thus, f is not an injective map.
Conclusion
The statements are correct: the image of (−1,3) under f is not the interval (f(−1),f(3)), and f is not an injective map.
---
- f(0)=−12
- f(3)=96
Topper's Solved these Questions
- FUNCTIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - FUNCTIONSEXERCISE - Exercise
48 Videos
- FUNCTIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - FUNCTIONSEXERCISE - Chapter Test
29 Videos
- FUNCTIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - FUNCTIONSEXERCISE - Section I - Solved Mcqs
49 Videos
- DISCRETE PROBABILITY DISTRIBUTIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DISCRETE PROBABILITY DISTRIBUTIONSEXERCISE - Exercise
40 Videos
- HYPERBOLABOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - HYPERBOLAEXERCISE - Chapter Test
29 Videos
Similar Questions
A function f :R→R is defined by f(x)=2x3+3 . Show that f is a bijective mapping .
View SolutionIf f:R→R is defined by f(x)=x2+1, then show that f is not an injection.
View SolutionStatement -1: f(x)=x3−3x+1=0 has one root in the interval [-2,2].
Statement-2: f(-2) and f(2) are of opposite sign.
View SolutionSuppose that the quadratic function f(x)=ax2+bx+c is non-negative on the interval [-1,1] . Then, the area under tha graph of f over the interval [-1,1] and the X - axis is given by the formula
View SolutionLeg f be continuous on the interval [0,1] to R such that f(0)=f(1). Prove that there exists a point c in [0,12] such that f(c)=f(c+12).
View SolutionFind the image of interval [−1,3] under the mapping specified by the function f(x)=4x3−12x.
View SolutionFind the image of interval [−1,3] under the mapping specified by the function f(x)=4x3−12x.
View SolutionLet f,g:[−1,2]→ be continuous functions which are twice differentiable on the interval (-1,2). Let the values of f and g at the points and 2 be as given in the following table:
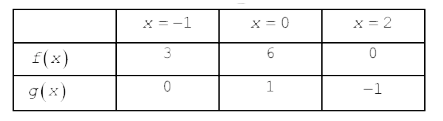
In each of the intervals (-1,0) and (0, 2) the function (f-3g) never vanishes. Then the correct statements(s) is(are) :
View Solutiony=f(x) is a polynomial function passing through point (0, 1) and which increases in the intervals (1,2)and(3,∞) and decreases in the intervals (−∞,1)and(2,3).
If f(1)=−8, then the range of f(x) is (a) [3,∞) (b) [−8,∞) (c) [−7,∞) (d) (−∞,6]
View SolutionConsider, f (x) is a function such that f(1)=1,f(2)=4andf(3)=9
Statement 1 : f''(x)=2 for some x∈(1,3)
Statement 2 : g(x)=x2⇒g''(x)=2,∀x∈R
View Solution
