If A1 is the area of the parabola y2=4ax lying between vertex and the latusrectum and A2 is the area between the latusrectum and the double ordinate x=2a, then A1A2 is equal to
2√2−1
(2√2+1)/7
(2√2−1)/7
none of these
The correct Answer is:B
To solve the problem, we need to find the areas A1 and A2 as defined in the question, and then compute the ratio A1A2.
Step 1: Understand the Parabola
The equation of the parabola is given as y2=4ax. The vertex of the parabola is at the origin (0,0), and the latus rectum is a vertical line that passes through the focus of the parabola, which is at the point (a,0).
Step 2: Determine the Area A1
The area A1 is the area between the vertex (0,0) and the latus rectum (which is at x=a). To find this area, we will integrate the function representing the parabola from x=0 to x=a.
The equation can be rewritten for y:
y=2√ax
Now, we calculate the area A1:
A1=∫a02√axdx
Step 3: Solve the Integral for A1
To solve the integral:
A1=2√a∫a0√xdx
The integral ∫√xdx is:
∫√xdx=23x3/2
Thus,
A1=2√a(23x3/2)a0=2√a⋅23a3/2=43a2
Step 4: Determine the Area A2
The area A2 is the area between the latus rectum (at x=a) and the double ordinate x=2a. We will integrate the same function from x=a to x=2a.
A2=∫2aa2√axdx
Step 5: Solve the Integral for A2
Again, we can factor out 2√a:
A2=2√a∫2aa√xdx
Calculating the integral:
A2=2√a(23x3/2)2aa=2√a(23(2a)3/2−23a3/2)
Calculating (2a)3/2=23/2a3/2=2√2a3/2:
A2=2√a⋅23(2√2a3/2−a3/2)=4√a3((2√2−1)a3/2)
A2=43a2(2√2−1)
Step 6: Calculate the Ratio A1A2
Now we have:
A1=43a2
A2=43a2(2√2−1)
Thus, the ratio is:
A1A2=43a243a2(2√2−1)=12√2−1
Step 7: Rationalize the Denominator
To rationalize 12√2−1:
12√2−1⋅2√2+12√2+1=2√2+1(2√2)2−12=2√2+18−1=2√2+17
Final Answer
Thus, the final result is:
A1A2=2√2+17
The equation of the parabola is given as y2=4ax. The vertex of the parabola is at the origin (0,0), and the latus rectum is a vertical line that passes through the focus of the parabola, which is at the point (a,0).
The area A1 is the area between the vertex (0,0) and the latus rectum (which is at x=a). To find this area, we will integrate the function representing the parabola from x=0 to x=a.
y=2√ax
A1=∫a02√axdx
To solve the integral:
A1=2√a∫a0√xdx
∫√xdx=23x3/2
A1=2√a(23x3/2)a0=2√a⋅23a3/2=43a2
The area A2 is the area between the latus rectum (at x=a) and the double ordinate x=2a. We will integrate the same function from x=a to x=2a.
Again, we can factor out 2√a:
A2=2√a∫2aa√xdx
A2=2√a(23x3/2)2aa=2√a(23(2a)3/2−23a3/2)
A2=2√a⋅23(2√2a3/2−a3/2)=4√a3((2√2−1)a3/2)
A2=43a2(2√2−1)
Now we have:
A1=43a2
A2=43a2(2√2−1)
A1A2=43a243a2(2√2−1)=12√2−1
To rationalize 12√2−1:
12√2−1⋅2√2+12√2+1=2√2+1(2√2)2−12=2√2+18−1=2√2+17
Thus, the final result is:
A1A2=2√2+17
Topper's Solved these Questions
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test
60 Videos
- AREAS OF BOUNDED REGIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - AREAS OF BOUNDED REGIONSEXERCISE - Chapter Test
60 Videos
- ALGEBRAIC INEQUATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - ALGEBRAIC INEQUATIONSEXERCISE - Exercise
39 Videos
- CARTESIAN PRODUCT OF SETS AND RELATIONS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CARTESIAN PRODUCT OF SETS AND RELATIONS EXERCISE - Chapter Test
30 Videos
Similar Questions
Knowledge Check
Let A1,A2…..,A3 be n arithmetic means between a and b. Then the common difference of the AP is
A(a) b−aB(b) a−bC(c) a−bn+1D(d) b−an+1A body A starts from rest with an acceleration a1. After 2 seconds, another body B starts from rest with an acceleration a2. If they travel equal distances in the 5th second, after the start of A, then the ratio a1:a2 is equal to :
A0.21458333333333B0.21319444444444C0.37847222222222D0.37986111111111
Area of the triangle formed by the vertex, focus and one end of latusrectum of the parabola (x+2)2=−12(y−1) is
View SolutionLet V be the vertex and L be the latusrectum of the parabola x2=2y+4x−4. Then the equation of the parabola whose vertex is at V. Latusrectum L/2 and axis s perpendicular to the axis of the given parabola.
View SolutionIf the area of the circle is A1 and the area of the regular pentagon inscribed in the circle is A2, then find the ratio A1A2.
View SolutionIf the area of the circle is A1 and the area of the regular pentagon inscribed in the circle is A2, then find the ratio A1A2.
View SolutionIf A1 and A2 are two A.M.s between a and b and G1 and G2 are two G.M.s between the same numbers then what is the value of A1+A2G1G2
View SolutionIn the circuit of figure A1 and A2 are ideal ammeters. Then the reading in ammeter A2 is
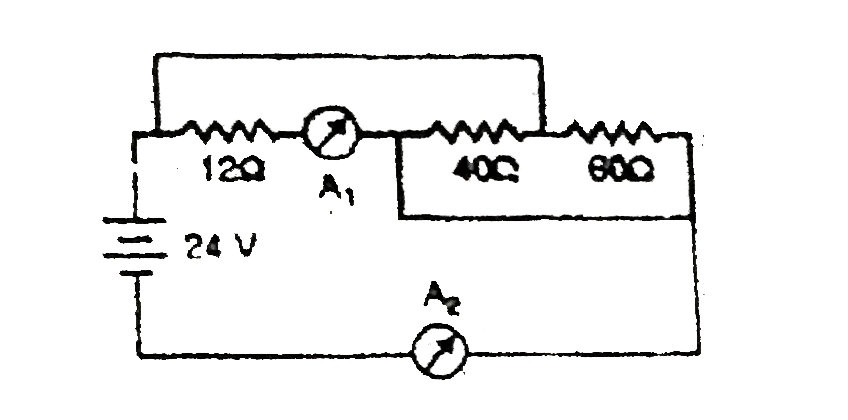
View Solution
