If the mean of the following frequency distribution is 5, then b =
xi :3574fi :2a5b
10
6
8
none of these
The correct Answer is:B
To find the value of b in the given frequency distribution, we will follow these steps:
Step 1: Set up the frequency distribution
We are given the following frequency distribution:
xifi325a754b
Step 2: Calculate xifi
We will calculate xifi for each xi and fi:
- For x1=3 and f1=2:
x1f1=3×2=6
- For x2=5 and f2=a:
x2f2=5×a=5a
- For x3=7 and f3=5:
x3f3=7×5=35
- For x4=4 and f4=b:
x4f4=4×b=4b
Now, we can summarize the xifi values:
xifixifi3265a5a75354b4b
Step 3: Calculate the total of xifi and fi
Now we will find the total of xifi and fi:
Total of xifi=6+5a+35+4b=41+5a+4b
Total of fi=2+a+5+b=7+a+b
Step 4: Set up the mean equation
We know that the mean ¯x is given as 5. The formula for mean is:
¯x=∑xifi∑fi
Substituting the totals we found:
5=41+5a+4b7+a+b
Step 5: Cross-multiply to eliminate the fraction
Cross-multiplying gives us:
5(7+a+b)=41+5a+4b
Expanding both sides:
35+5a+5b=41+5a+4b
Step 6: Simplify the equation
Now, we can simplify the equation:
Subtract 5a from both sides:
35+5b=41+4b
Subtract 4b from both sides:
35+b=41
Step 7: Solve for b
Now, isolate b:
b=41−35
b=6
Conclusion
Thus, the value of b is 6.
---
We are given the following frequency distribution:
We will calculate xifi for each xi and fi:
x1f1=3×2=6
- For x2=5 and f2=a:
x2f2=5×a=5a
- For x3=7 and f3=5:
x3f3=7×5=35
- For x4=4 and f4=b:
x4f4=4×b=4b
Now we will find the total of xifi and fi:
We know that the mean ¯x is given as 5. The formula for mean is:
Cross-multiplying gives us:
Now, we can simplify the equation:
Now, isolate b:
Thus, the value of b is 6.
Similar Questions
If the arithmetic mean of the following distribution is 8.2, then a =
xi :13591113fi :327a48
View SolutionIf the mean of the following distribution is 13, then p =
xi:51012171620fi:93P875
View SolutionThe median for the following frequency distribution is :
xi :123456789fi :810111620251596
View SolutionIf the mean of the following frequency distribution is 8, find the value of p.
Variable(xi)35791113Frequency(fi)6815p84
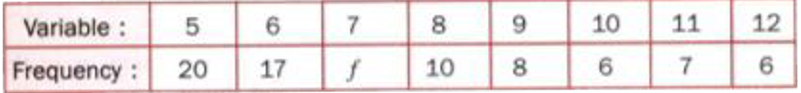
View SolutionIf the mean of the following distribution is 7.5, find the missing frequency f
View SolutionIf the mean of the following data is 5.5, then x =
xi2468fi356x
View SolutionFind the value of if the mean of the following distribution is 7.5
View SolutionIf the arithmetic mean of the following data is 7, then a + b =
xi4679fia4b5
View SolutionIf the mean of the following distribution is 6, find the value of p
x:24610p+5f:32312
View SolutionFind the mean of the following distribution: x: 4 6 9 10 15 f: 5 10 10 7 8
View Solution
