If the mean of the following frequency distribution is 8, find the value of p.
Variable(xi)35791113Frequency(fi)6815p84
The correct Answer is:p=25
To find the value of p in the given frequency distribution, we will follow these steps:
Step 1: Write down the given data
We have the following values for the variable x and their corresponding frequencies f:
| xi | 3 | 5 | 7 | 9 | 11 | 13 |
|-----------|----|----|----|----|----|----|
| fi | 6 | 8 | 15 | p | 8 | 4 |
Step 2: Calculate the total frequency Σf
The total frequency is the sum of all frequencies:
Σf=6+8+15+p+8+4=41+p
Step 3: Calculate Σfx
Next, we calculate Σfx by multiplying each xi by its corresponding fi:
Σfx=(3×6)+(5×8)+(7×15)+(9×p)+(11×8)+(13×4)
Calculating each term:
- 3×6=18
- 5×8=40
- 7×15=105
- 9×p=9p
- 11×8=88
- 13×4=52
Now, summing these values:
Σfx=18+40+105+9p+88+52=303+9p
Step 4: Set up the equation for the mean
The mean is given by the formula:
Mean=ΣfxΣf
Given that the mean is 8, we can set up the equation:
8=303+9p41+p
Step 5: Cross-multiply to eliminate the fraction
Cross-multiplying gives us:
8(41+p)=303+9p
Expanding both sides:
328+8p=303+9p
Step 6: Rearranging the equation
Now, we will rearrange the equation to isolate p:
328−303=9p−8p
This simplifies to:
25=p
Final Answer
Thus, the value of p is 25.
---
We have the following values for the variable x and their corresponding frequencies f:
|-----------|----|----|----|----|----|----|
| fi | 6 | 8 | 15 | p | 8 | 4 |
The total frequency is the sum of all frequencies:
Next, we calculate Σfx by multiplying each xi by its corresponding fi:
- 5×8=40
- 7×15=105
- 9×p=9p
- 11×8=88
- 13×4=52
The mean is given by the formula:
Cross-multiplying gives us:
Now, we will rearrange the equation to isolate p:
Thus, the value of p is 25.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - DATA HANDLING EXERCISE - EXERCISE 24A18videos
DATA HANDLING
- BOOK - ICSECHAPTER - DATA HANDLING EXERCISE - EXERCISE 24B10videos
DATA HANDLING
- BOOK - ICSECHAPTER - DATA HANDLING AND MEASURES OF CENTRAL TENDENCYEXERCISE - TRY THIS19videos
DATA HANDLING AND MEASURES OF CENTRAL TENDENCY
- BOOK - ICSECHAPTER - DECIMALS EXERCISE - TRY THIS41videos
DECIMALS
Similar Questions
If the mean of the following frequency distribution is 15, find the value of p.
Variable(xi)1012141618Frequency(fi)13p153228View SolutionThe mean of the following frequency distribution is 2117. Find the value of f
 View Solution
View SolutionIf the mean of the following distribution is 7.5, find the missing frequency f
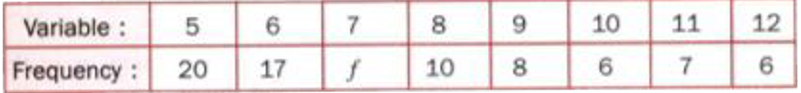 View Solution
View SolutionIf the mean of the following distribution is 6, find the value of p. x: 2 4 6 10 p+5 f: 3 2 3 1 2
View SolutionIf the mean of the following frequency distribution is 5, then b =
xi :3574fi :2a5bView SolutionIf the mean of the following distribution is 6, find the value of p
x:24610p+5f:32312View SolutionFind the median for the following frequency distribution :
Marks obtained(xi)293234274237Number of students(fi)25101284View SolutionFind the mean of the following distribution: 4 6 9 10 15 5 10 10 7 8
View SolutionFind the mean of the following frequency distribution: Variable (xi) , 2, 4, 6, 8, 10, 12, 14, 16 Frequency (fi) , 4, 4, 5, 15, 8, 5, 4, 5
View SolutionIf the arithmetic mean of the following distribution is 8.2, then a =
xi :13591113fi :327a48View Solution

