Question

A circle of radius 6 units touches the coordinates axes in the first
quadrant. Find the equation of its image in the line mirror $y=0.$

Options

A. `x^(2)+y^(2)-12x+12y+36=0`
B. `x^(2)+y^(2)-12x-12y+36=0`
C. `x^(2)+y^(2)+12x-12y+36=0`
D. `x^(2)+y^(2)+12x+12y+36=0`

Answer

A

Explanation

The given circle has radius 6 and the coordinates or its centre C are (6, 6). The coordinates of its image `C_(1)` in the line mirror y=0 i.e. x-axis are (6, -6).
So, the centre of the required circle is at `C_(1)(6, -6)` and its radius is 6.

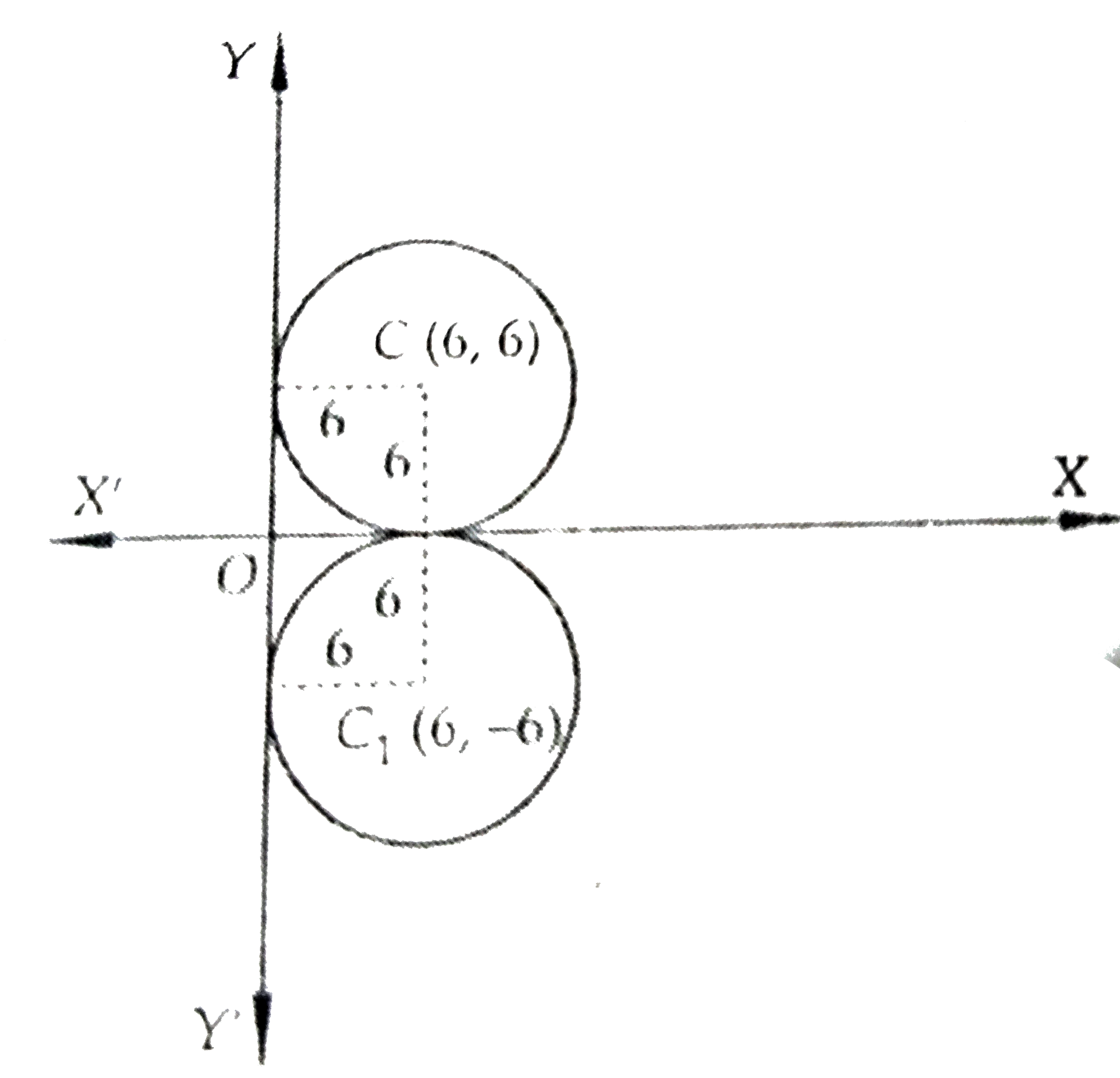
Hence, its equation is `(x-6)^(2)`