If f(x) is a cubic polynomial which has local maximum
at x=−1,Iff(2)=18,f(1)=−1andf'(x) has local minimum at x=0, then
the distance between (-1,2) and (α,f(α) where x=α is the point of local minima is 2√5
f(x) is increasing for x in [1,2sqrt5] and has a local minima at x=1
the value of f(0) is 5
none of these
The correct Answer is:B
It given that f'(x) has a local minimum at x=0
∴x=0 is a zero of f''(x)
⇒f''(x)=ax [∴f(x) is a cubic polynomial]
⇒f'(x)=ax22+bandf(x)=ax36+bx+c
It also given that f(x) has a local maximum at x=-1
∴f'(−1)=0⇒a2+b=0⇒a=−2b
We have ,
f(1) and f(2)=18
⇒−1=16+b+cand18=4a3+2b+c
Solving these eqations we get a−572,b=574,c=172
∴f(x)=5712x3−574x+172=14(19x3−57x+34) It is given that x=a is the point of local minima
∴a=1andf(α)=−1
Distance between (-1,2) and (-1,1) is (√13)
So option (a) is not correct
Since f(x) is a continous function localmaxima at x=-1 and local minima at x=1 such that f(-1) =18 and f(1) =-1 so a rough sketch of f(x) is as shown below
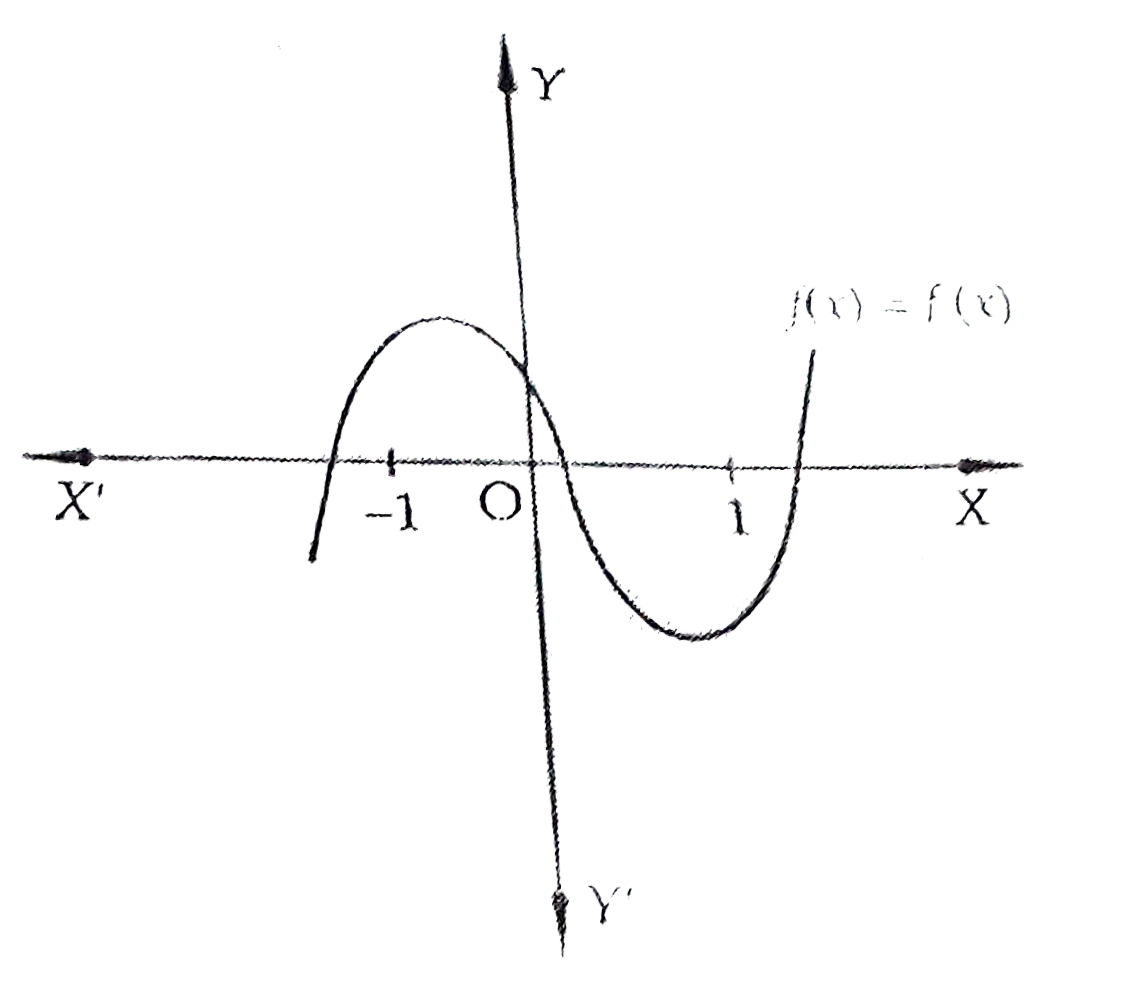
Clearly ,f(x) is increasing in [1,2√5] and f(x)has a local minima at x=1 .So option (b) is correct.
Topper's Solved these Questions
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Section II - Assertion Reason Type
7 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Exercise
47 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Chapter Test
29 Videos
- MATHEMATICAL REASONING BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MATHEMATICAL REASONING EXERCISE - Chapter Test
20 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
Similar Questions
If f(x) is a cubic polynomil which as local maximum at x=-1 . If f(2)=18,f(1)=-1 and f'(x) has minimum at x=0 then
View SolutionLet f(x) be a cubic polynomial which has local maximum at x=−1 and f(x) has a local minimum at x=1.iff(−1)=10 and f(3)=−22, then one fourth of the distance between its two horizontal tangents is ____________
View Solution
Knowledge Check
The function f(x)=x2+2x has a local minimum at
Ax = 1Bx = 2Cx = - 2Dx = - 1
Let g(x) be a cubic polnomial having local maximum at x=−1 and g '(x) has a local minimum at x=1,Ifg(−1)=10g,(3)=−22, then
View Solutionlet f(x)=(x2−1)n(x2+x−1) then f(x) has local minimum at x=1 when
View Solutionf(x) is cubic polynomial with f(x)=18andf(1)=−1 . Also f(x) has local maxima at x=−1andf′(x) has local minima at x=0 , then (A) the distance between (−1,2)and(af(a)), where x=a is the point of local minima is 2√5 (B)f(x) is increasing for x∈[1,2√5) (C)f(x) has local minima at x=1 (D)the value of f(0)=15
View SolutionLet p(x) be a real polynomial of least degree which has a local maximum at x=1 and a local minimum at x=3. If p(1)=6andp(3)=2, then p′(0) is_____
View SolutionLet p(x) be a real polynomial of least degree which has a local maximum at x=1 and a local minimum at x=3. If p(1)=6andp(3)=2, then p′(0) is_____
View SolutionLet f(x) be a polynomial of degree 3 such that f(−2)=5,f(2)=−3,f'(x) has a critical point at x=−2 and f''(x) has a critical point at x = 2. Then f(x) has a local maxima at x = a and local minimum at x = b. Then find b-a.
View SolutionThe function f(x)= x2+ 2x has a local minimum at
View Solution
