Let f(x)=∫x0(sint−cost)(et−2)(t−1)3(t−1)3(t−2)5dt,0<x≤4
Then , the number of points where f(x) assumes local maximum value , is
A
1
B
2
C
3
D
none of these
Video Solution
Text Solution
The correct Answer is:C
We have f(x)=x∫0(sint−cost)(et−2)(t−1)3(t−1)3(t−2)5dt,0<x≤4
⇒f'(x)=x∫0(sint−cost)(et−2)(x−1)3(x−2)5,0<x≤4
for local maximum or minimum , we must have
f'(x)=0
⇒sinx−cosx=0,ex−2=0,(x−1)3=0,(x−2)5=0
⇒tanx=1,ex=2,x=1,2
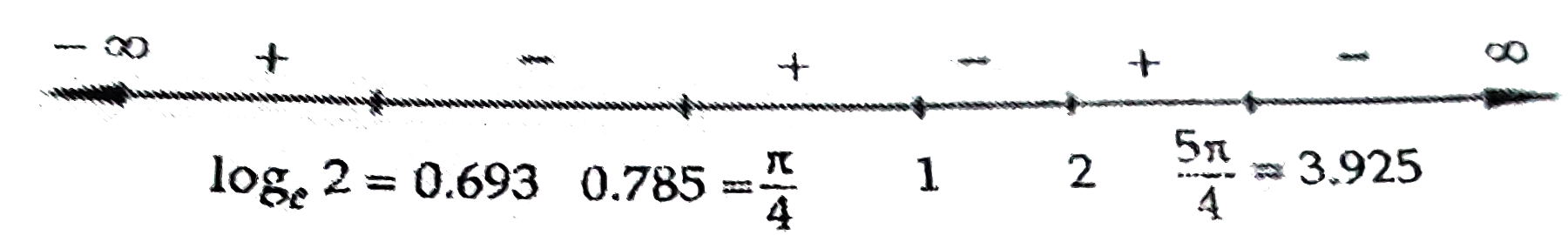
⇒x=54,5π4,x=loge2,x=1,2 [∴0<x≤4]
⇒x=0.785,3.925,0.693,1,2
The changes in sign s of f'(x) in the nieghbourhoods of these points are shown in Fig.17.
Clearly x=loge2,π4and5π4 are points of local maxima
|
Topper's Solved these Questions
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Section II - Assertion Reason Type
7 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Exercise
47 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Chapter Test
29 Videos
- MATHEMATICAL REASONING BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MATHEMATICAL REASONING EXERCISE - Chapter Test
20 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
Similar Questions
if f(x)=∫x−1t(et−1)(t−1)(t−2)3(t−3)5dthas a local minimum then sum of values of x
View SolutionLet f(x)=∫x2f(t2−3t+4)dt. Then
View Solutionf(x)=/∫x0(t−1)(t−2)2(t−4)5dt(x>0) then numb er of points of extremum of f(x) is
View SolutionLet f(x)=∫x0sin100tsin100t+cos100tdt, then f(2π)=
View Solutionf(x)=∫x0et3(t2−1)(t+1)2011dt(x>0) then :
View SolutionLet f(x)=∫x0cos(t2+2t+15)dt,o>x>2, then
View SolutionLet f(x)=∫x−2|t+1|dt, then
View SolutionLet f(x)=∫x0et(t−1)(t−2)dt. Then, f decreases in the interval
View SolutionLet f(x)=∫x0(t−1)(t−2)2dt, then find a point of minimum.
View SolutionThe function ∫x−1t(et−1)(t−1)(t−2)3(t−3)5dt has local minimum at x=
View Solution
