Let IR→IR be defined as f(x)=|x|++x2−1∣. The total number of points at which f attains either a local maximum or a local minimum is_______
2
4
5
6
The correct Answer is:C
We have
f(x)=|x|+∣∣x2−1∣∣=⎧⎪
⎪
⎪
⎪⎨⎪
⎪
⎪
⎪⎩−x+x2−1,x≤−1−x−x2+1,−1x<0x−x2+1,0≤x<1x+x2−1,x≥1
⇒f(x)=⎧⎪
⎪
⎪
⎪⎨⎪
⎪
⎪
⎪⎩2x−1,x<−1−2x−1,−1<x<0−2x+1,0<x<12x+1,x<1
Clearly f(x) is not differetaible at x = -1 ,0,1
The chages in sings of f(x) for different values of x are shown is Fig. 31
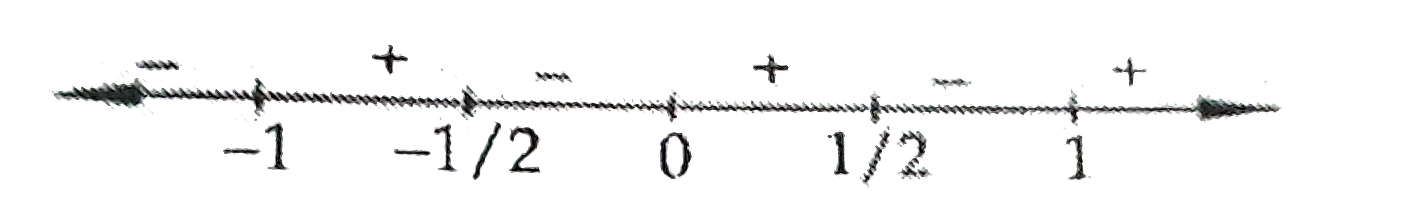
So f(x) changes its sign at 5 points
Hence , total number of points of local maximum of local minimum of f(x)is 5.
Topper's Solved these Questions
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Section II - Assertion Reason Type
7 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Exercise
47 Videos
- MAXIMA AND MINIMA BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MAXIMA AND MINIMA EXERCISE - Chapter Test
29 Videos
- MATHEMATICAL REASONING BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MATHEMATICAL REASONING EXERCISE - Chapter Test
20 Videos
- MEASURES OF CENTRAL TENDENCYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - MEASURES OF CENTRAL TENDENCYEXERCISE - Chapter Test
21 Videos
Similar Questions
Let f:R→R be defined as f(x)=|x|+∣∣x2−1∣∣. The total number of points at which f attains either a local maximum or a local minimum is_______
View SolutionLet f(x)=x3 find the point at which f(x) assumes local maximum and local minimum.
View SolutionLet f(x)=x3−3x2+6 find the point at which f(x) assumes local maximum and local minimum.
View SolutionLet f(x)=sinx−x on[0,π/2] find local maximum and local minimum.
View SolutionFind the local maximum and local minimumof f(x)=x3−3x in [-2,4].
View SolutionLet f(x)=x(x−1)2, find the point at which f(x) assumes maximum and minimum.
View SolutionLet f(x)=−sin3x+3sin2x+5 on [0,π2] . Find the local maximum and local minimum of f(x).
View SolutionLet f be a function defined on R (the set of all real numbers) such that f′(x)=2010(x−2009)(x−2010)2(x−2011)3(x−2012)4, for all x∈R. If g is a function defined on R with values in the interval (0,∞) such that f(x)=ln(g(x)), for all x∈R, then the number of point is R at which g has a local maximum is ___
View SolutionLet f be a function defined on R (the set of all real numbers) such that f′(x)=2010(x−2009)(x−2010)2(x−2011)3(x−2012)4, for all x∈R. If g is a function defined on R with values in the interval (0,∞) such that f(x)=ln(g(x)), for all x∈R, then the number of point is R at which g has a local maximum is ___
View SolutionLet f(x) be a function defined as follows: f(x)=sin(x2−3x),x≤0;and6x+5x2,x>0 Then at x=0,f(x) (a) has a local maximum (b) has a local minimum (c) is discontinuous (d) none of these
View Solution