If zand¯z represent adjacent vertices of a regular polygon of n sides where centre is origin and if Im(z)Re(z)=√2−1, then n is equal to:
8
16
24
32
The correct Answer is:A
Clearly, ¯z=zei2π/n. Let z=r,eiθ. Then, ¯z=re−θ.
⇒re−iθei2π/n⇒e−i2θ=ei2πn⇒θ=−πn
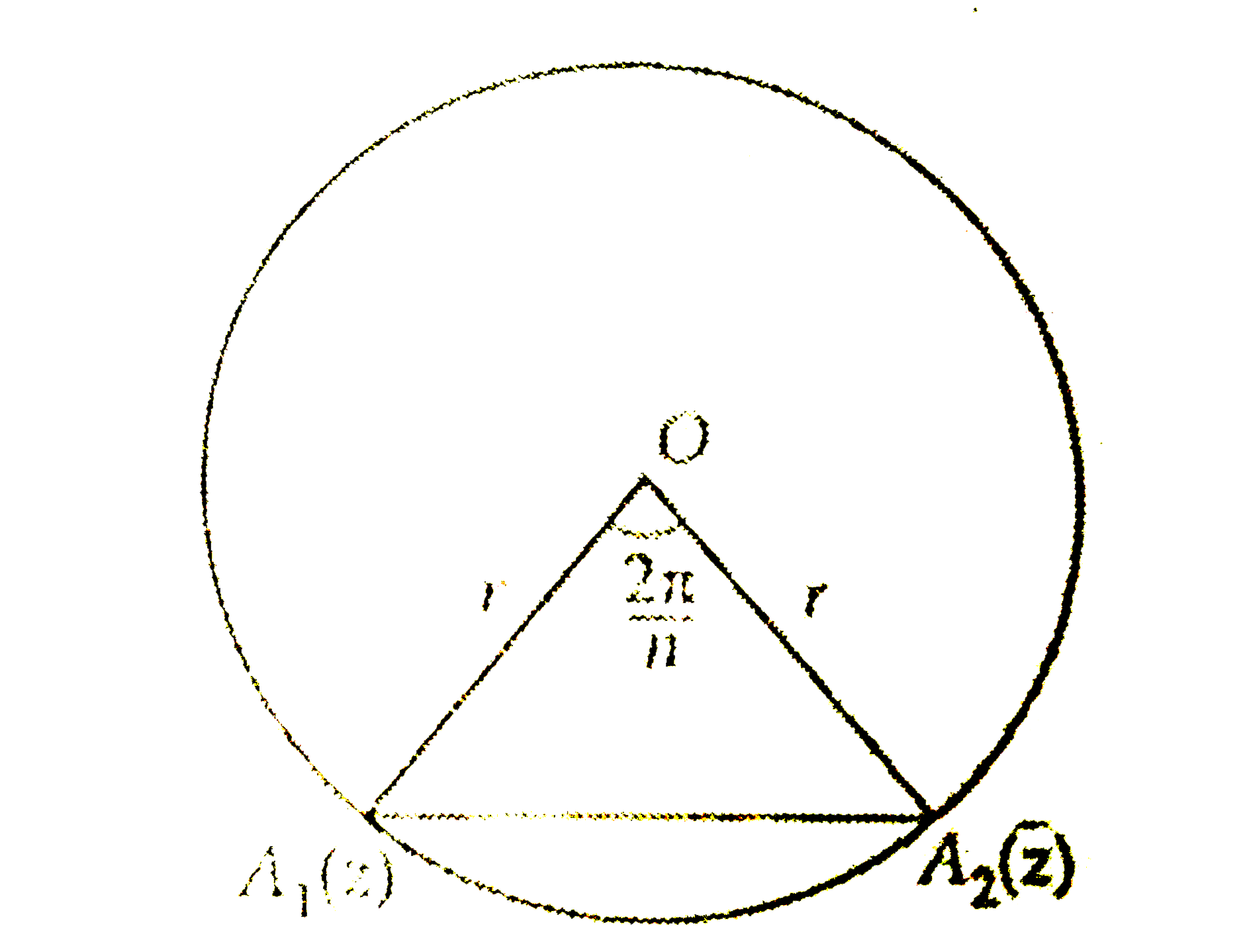
Now, ∣∣∣Im(z)Re(z)∣∣∣=√2−1
⇒∣∣∣rsinθrcosθ∣∣∣=√2−1
⇒tanπ2=√2−1⇒tanπn=tanπ8⇒n=8
Topper's Solved these Questions
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Section II - Assertion Reason Type
15 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Exercise
129 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test
58 Videos
- CIRCLESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CIRCLESEXERCISE - Chapter Test
53 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
87 Videos
Similar Questions
If i=√−1, then (in+i−n,n∈Z) is equal to
View SolutionIf i=√−1, then (in+i−n,n∈Z) is equal to
View SolutionIf z1=cosθ+isinθ and 1,z1,(z1)2,(z1)3,.....,(z1)n−1 are vertices of a regular polygon such that Im(z1)2ReZ1=√5−12, then the value n is
View SolutionIf z=−2+2√3i, then z2n+22n⋅zn+24n is equal to
View SolutionLet z be a complex number such that |z|+z=3+I
(Where i=√−1)
Then ,|z| is equal to
View SolutionThe center of a regular polygon of n sides is located at the point z=0, and one of its vertex z1 is known. If z2 be the vertex adjacent to z1, then z2 is equal to _____________.
View SolutionIf (3+i)(z+¯z)−(2+i)(z−¯z)+14i=0, where i=√−1, then z ¯z is equal to
View SolutionIf z4+1=√3i (A) z3 is purely real (B) z represents the vertices of a square of side 214 (C) z9 is purely imaginary (D) z represents the vertices of a square of side 234
View SolutionIf z1andz2 are complex numbers such that |z1−z2|=|z1+z2| and A and B re the points representing z1andz2 then the orthocentre of △OAB, where O is the origin is (A) z1+z22 (B) 0 (C) z1−z22 (D) none of these
View SolutionIf z1,z2,z3………….zn are in G.P with first term as unity such that z1+z2+z3+…+zn=0. Now if z1,z2,z3……..zn represents the vertices of n-polygon, then the distance between incentre and circumcentre of the polygon is
View Solution
