Let z∈C and if A={z,arg(z)=π4} and B={z,arg(z−3−3i)=2π3}. Then n(A∩B) is equal to
A
1
B
2
C
3
D
0
Video Solution
Text Solution
The correct Answer is:D
We observe that arg(z) =π/4 is the ray originating from origin making angle π/4 with OX. So, A is an infinite set of points lying on this ray. The set B is the set of all points on the ray originating from (3,3) making angle (2π/3) with x-axis as shown in fig. 70. The initial points of these two rays are not included in the two sets .
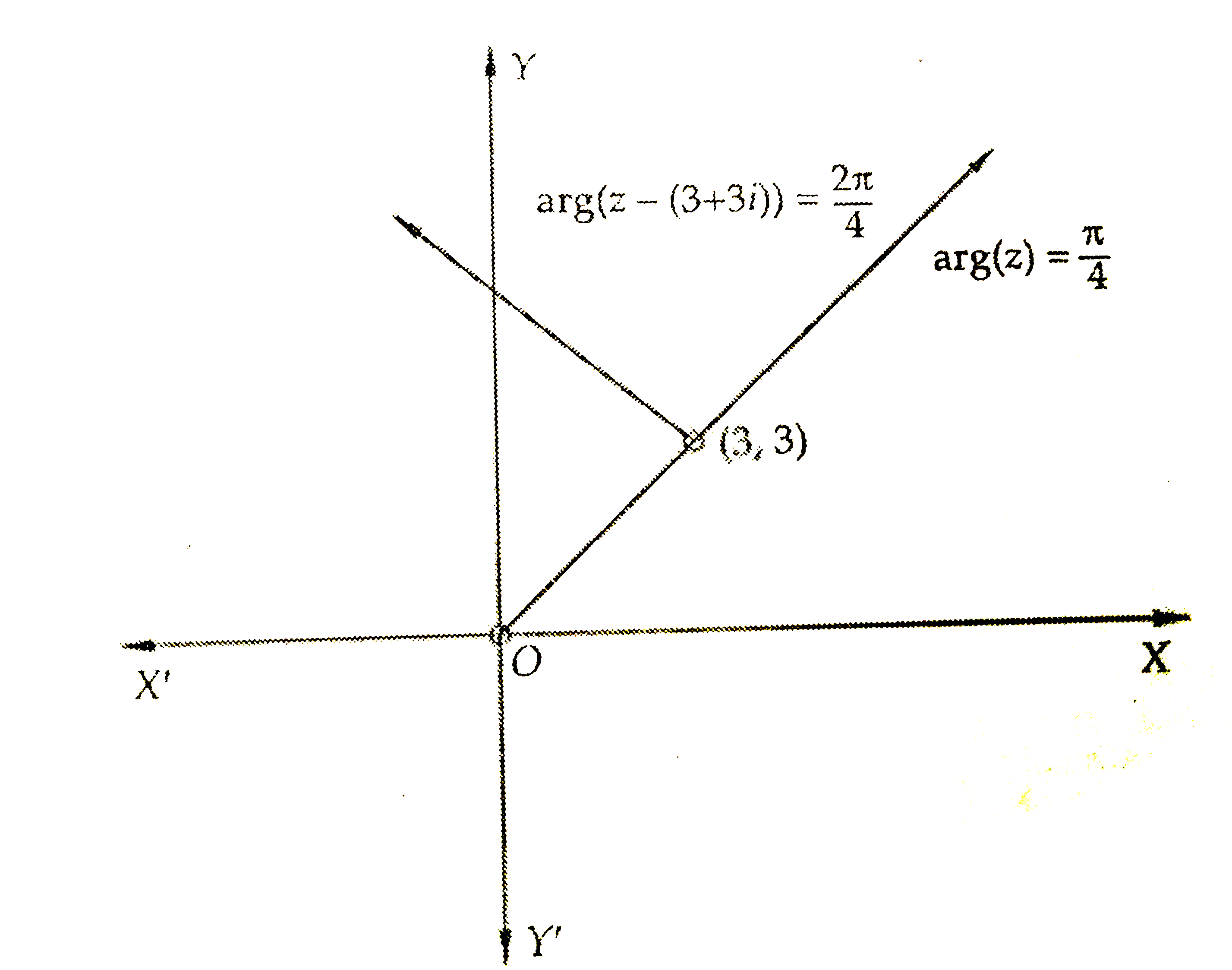
Hence, A⌢B=ϕ and so n(A⌢B)=0.
|
Topper's Solved these Questions
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Section II - Assertion Reason Type
15 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Exercise
129 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - COMPLEX NUMBERS EXERCISE - Chapter Test
58 Videos
- CIRCLESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CIRCLESEXERCISE - Chapter Test
53 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
87 Videos
Similar Questions
Let z∈C and if A={z:arg(z)=π4}and B={z:arg(z−3−3i)=2π3}. Then n(A⌢B)=
View Solutionif arg(z+a)=π6 and arg(z−a)=2π3 then
View SolutionIf |z|=2 and arg (z)=π4 , find z.
View SolutionIf argz=π4 ,then
View SolutionFor a complex number Z, if arg Z=π4 and ∣∣∣Z+1Z∣∣∣=4, then the value of ∣∣∣|Z|−1|Z|∣∣∣ is equal to
View SolutionLet Z0 is the root of equation x2+x+1=0 and Z=3+6i(Z0)81−3i(Z0)93 Then arg (Z) is equal to (a) π4 (b) π3 (c) π (d) π6
View SolutionThe area bounded by the curves argz=π3andargz=2π3andarg(z−2−2i√3)=π in the argand plane is (in sq. units)
View SolutionFind the value of z, if |z|=4andarg(z)=5π6.
View SolutionFind the area bounded by |argz|≤π/4 and |z−1|<|z−3|.
View SolutionIf arg(z−6−3iz−3−6i)=π4 , then maximum value of |z| :
View Solution
