If p , q , r are the legths of the internal bisectors of angles A ,B , C respectively of a !ABC , then area of ABC

1a+1b−1c
1a+1c−1b
1a+1b+1c
1b+1c−1a
We have,
Δ=Area of Δ ABD + Area of Δ ACD
⇒Δ=12pcsinA2+12pbsinA2
⇒12bcsinA=12pcsinA2+12pbsinA2
⇒12bc(2sinA2cosA2)=12pcsinA2+12pbsinA2
⇒2bosA2=pc+pb⇒2pcosA2=1b+1c
Similarly, we have
2qcosB2=1a+1c and 2rcosC2=1a+1b
∴2pcosA2+2qcosB2+2rcosC2=(1b+1c)+(1a+1c)+(1a+1b)
⇒1pcosA2+1qcosB2+1rcosC2=1a+1b+1c
Topper's Solved these Questions
- PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMBook:OBJECTIVE RD SHARMA ENGLISHChapter:PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMExercise:Section II - Assertion Reason Type
Explore 13 Videos
- PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMBook:OBJECTIVE RD SHARMA ENGLISHChapter:PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMExercise:Exercise
Explore 97 Videos
- PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMBook:OBJECTIVE RD SHARMA ENGLISHChapter:PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMExercise:Section I - Solved Mcqs
Explore 95 Videos
- PLANE AND STRAIGHT LINE IN SPACE Book:OBJECTIVE RD SHARMA ENGLISHChapter:PLANE AND STRAIGHT LINE IN SPACE Exercise:Chapter Test
Explore 31 Videos
- REAL FUNCTIONS Book:OBJECTIVE RD SHARMA ENGLISHChapter:REAL FUNCTIONS Exercise:Chapter Test
Explore 58 Videos
Similar Questions
Let AD, BE, CF be the lengths of internal bisectors of angles A, B, C respectively of triangle ABC. Then the harmonic mean of ADsecA2,BEsecB2,CFsecC2 is equal to :
View SolutionLet ABC be a triangle inscribed in a circle and let la=maMa,lb=mbMb,lc=mcMc where ma,mb,mc are the lengths of the angle bisectors of angles A, B and C respectively , internal to the triangle and Ma,MbandMc are the lengths of these internal angle bisectors extended until they meet the circumcircle.
Q. la equals :
View SolutionLet ABC be a triangle inscribed in a circle and let la=maMa,lb=mbMb,lc=mcMc where ma,mb,mc are the lengths of the angle bisectors of angles A, B and C respectively , internal to the triangle and Ma,MbandMc are the lengths of these internal angle bisectors extended until they meet the circumcircle.
Q. The maximum value of the product (lalblc)×cos2(B−C2)×cos2(C−A)2)×cos2(A−B2) is equal to :
View SolutionLet ABC be a triangle inscribed in a circle and let la=maMa,lb=mbMb,lc=mcMc where ma,mb,mc are the lengths of the angle bisectors of angles A, B and C respectively , internal to the triangle and Ma,MbandMc are the lengths of these internal angle bisectors extended until they meet the circumcircle.
Q. The minimum value of the expression lasin2A+lbsin2B+lcsin2C is :
View SolutionProve that the internal bisector of the angle A of a triangle ABC divides BC in the ratio AB:AC
View SolutionIn the triangle ABC, vertices A, B, C are (1,2),(3,1),(−1,6) respectively. If the internal angle bisector of ∠BAC meets BC at D, then the coordinates of D are (53,83)
View SolutionABC has integral side lengths. Internal angle bisector of angle B meets side AC at D. If AD = 3, DC = 8, then perimeter of ABC is- (1) 37 (2) 36 (3) 35 (4) 33
View SolutionIn △ABC, the coordinates of the vertex A are , (4,−1) and lines x−y−1=0and2x−y=3 are the internal bisectors of angles B and C . Then the radius of the circles of triangle AbC is
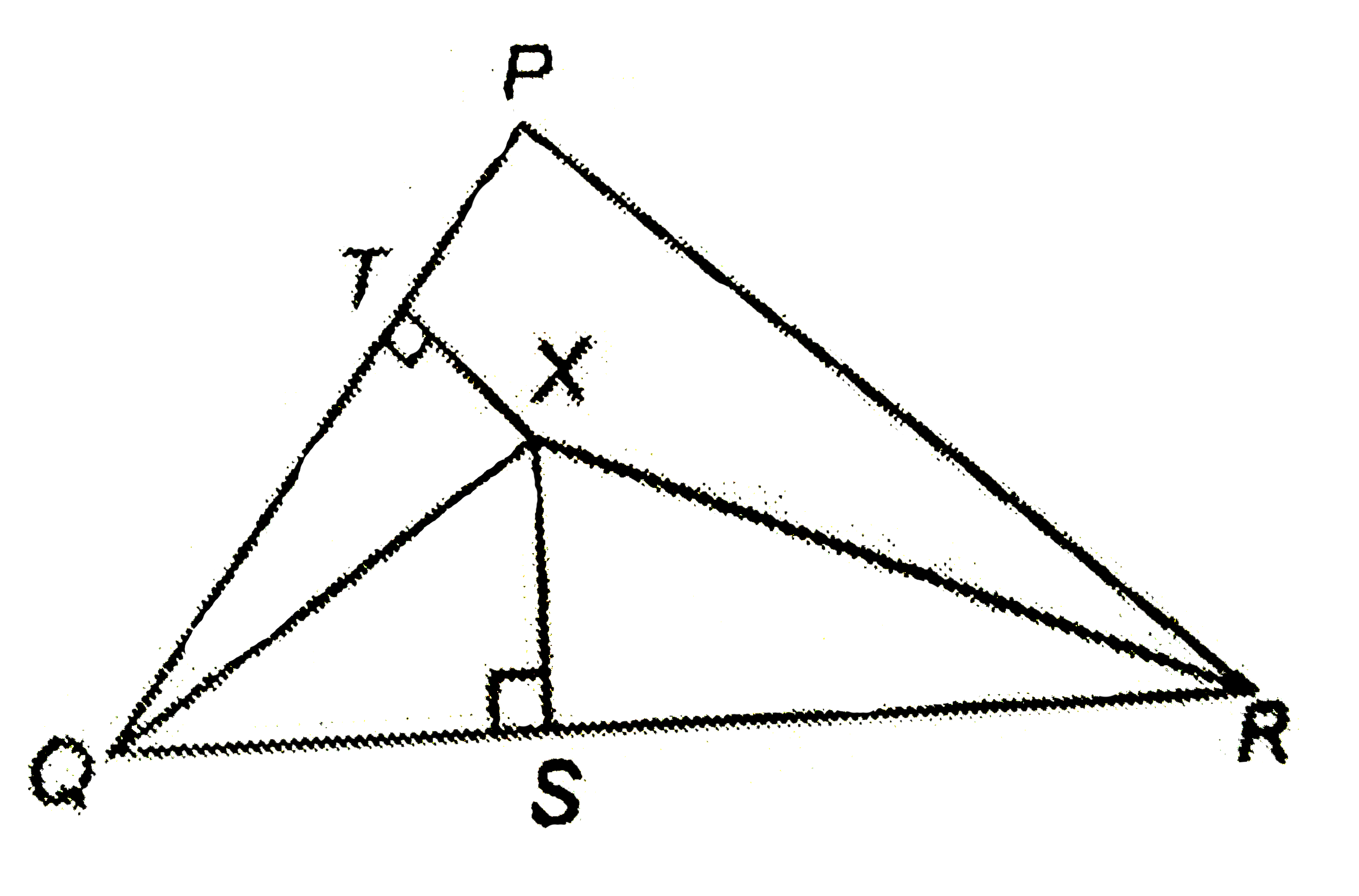
View SolutionIn the adjoining figure, QX and RX are the bisectors of the angles Q and R respectively of the angles Q and R respectively of the triangle PQR. If XS⊥PQ. Prove that :
(i) ΔXTQ≅ΔXSQ
(ii) PX bisects angle P.
View SolutionIn a triangle ABC , AB = AC and ∠A=36∘ If the internal bisector of angle C meets AB at D . Prove that AD = BC.
View Solution
