If $θ_{1}$ of $θ_{2}$ be the apparent angles of dip observed in two vertical planes at right angles to each other, then the true angle of dip $θ$ given by

A

${tan}^{2} θ = {tan}^{2} θ_{1} + {tan}^{2} θ_{2}$

B

${cot}^{2} θ = {cot}^{2} θ_{1} - {cot}^{2} θ_{2}$

C

${tan}^{2} θ = {tan}^{2} θ_{1} - {tan}^{2} θ_{2}$

D

$cot θ = {cot}^{2} θ_{1} + {cot}^{2} θ_{2}$

Text Solution

Verified by Experts

The correct Answer is:D

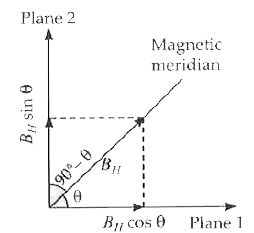
Let $B_{H}$ and $B_{v}$ be the horizontal and vetical components of earth.s magnetic field $\to B$ . Since $θ$ is the angle of dip
$∴ tan θ = \frac{B_{V}}{B_{H}} or cot θ = \frac{B_{H}}{B_{V}} ... . (i)$
Suppose planes 1 and 2 are two mutually perpendicualr planes and respectively make angle $θ$ and $90^{\circ} - θ$ withe the magnetic meridian. The vetical compunets of earth.s magnetic field remains same in the the two planes but the effective horizontal components in the planes will be
$B_{1} = B_{H} sin θ$
and $B_{2} = B_{H} sin θ$ The angles of dip $θ_{1}$ and $θ_{2}$ in the two planes are given by
${tan θ}_{1} = \frac{B_{V}}{B_{1}}$
${tan θ}_{1} = \frac{B_{V}}{B_{H} cos θ}$
or ${cot θ}_{1} = \frac{B_{H} cos θ}{B_{V}}$
Similary,
${cot θ}_{2} = \frac{B_{H} sin θ}{B_{V}}$
From eqns . (ii) and (iii)
${cot}^{2} θ_{1} + {cot}^{2} θ_{2} = \frac{B_{H}^{2}}{B_{V}^{2}} ({cos}^{2} θ + {sin}^{2} θ) = \frac{B_{H}^{2}}{B_{V}^{2}}$
$∴ {cot}^{2} θ_{1} + {cot}^{2} θ_{2} = {cot}^{2} θ$

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If $θ_{1}$ and $θ_{2}$ be the apparent angles of dip observed in two vertical planes at right angles to each other, then the true angle of dip $θ$ is given by
A ${cot}^{2} θ = {cot}^{2} θ_{1} + {cot}^{2} θ_{2}$
B ${tan}^{2} θ = {tan}^{2} θ_{1} + {tan}^{2} θ_{2}$
C ${cot}^{2} θ = {cot}^{2} θ_{1} - {cot}^{2} θ_{2}$
D ${tan}^{2} θ = {tan}^{2} θ_{1} - {tan}^{2} θ_{2}$
Question 2 - Select One
If $θ_{1}$ and $θ_{2}$ be the apparent angles of dip observed in two vertical planes at right angles to each other, then the true angle of dip $θ$ is given by
A ${cot}^{2} θ = {cot}^{2} θ_{1} + {cot}^{2} θ_{2}$
B ${tan}^{2} θ = {tan}^{2} θ_{1} + {tan}^{2} θ_{2}$
C ${cot}^{2} θ = {cot}^{2} θ_{1} + {cot}^{2} θ_{2}$
D ${tan}^{2} θ = {tan}^{2} θ_{1} - {tan}^{2} θ_{2}$
Question 3 - Select One
If $θ_{1}$ and $θ_{2}$ be the apparent angles of dip observed in two vertical planes at right angles to each other, then the true angle of dip $θ$ is given by
A ${tan}^{2} θ = {tan}^{2} θ_{1} + {tan}^{2} θ_{2}$
B ${cot}^{2} θ = {cot}^{2} θ_{1} - {cot}^{2} θ_{2}$
C ${tan}^{2} θ = {tan}^{2} θ_{1} - {tan}^{2} θ_{2}$
D ${cot}^{2} θ = {cot}^{2} θ_{1} + {cot}^{2} θ_{2}$

MTG GUIDE-MAGNETIC EFFECTS OF CURRENT AND MAGNETISM-AIPMT/NEET (MCQs)

Home
Ask Doubt
Profile