Question

For which values of a and b, the zeroes of $q\left(x\right)=x^3+2x^2+a$ are also the zeroes of the polynomial $p\left(x\right)=x^5-x^4-4x^3+3x^2+3x+b$? Which zeores of $p\left(x\right)$ are not the zeroes of $p\left(x\right)$?

Answer

N/a

Explanation

`:'` The zeroes of q(x) are also the zeroes fo p(x)
`:.` q(x) is a factor of p(x). `" "` [`:'` degree of q(x) is less than the degree of p(x)]
Polynomials
Now are division algorithm,

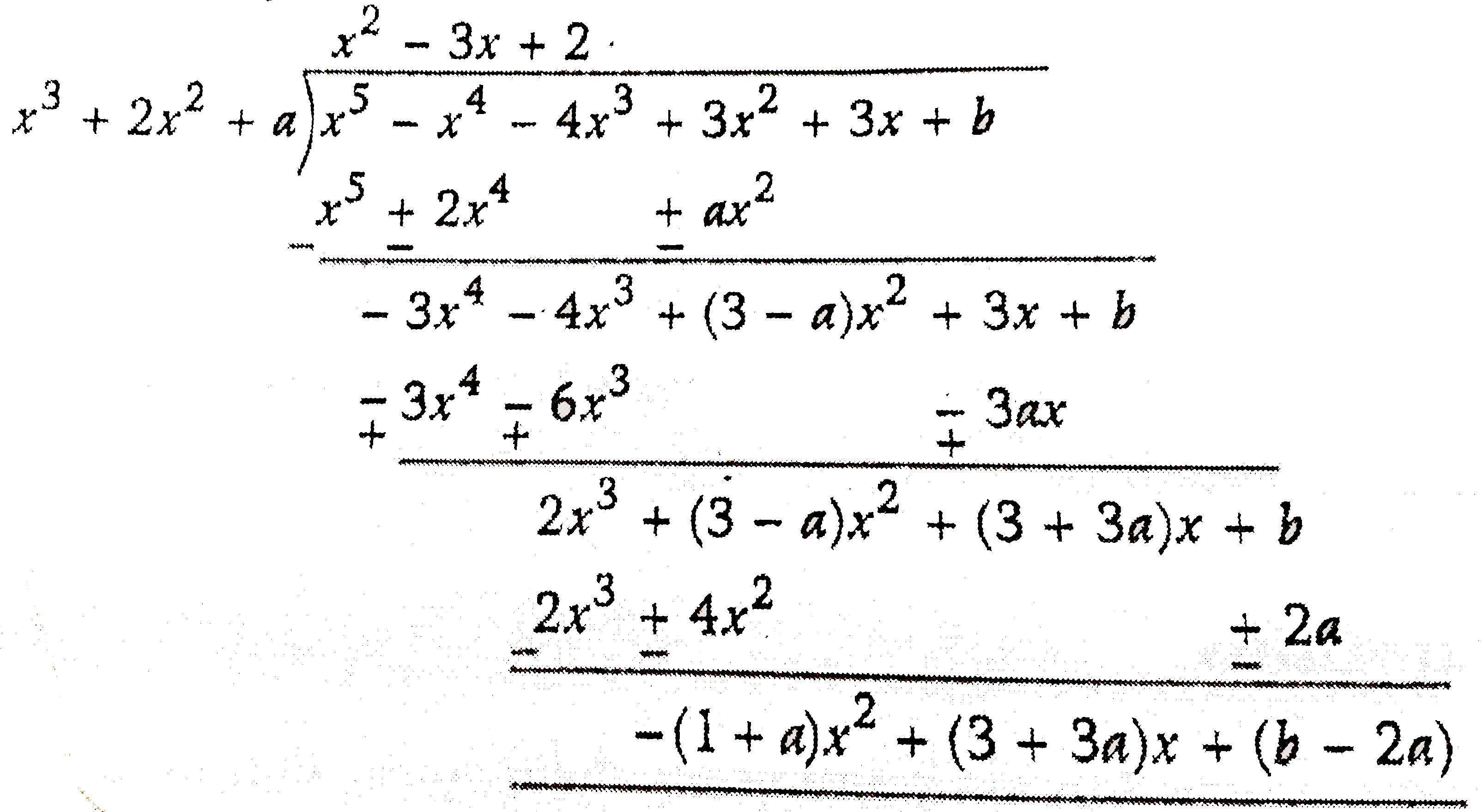
Here, since q(x) is a factor of p(x). So, the remainder should be zero. i.e..,
`-(1+a)x^(2)+(3+3a)x+(b-2a)=0`
`implies " " 1+a=0, " "3+3a=0, " " b-2a=0`
`implies " "a=-1, " " a=-1, " " b=2a`
`implies " " a=-1, " " b=-2,`
`:." "q(x)=x^(3)+2x^(2)-1`
and`" " p(x)=x^(5)-x^(4)-4x^(3)+3x^(2)+3x-2`
Now, `" " p(x)=(x^(3)+2x^(2)-1)(x^(2)-3x+2)+0`
`=(x^(3)+2x^(2)-1)(x-1)(x-2)`
Other zeroes of p(x) are 1 and 2.