In the adjoining figure, CM = 5 cm, RB = 9 cm, , O is the centre of larger circle and K is the centre of smaller circle. Find the area of shaded region
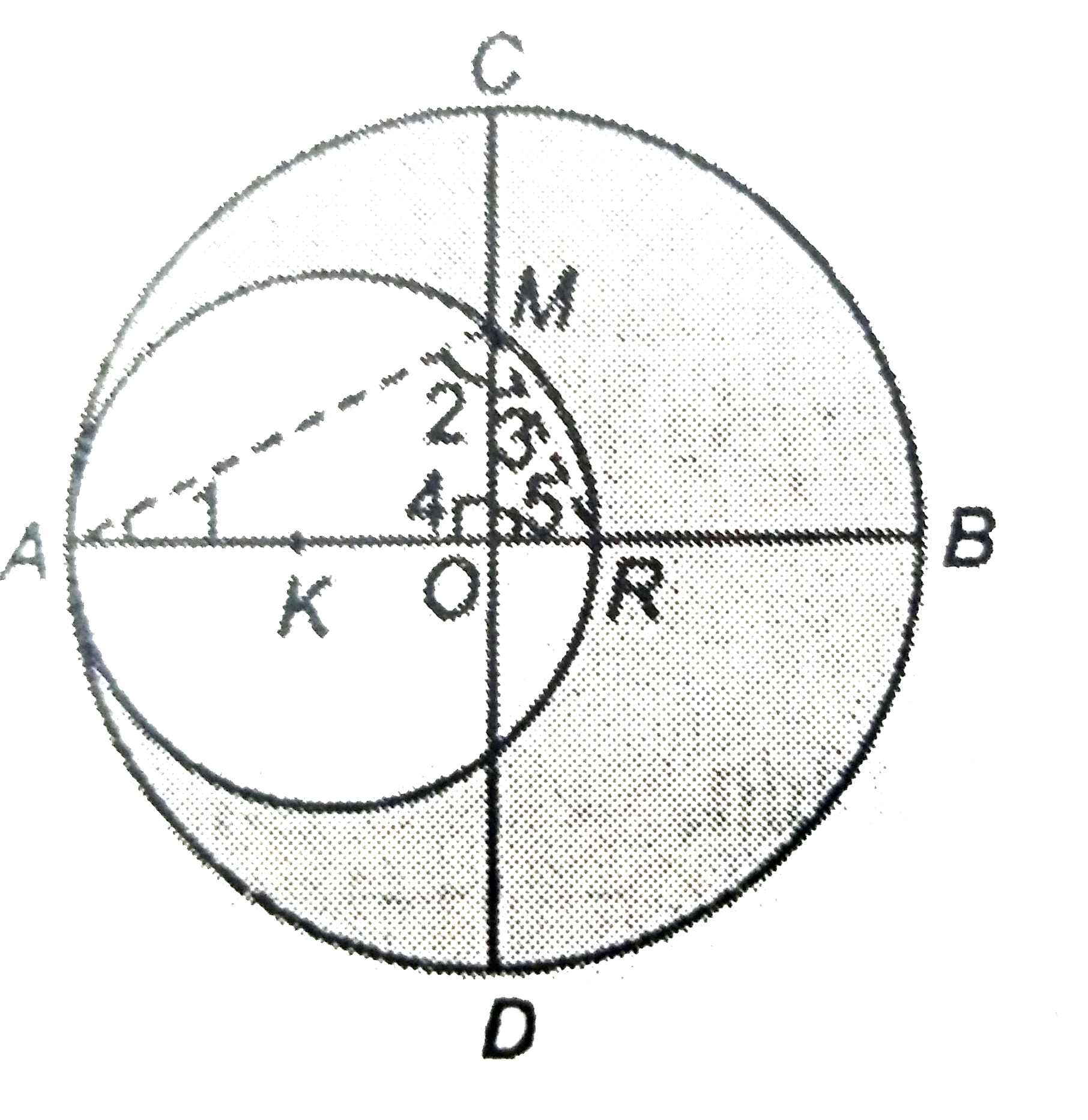
Let radius of larger circle be R and the radius of smaller circle is r.
Since,
Also
In ,
...(1)
In ,
(angle in a semicircle is right angle) ..(2)
From eqs. (1) and (2), we get
In
(just proved)
(each )
(AA corollary)
Now,
Required area
Topper's Solved these Questions
- AREA RELATED TO CIRCLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - AREA RELATED TO CIRCLESEXERCISE - Problems From NCERT /exemplar
25 Videos
- AREA RELATED TO CIRCLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - AREA RELATED TO CIRCLESEXERCISE - Exercise 12 A
24 Videos
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Revision Exercise Long Answer Question
5 Videos
Similar Questions
In the figure, AB is a diameter of the circle with centre O and OA = 7cm. Find the area of the shaded region
View SolutionIn the given figure, O is the centre of the circle with radius 14 cm. Find the area of the shared portion

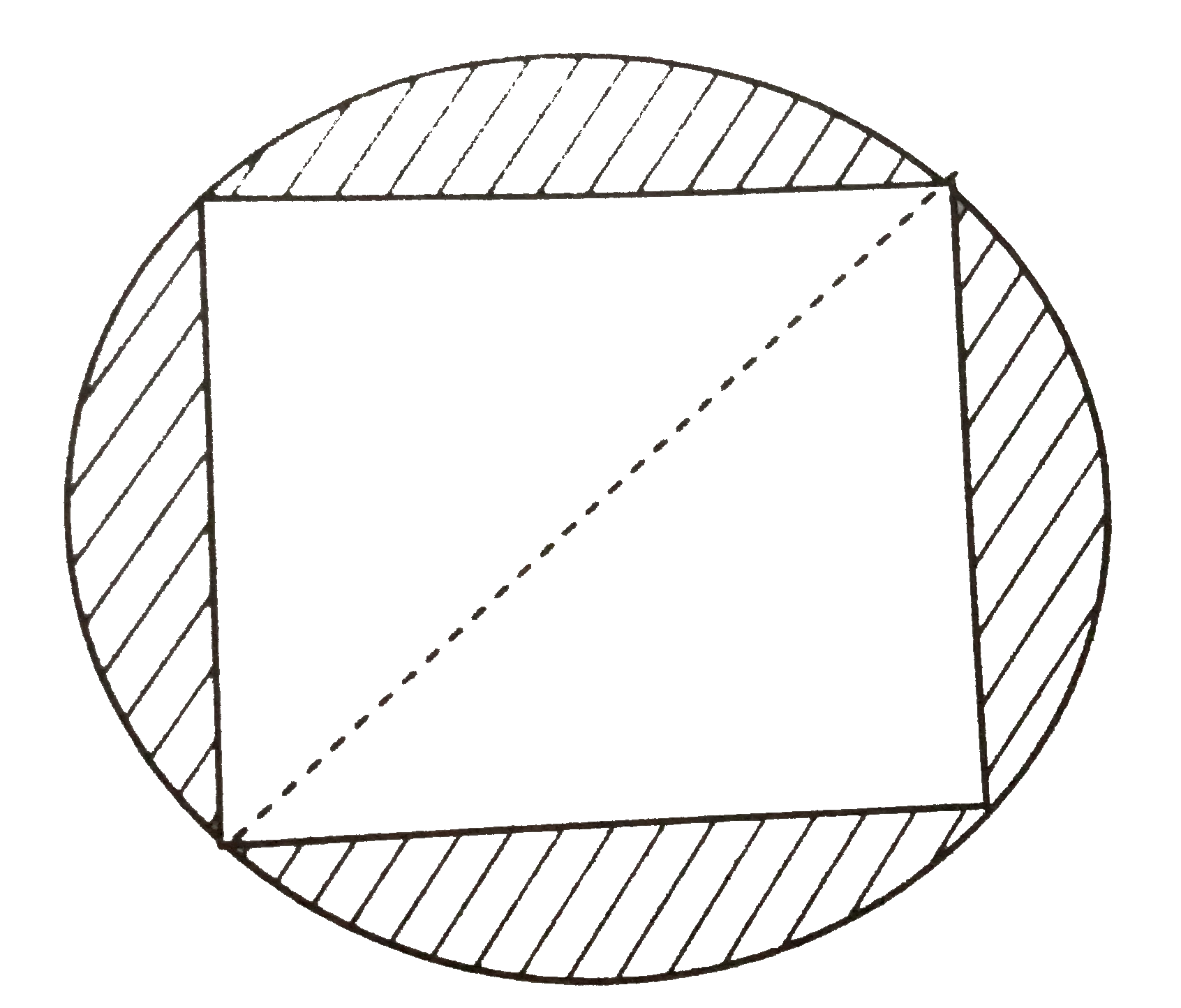
View SolutionIn figure, a square of diagonal 8 cm is inscribed in a circle. Find the area of the shaded region.
View SolutionIn the given figure, PQ = 24 cm PR = 7 cm and O is the centre of the circle. Find the area of the shaded region. (Use π=3.14)

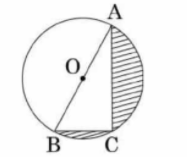
View SolutionIn fig. O is the center of a circle such that diameter AB=13cm and AC= 12 cm. BC is joined. Find the area of the shaded region.
View SolutionIn Figure, O is the centre of circle of radius 28 cm. Find the area of minor segment ASB .
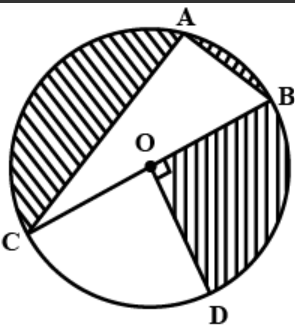
View SolutionIn Figure , O is the centre of the circle with AC = 24 cm, AB = 7 cm and ∠BOD=90°. Find the area of the shaded region.[Useπ=3⋅14]
View SolutionIn the adjoining figure O is the centre of circle and c is the mid point. the radius of circle is 17 cm . if OC=8cm, then find the length of chord AB.
View SolutionIn Figure 6, O is the centre of the circle with AC = 24 cm, AB = 7 cm and ∠BOD=90°. Find the area of the shaded region.[Useπ=3⋅14]

View SolutionIn the adjoining figure, O is the centre of the circle. If the chord AB is equal to the radius of the circle, then find the value of ∠ADB.

View Solution
