The area of an equilateral triangle ABC is $17320.5 c m^{2}$ . With each vertex of the triangle as centre, a circle is drawn with radius equal to half the length of the side of the triangle (see Fig. 12.28). Find the area of the shaded region. ( $U s e$ $π = 3.14 and$ $\sqrt{3}$ = 1.73205)

Video Solution

Text Solution

Verified by Experts

Let each side of equilateral triangle be x cm
Area of equilateral triangle $= \frac{\sqrt{3}}{4} x^{2}$
Given area of equilateral triangel $= 17320.5 c m^{2}$
$∴ 17320.5 = \frac{\sqrt{3}}{4} x^{2}$
or $x^{2} = \frac{17320.5 \times 4}{\sqrt{3}}$ ...(1)
Area of sector AMN $= \frac{π r^{2} θ}{360^{\circ}} = 3.14 \times {(\frac{x}{2})}^{2} \times \frac{60^{\circ}}{360^{\circ}}$

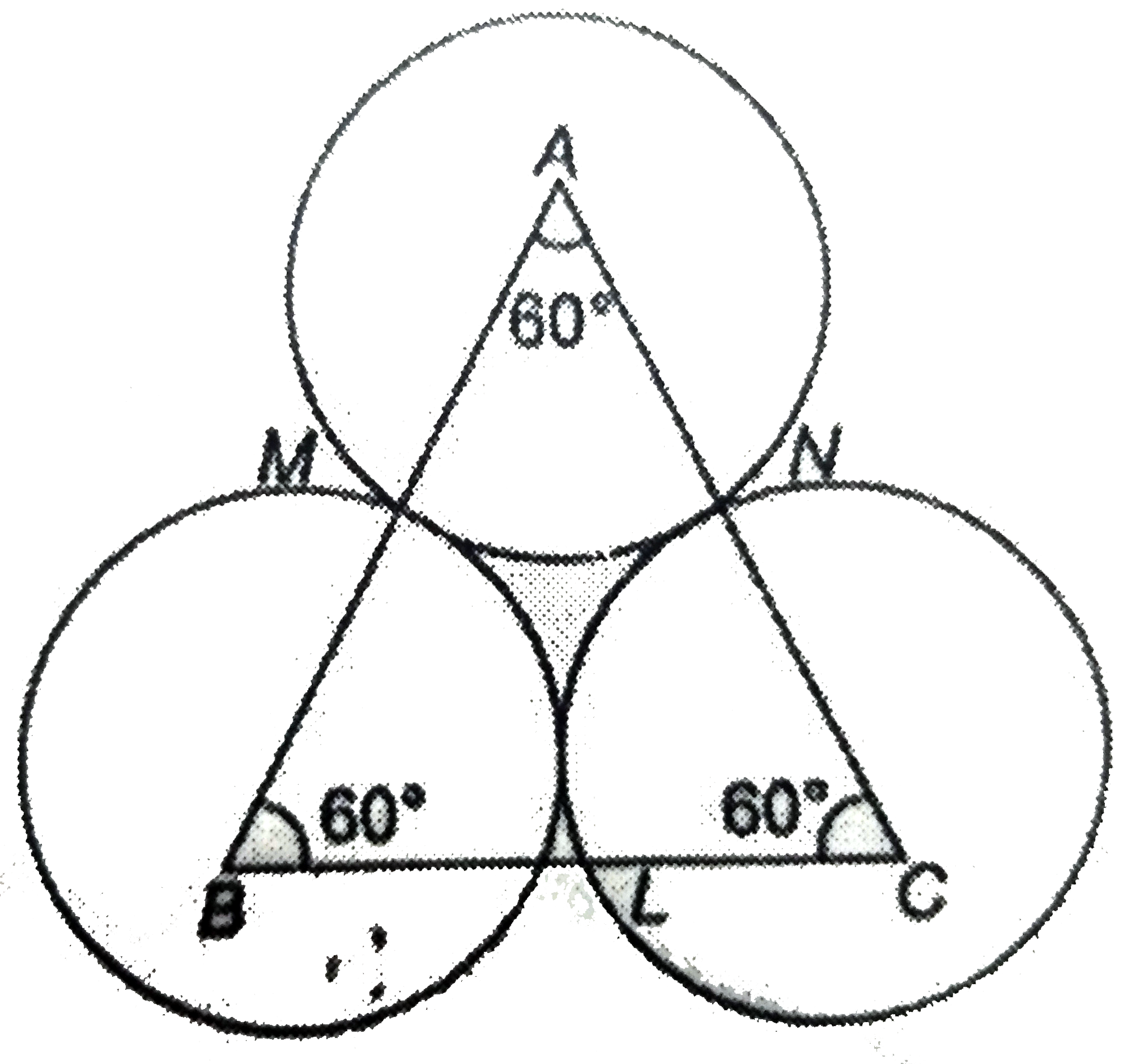
Area of 3 sectors $= 3.14 \times \frac{x^{2}}{4} \times \frac{60^{\circ}}{360^{\circ}} \times 3$
$= 3.14 \times \frac{1}{4} \times \frac{17320.5 \times 4}{\sqrt{3}} \times \frac{60^{\circ}}{360^{\circ}} \times 3$ [from (1)]
$= 3.14 \times \frac{17320.5}{2 \sqrt{3}}$
$= 3.14 \times \frac{17320.5}{2 \sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}}$ (Multiplying with $\frac{\sqrt{3}}{\sqrt{3}}$ )
$= \frac{1.57 \times 17320.5 \times \sqrt{3}}{3} = 15700 c m^{2}$
Hence, the area not included in the circle = Area of $Δ -$ Area of three sectors
$= 17320.5 - 15700 = 1620.5 c m^{2}$

|

Updated on:21/07/2023

Class 10 MATHS AREAS RELATED TO CIRCLES

Topper's Solved these Questions

Similar Questions

NAGEEN PRAKASHAN ENGLISH-AREA RELATED TO CIRCLES-Problems From NCERT /exemplar

Home
Ask Doubt
Profile