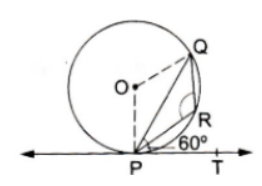
In the adjoining figure, PQ is a chord of a circle and PT is the tangent at P such that ∠QPT=60∘.Find ∠PRQ.
Join OP and OQ. Take any point S on the circumference in the alternate segment. Join SP and SQ.
Since, OP⊥PT
(radius through point of contact is bot to the tangent)

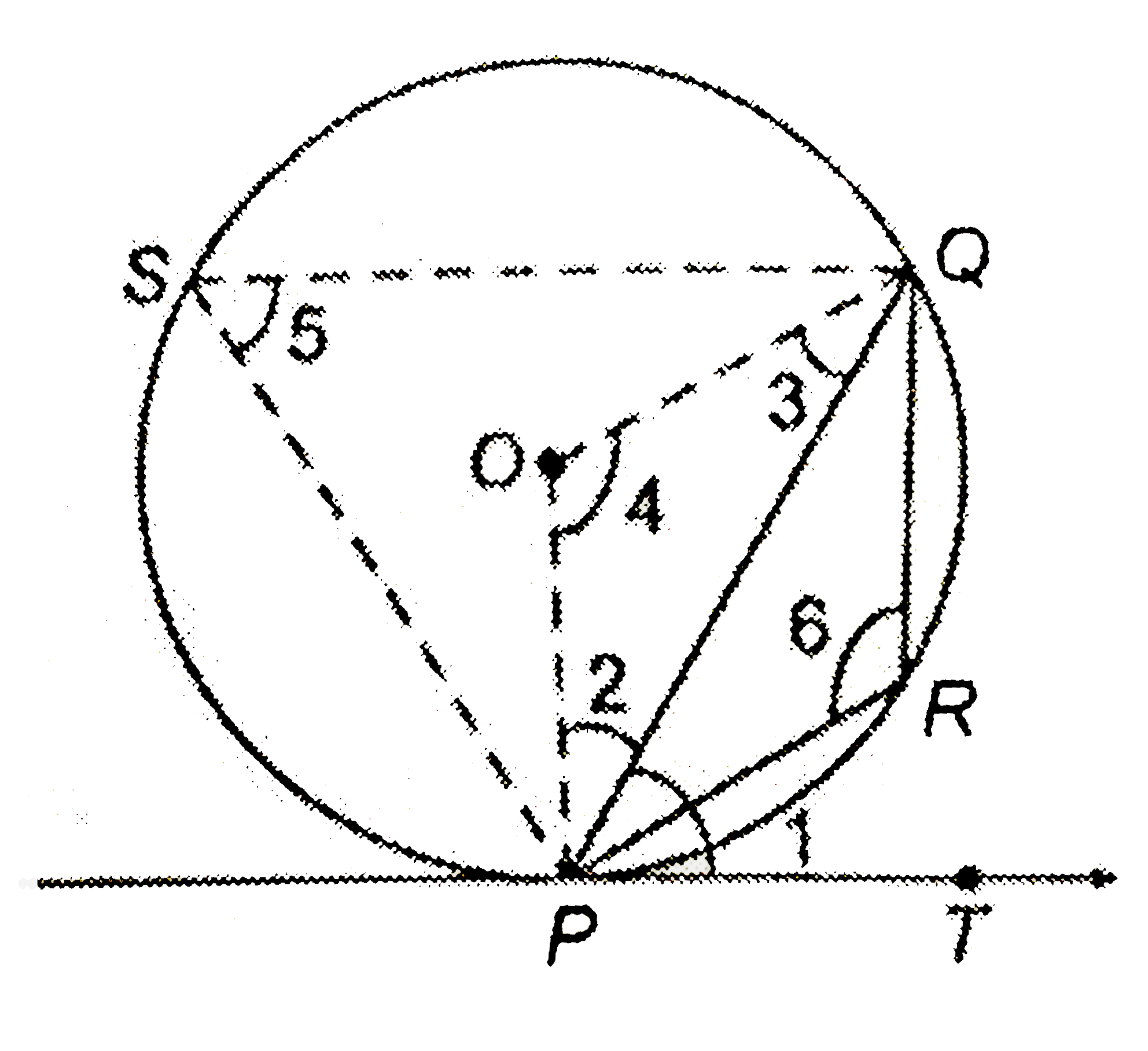
∴ ∠2+∠1=90∘
⇒ ∠2+60∘=90∘ (given)
⇒ ∠2=90∘−60∘=30∘ ...(1)
But OP=OQ (each radii)
∴ ∠2=∠3(angles opposite to equal sides are equal)
...(2)
Now in ΔPOQ,
∠2+∠3+∠4=180∘ (angle sum property)
⇒30∘+30∘+∠4=180∘ [from(1)and(2)]
⇒ ∠4=120∘
∴ ∠5=12×∠4
(∵ degree measure of an are is twice the angle subtended by it in alternate segment)
⇒ ∠5=12×120∘
⇒ ∠5−60∘
Also, ∠5+∠6=180∘ (Sum of opposit angles of a cyclic quad.)
⇒ 60∘+∠6=180∘
⇒ ∠6=∠PRQ=120∘
Topper's Solved these Questions
- CIRCLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLESEXERCISE - Problems From NCERT/exemplar
11 Videos
- CIRCLESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise
32 Videos
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Revision Exercise Long Answer Question
5 Videos
- CO-ORDINATE GEOMETRYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Revision Exercise Short Answer Questions
10 Videos
Similar Questions
In the adjoining figure find ∠x
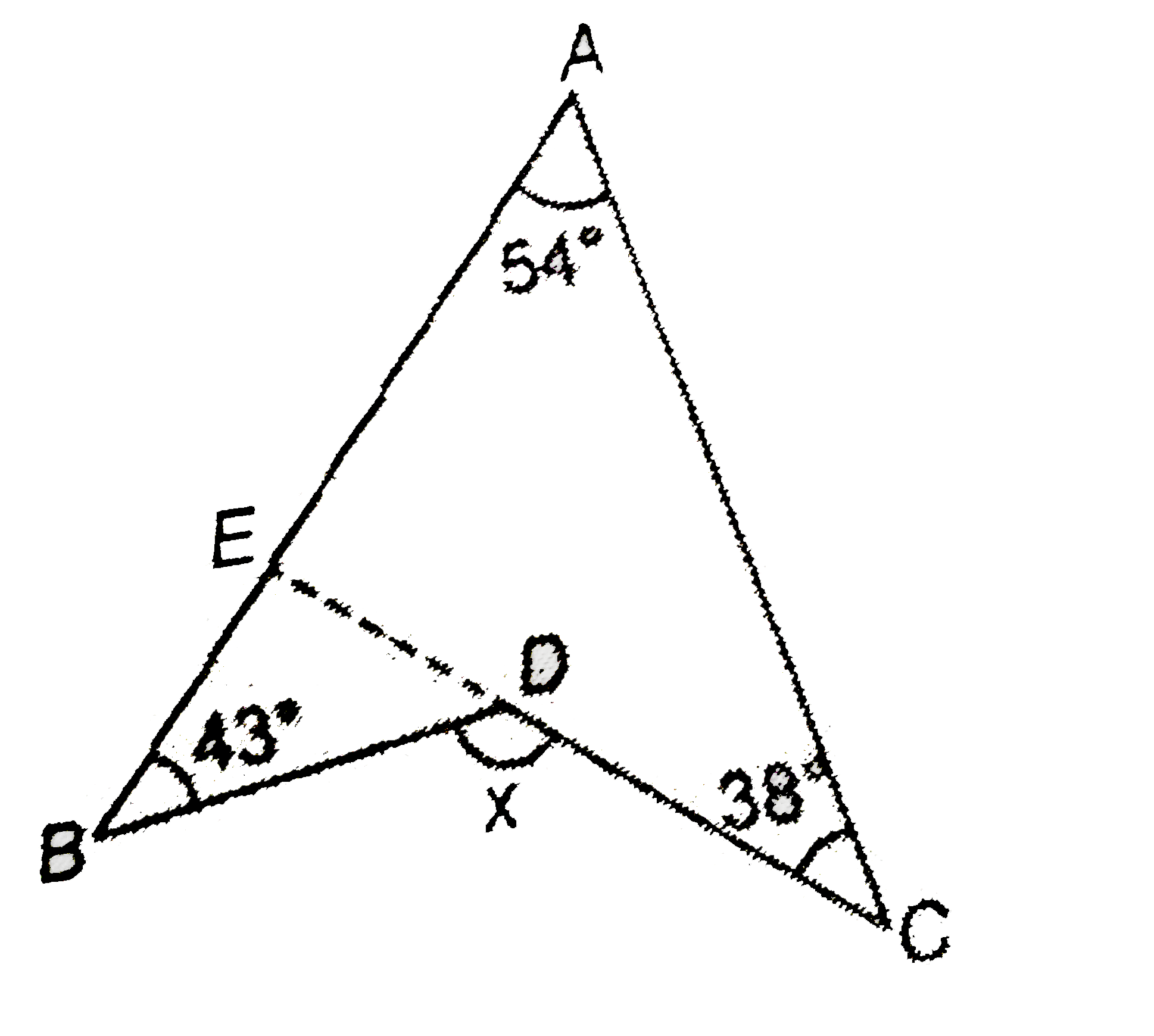
View SolutionIn the adjoining figure find ∠x
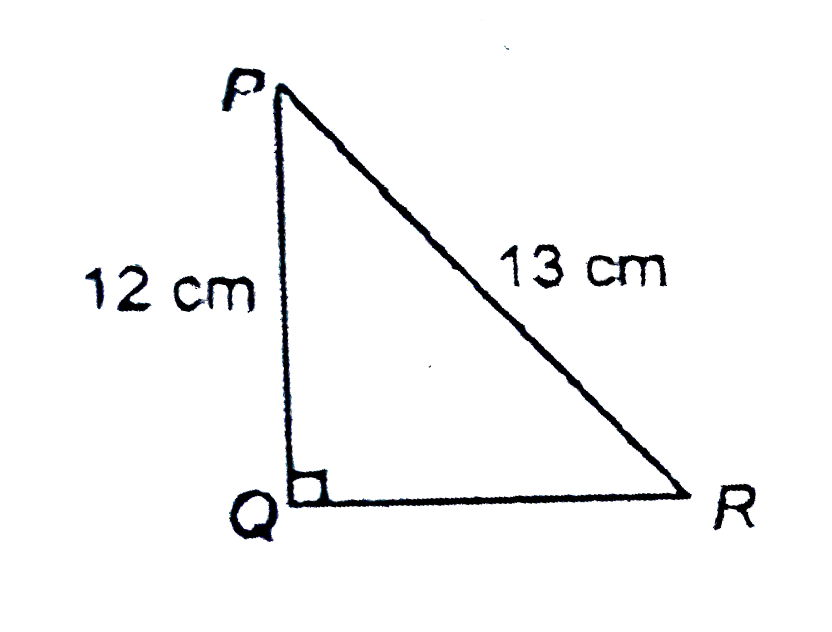
View SolutionIn the adjoining figure , find tan P -cot R.
View Solutionin the adjoining figure find ∠F.
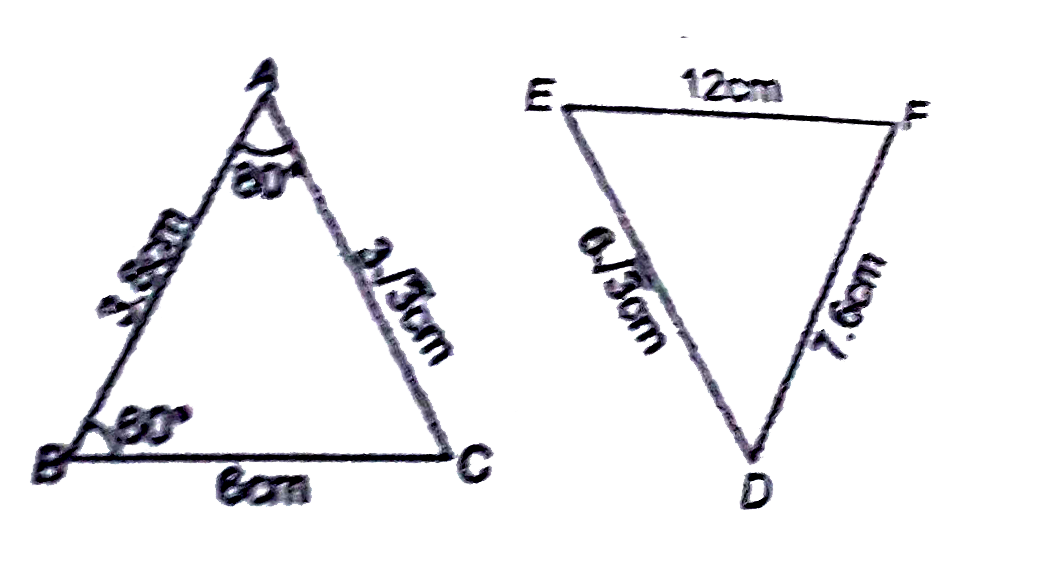
View SolutionIn the adjoining figure, find AC . (FIGURE)
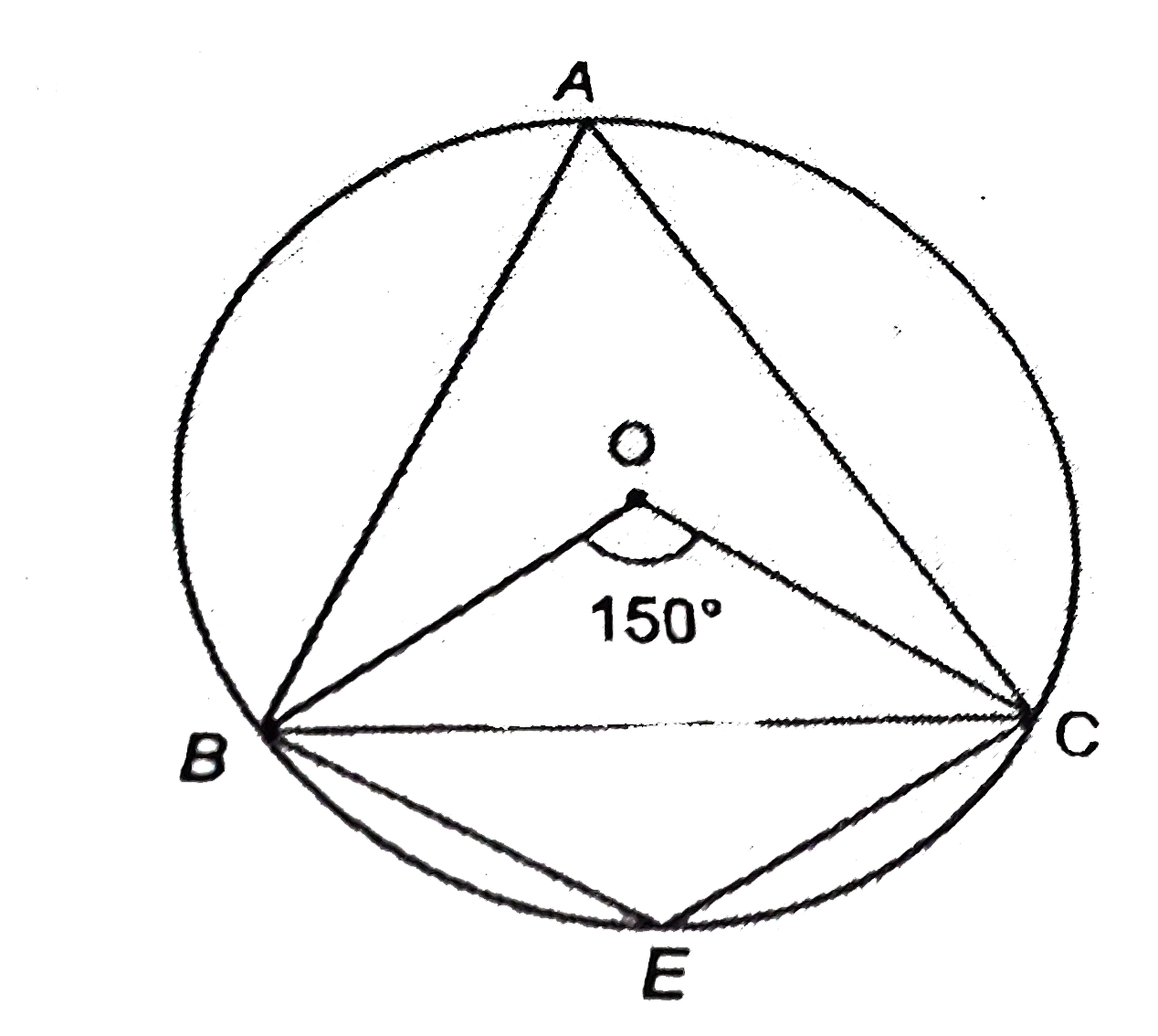
View SolutionIn the adjoining figure, O is the centre of the circle. Find the value of ∠BEC.
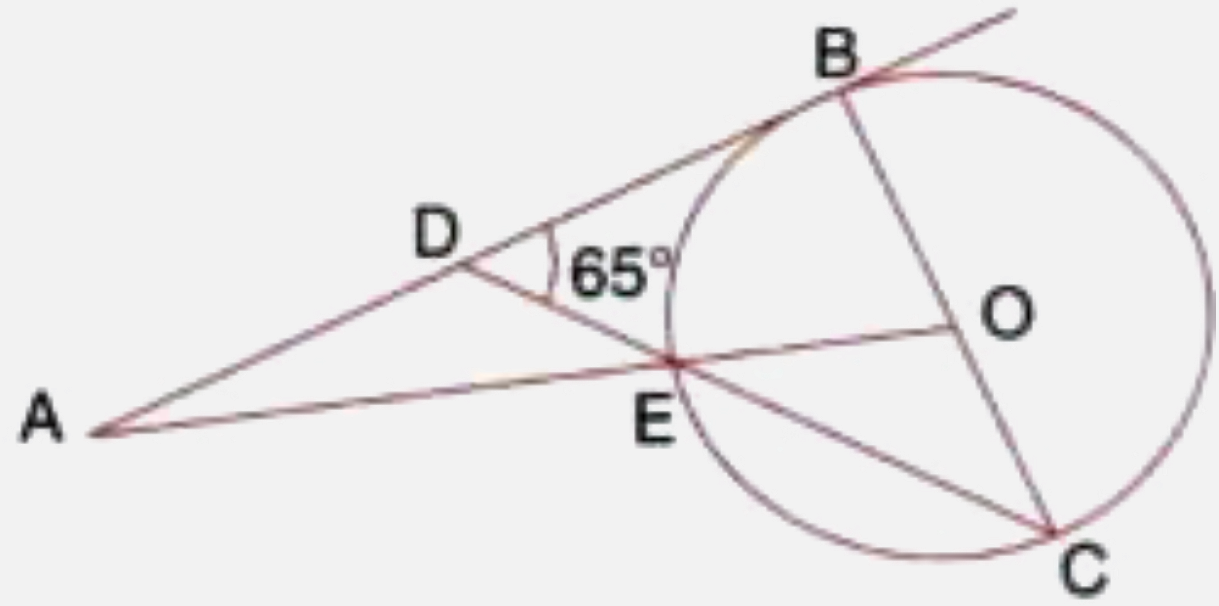
View SolutionIn the adjoining figvure O is the centre of the circle and AB is a tangent to it at point B.
∠BDC=65∘. Find ∠BAO
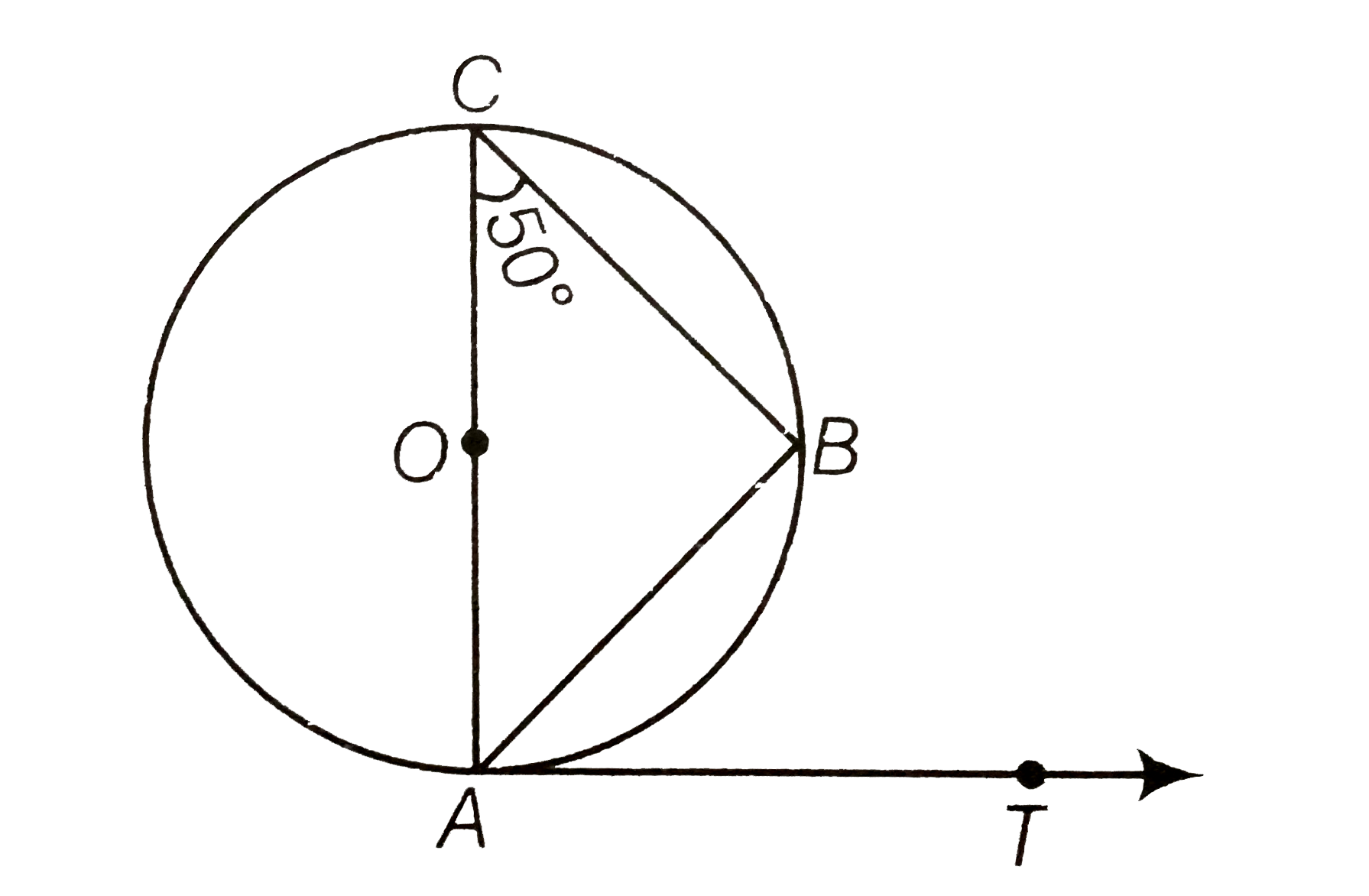
View SolutionIn figure, AB is a chord of the circle and AOC is the diameter such that ∠ACB=50∘. If AT is the tangent to the circle at the point A, then angleBAT is equal to : (a) 45∘ , (b) 60∘ , (c) 50∘ , (d) 55∘
View SolutionIn the adjoining figure , O is the centre of the circle and ∠OAB=60∘. Find ∠APC.

View SolutionIn figure, AB is a chord of the circle and AOC is its diameter such that ∠ACB=50∘. If AT is the tangent to the circle at the point A, then ∠BAT is equal to
View Solution
