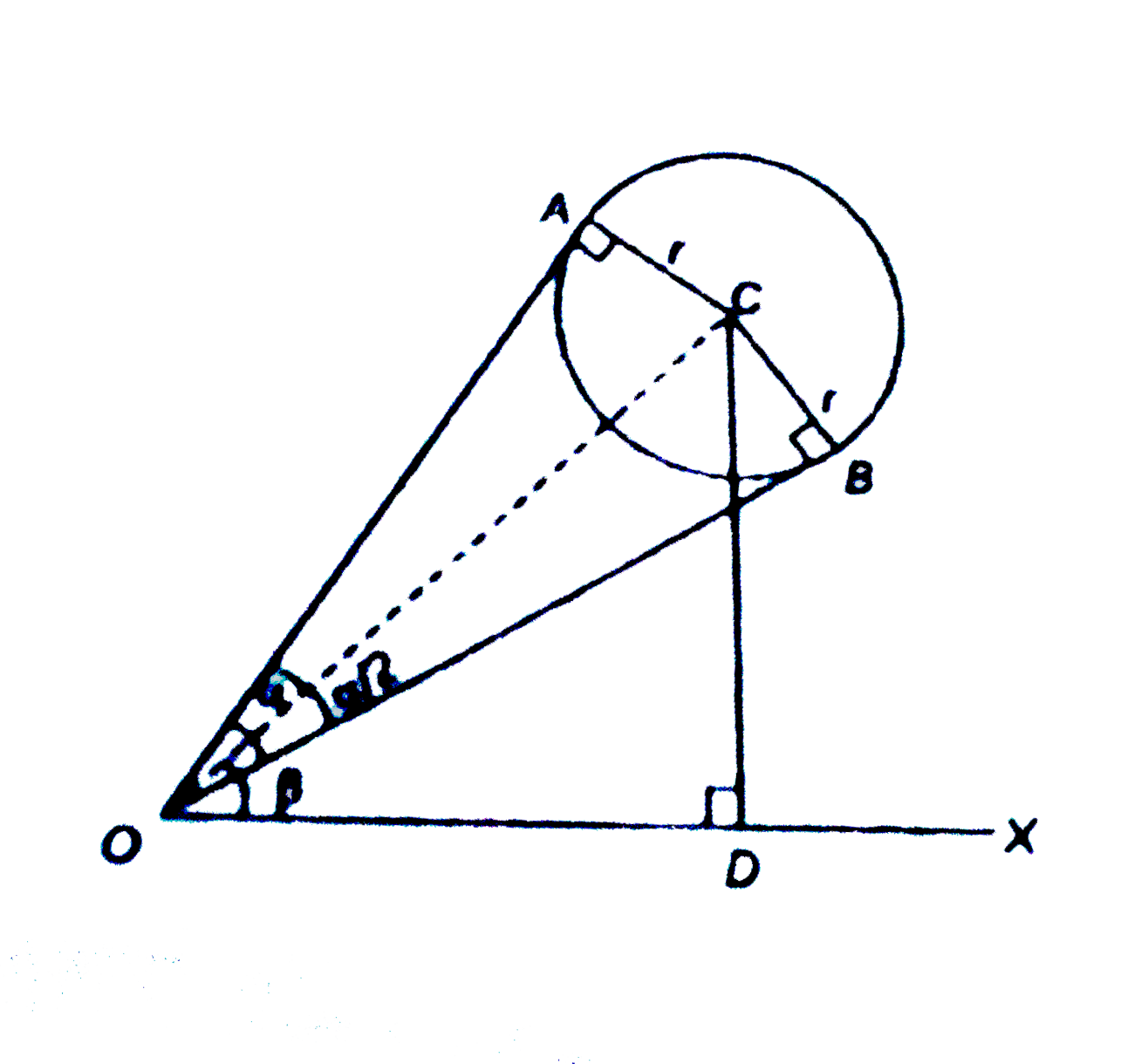
A round balloon of radius r subtends an angle at the eye of the observer while the angle of elevation of its centre is β. Prove that the height of the centre of the balloon is rsinβcosecα2.
Let C be the cerntre of the balloon of radius r and 'O' be the point of observation. OA and OB are the tangents from O to the circle.
Join CA, CB and CO.
Draw CD⊥OX.
Now, ∠AOB=α
⇒∠AOC=∠BOC=α2
(∴ tangent are equally inclined at the tangent)
∠DOC=β
( ∴ radius through point of contact is ⊥ to the tangent)
First we shall find OC with the help of BC = r and then with the help of OC, we shall find DC (Quite simple)
In right ΔOBC,
cosecα2=OCBC
OC=BC.cosecα2=rcosecα2
In right ΔODC,
sinβ=CDOC⇒CD=OCsinβ
CD=rcosecα2sinβ=rsinβcosecα2 [from(1)]
Hence, the height of the centre of the balloon from the ground is rsinβcosecα2.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Exercise32videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Revision Exercise (short Answer Questions)5videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - REAL NUMBERSEXERCISE - Revision Exercise Long Answer Questions5videos
REAL NUMBERS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions4videos
STATISTICS
Similar Questions
A balloon of radius r subtends an angleα at the eyes of an observer and the center of balloon from the eye is β . Find the height of the center of the balloon from the eye of observer .
View SolutionProve Equal chords of a circle subtend equal angles at the centre.
View SolutionAn arc of a circle of raduis R subtends an angle π2 at the centre. It carriers a current i. The magnetic field at the centre will be
View SolutionA tower subtends an angle α at a point A in the plane of its base and the angle of depression of the foot of the tower at a point b metres just above A is β . Prove that the height of the tower is btanαcotβ .
View SolutionIf the latusrectum subtends a right angle at the centre of the hyperbola then its eccentricity
View SolutionIn a circle of radius 10 cm, an arc subtends an angle of 108o at the centre. What is the area of the sector in terms of π ?
View SolutionA chord of a circle of radius 10cm subtends a right angle at the centre. The length of the chord (in cm) is
View SolutionIn a circle of radius 35 cm, an arc subtends an angle of 72o at the centre. Find the length of the arc and area of the sector.
View SolutionProve Equal chords of congruent circles subtend equal angles at the centre.
View SolutionAn arc of length 20π cm subtends an angle of 144o at the centre of a circle. Find the radius of the circle.
View Solution
