In the given figure △ACB~△APQ. If BC= 8cm , PQ = 4cm BA= 6.5 cm, AP = 2.8 cm, find CA and AQ.
To solve the problem, we will use the property of similar triangles, which states that the corresponding sides of similar triangles are in proportion.
1. Identify the Similar Triangles: We know that triangle ACB is similar to triangle APQ. This gives us the relationship between the sides of the triangles.
2. Write the Proportions: From the similarity of the triangles, we can set up the following proportions:
ACAP=BCPQandABAQ=BCPQ
3. Substitute Known Values: We know the following values:
- BC=8cm
- PQ=4cm
- BA=6.5cm
- AP=2.8cm
Using the first proportion:
AC2.8=84
4. Simplify the Proportion: The ratio 84 simplifies to 2:
AC2.8=2
5. Cross-Multiply to Solve for AC:
AC=2×2.8=5.6cm
6. Find AQ Using the Second Proportion: Now we will use the second proportion:
ABAQ=BCPQ
Substituting the known values:
6.5AQ=84
7. Simplify the Proportion: Again, 84 simplifies to 2:
6.5AQ=2
8. Cross-Multiply to Solve for AQ:
6.5=2×AQ⟹AQ=6.52=3.25cm
Final Answers:
- AC=5.6cm
- AQ=3.25cm
ACAP=BCPQandABAQ=BCPQ
- BC=8cm
- PQ=4cm
- BA=6.5cm
- AP=2.8cm
AC2.8=84
AC2.8=2
AC=2×2.8=5.6cm
ABAQ=BCPQ
Substituting the known values:
6.5AQ=84
6.5AQ=2
6.5=2×AQ⟹AQ=6.52=3.25cm
- AC=5.6cm
- AQ=3.25cm
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar14videos
TRIANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - TRIANGLES EXERCISE - Exercise 6a24videos
TRIANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions4videos
STATISTICS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions5videos
VOLUME AND SURFACE AREA OF SOLIDS
Similar Questions
In given figure, if ∠A=∠C, AB= 6 cm, BP = 15 cm, AP = 12 cm and CP= 4 cm, then find the lengths of PD and CD.
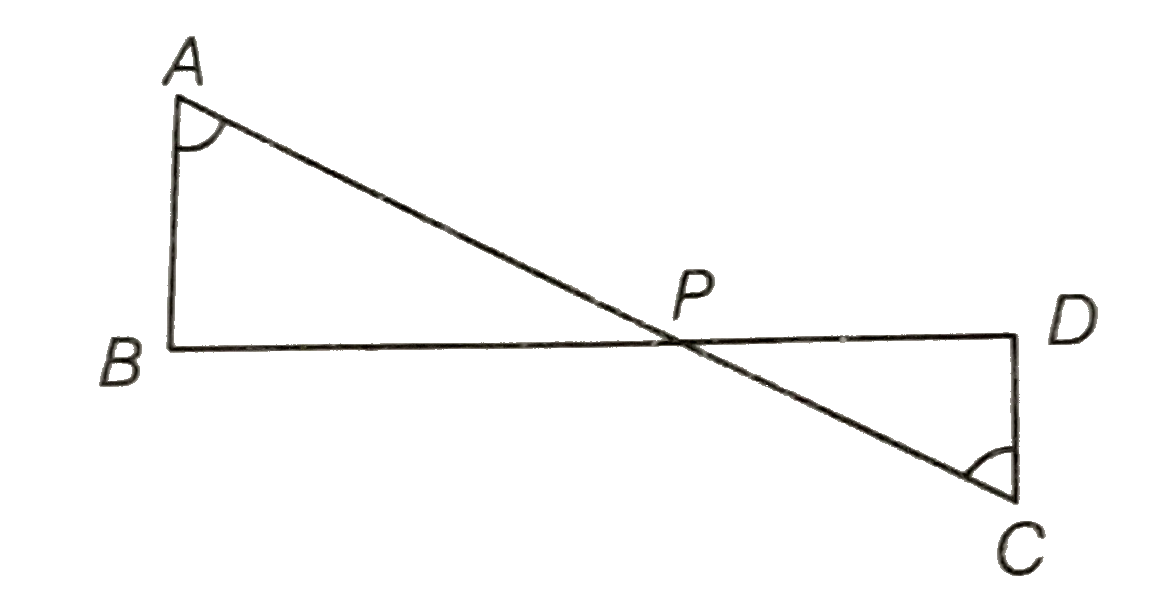 View Solution
View SolutionIn given figure, if ∠ACB=∠CDA,AC=8cm and AD=3 cm, then find BD.
View SolutionIn the given figure, if DE || BC, AE = 8 cm, EC = 2 cm and BC = 6 cm, then find DE
View SolutionConstruct a triangle ABC given that :
BC = 4 . 5 cm, AB = 5 . 2 cm and CA = 3 . 8 cmView SolutionIf P and Q are points on the sides AB and AC respeactively of △ABC, if PQ||BC,l AP= 2 cm , AB = 6 cm and AC= 9 cm find AQ.
View SolutionIn the given figure , ∠1=∠2and∠3=∠4. If BC= 7.5 cm , DE= 12.5cm and area of △ABC=13.5cm2 . Find the area of △ADE.
 View Solution
View SolutionIn the given figure, PQ//AB, CQ = 4.8 cm QB = 3.6 cm and AB = 6-3 cm. Find :

PQView SolutionConstruct a triangle ABC such that AB = 4 . 5 cm, BC = 6 cm and CA = 4 . 8 cm
View SolutionConstruct a triangle ABC given that :
AB = 5 cm, BC = 4 cm and CA = 6 cmView SolutionIn the given figure, AB//DC,BO=6cm and DQ=8cm, find: BP×DO.
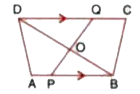 View Solution
View Solution

