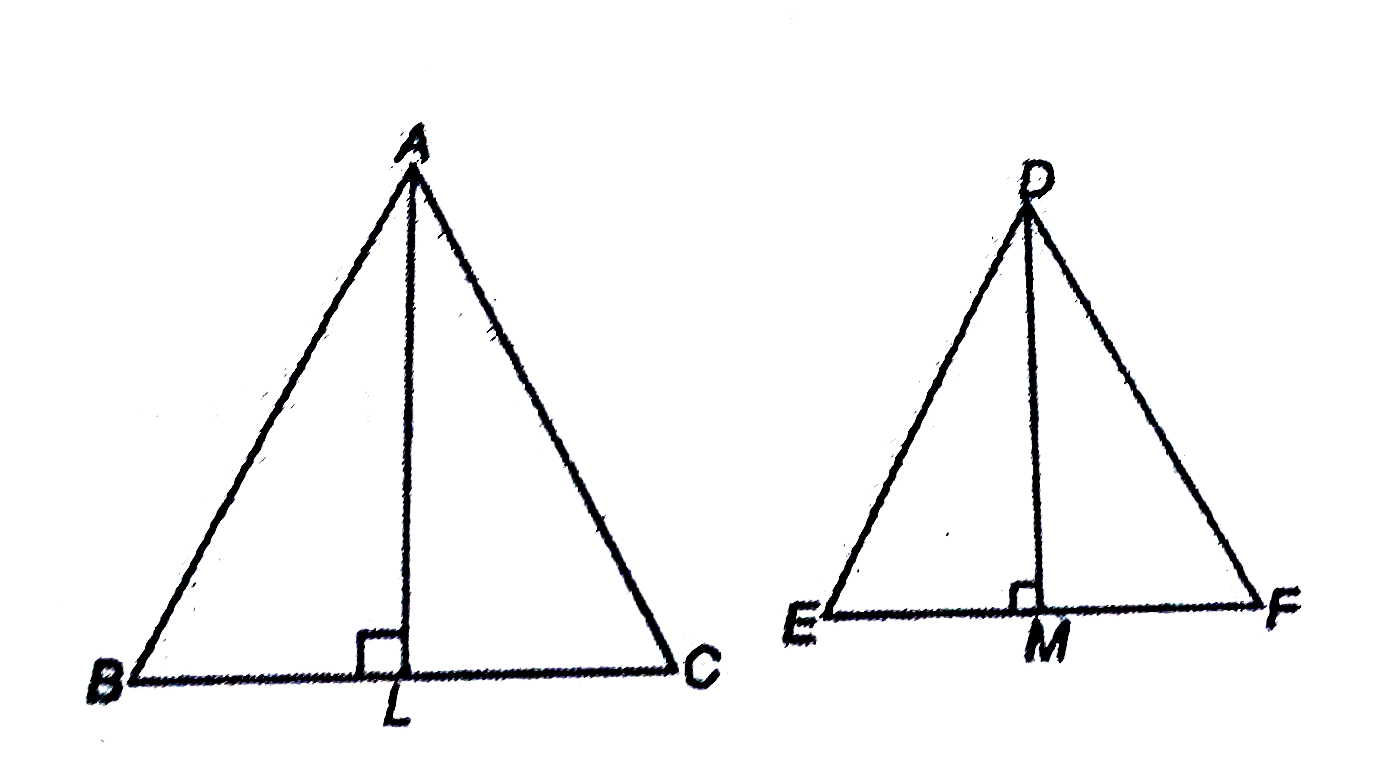
If two triangles are similar; prove that the ratio of corresponding area is equal to the ratio of squares corresponding altitudes.

Video Solution

Text Solution

Verified by Experts

Given : $△ A B C ~ △ D E F A L ⊥ B C and D M ⊥ E F$
To prove : $\frac{a r (△ A B C)}{a r (△ D E F)} = \frac{A L^{2}}{D M^{2}}$
proof: in $△ A L B and △ D M E$ ,
$∠ B = ∠ E (g i v e n △ A B C ~ △ D E F)$
$∠ A L B = ∠ D M E = 90^{\circ}$ (each)
$△ A B L ~ △ D E M$ (by AA similarity)
$\Rightarrow \frac{A B}{D E} = \frac{A L}{D M} . . (1)$
Now , $\frac{a r (△ A B C)}{a r (△ D E F)} = \frac{A B^{2}}{D E^{2}} ... (2)$
( the ratio of areas of two similar triangles is equal to ratio of squares of the corresponding sides)
Form (1) and (2) we have
$\frac{a r (△ A B C)}{a r (△ D E F)} = \frac{A L^{2}}{D M^{2}}$ Hence proved.

|

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

Similar Questions

NAGEEN PRAKASHAN ENGLISH-TRIANGLES -Revision Exercise Long Questions

Home
Ask Doubt
Profile