Calculate the magnetic field a point 10 cm from a North pole of strength 10 A-m.
(μ0=4π×10−7N−A−2)
Text Solution
Given, d=10cm=0.1m,
m=10A−m,
μ0=4π×10−7N−A2
and B=?
∴ Magnetic field due to an isolated magnetic dipole,
B=μ4π.mr2=10−7×10(0.1)2=10−4Wbm−2
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
The magnetic moment of a length 10 cm and pole strength 4.0 Am will be
A0.4Am2B1.6Am2C20Am2D8.0Am2 - Question 2 - Select One
A long straight wire is carrying a current of 12 A . The magnetic field at a distance of 8 cm is
(μ0=4π×10−7NA2)A2×10−4wb/m2B3×10−5Wb/m2C4×10−4Wb/m2D4×10−5Wb/m2 - Question 3 - Select One
The pole strength of 12cm long bar magnet is 20Am. The magnetic induction at a point 10 cm away from the centre of the magnet on its axial line is [μ04π=10−7H m−1]
A1.17×10−3 TB2.20×10−3 TC1.17×10−2 TD2.20×10−2 T
Find the magnetic field at point P due to a straight -line segment AB of length 6 cm carrying a current of 5A. (see figure) (μ0=4π×10−7N−A−2)
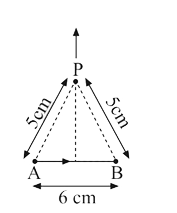 View Solution
View SolutionA current of 0.1A circulates around a coil of 100 turns and having a radius equal to 5cm. The magnetic field set up at the centre of the coil is
(μ0=4π×10−7 weber/amper-metre)View SolutionThe pole strength of 12 cm long bar magnet is 20 A m. The magnetic induction at a point 10 cm away from the centre of the magnet on its axial line is [μ04π=10−7H m−1]
View SolutionA small bar magnet has a magnetic moment 1.2A−m2. The magnetic field at a distance 0.1m on its axis will be:
(μ0=4π×10−7T−m/A)View SolutionA circular loop of radius 0.0157m carries a current of 2.0 amp. The magnetic field at the centre of the loop is
(μ0=4π×10−7weber/amp−m)View Solution

