Deduce the expression for the torque acting on a magnetic dipole placed in a uniform magnetism field?
Consider NS be a bar magnet of length 2l and strength of each pole = m. The magnet is held at ∠θ with the direction of magnetic field B.
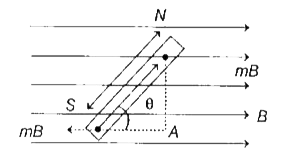
Force on N pole = mB, along B
Force on S pole = mB, opposite to B
where, m = strength of each pole and
and B = magnetic field induction.
These equal and unlike forces form a couple which tend to rotate the magnet clockwise, so as to align it along B.
Torque acting on the bar magnet,
τ = Force × Perpendicular distance
τ=mB×NA
In ΔNAS,sinθ=NANS=NA2l
NA=2lsinθ
Now, τ=mB×2lsinθ
=B×(m2l)sinθ
τ=MBsinθ
Similar Questions
Knowledge Check
- Question 1 - Select One
The torque acting on a magnetic dipole of moment Pm when placed in a magnetic field is
APmBB−→Pm×→BC−→Pm.→BDPm/B - Question 2 - Select One
The torque acting on a magnet of magnetic moment 'M' placed in a uniform magnetic field B is
Aperpendicular to →M, parallel to →BBparallel to →M, perpendicular to →BCperpendicular to both →Mand→BDparallel to both →Mand→B. - Question 3 - Select One
Torque acting on a current loop in a uniform magnetic field is equal to
A¯¯¯¯¯Pmׯ¯¯BB¯¯¯¯¯Pmׯ¯¯¯¯B2C¯¯¯¯¯Pm¯¯¯BD¯¯¯¯¯Pm¯¯¯¯¯B2
When is the torque acting on an electric dipole placed in a uniform electric field maximum?
View SolutionFind the expression of torque acting on an electric dipole placed in a uniform electric field . Using the above obtained expression of torque find the expression for potential energy of electric dipole kept in the uniform electric field . Assume potential energy of electric dipole to be zero when its axis is perpendicular to the externally applied electric field .
View SolutionTorque acting on a current loop in a uniform magnetic field is equal to
View SolutionDeduce the expression for the torque acting on a dipole of dipole moment →P in the presence of uniform electric field →E.
View SolutionDerive an expression for torque acting on a bar magnet held at an angle with the direction of a uniform magnetic field.
View Solution

