Find out an expression for energy stored in an inductor.
Let I be the instantaneous current at a time t, when the current is set up.
The back emf - VL produced in the inductor at the moment is −Ldidt.
∴ VL=Ldidt
Thus, work done by the source of emf to overcome the back emf and maintain the charge flow is given by
dW=VL=Ldidt⇒dW=L
If t0 is the time required for the current to come to its steady value I, then work done during it is given by
W=∫W0dW=∫I0L⇒W=12LI2=UB
Thus, UB is the stored magnetic energy in the inductor.
Similar Questions
Knowledge Check
A circuit contains an inductor of value 2H as shown at t=0 no current was flowing through battery. Find the energy stored in inductor at t=4 sec.
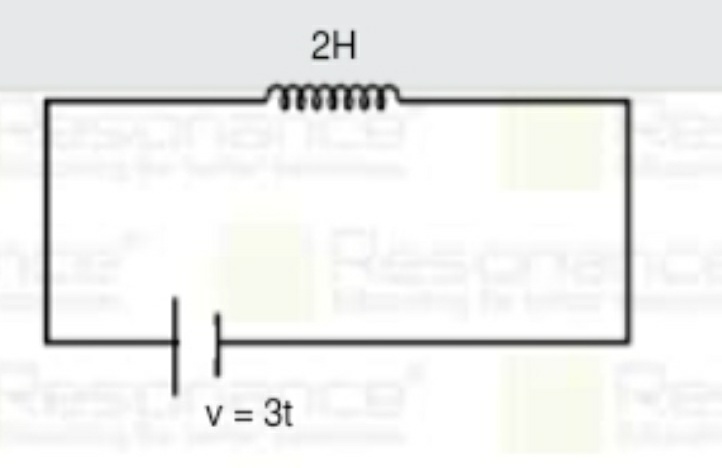 A144JB150JC160JD180J
A144JB150JC160JD180J
Obtain the expression for the magnetic energy stored in an ideal inductor of self inductance L when a current I passes through it.
Hence obtain the expression for the energy density of magentic field produced in the inductor.
View SolutionObtain the expression for the magnetic energy stored in an ideal inductor of self inductance L when a current I passes through it.
Hence obtain the expression for the energy density of magentic field produced in the inductor.
View SolutionIn the steady state of the circuit shown in the figure the ratio of energy stored in the inductor to th energy stored in the capacitor is 10000.
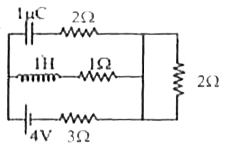
View SolutionIn the steady state of the circuit shown in the figure the ratio of energy stored in the inductor to th energy stored in the capacitor is 1000000.

View SolutionReferring to the previous illustration find the energy stored in the inductor when the current l is dropped to a value of 5A .
View SolutionTick the correct option .Energy stored in an inductor reside in it is in the form of
View SolutionConsider the given circuit.Initially there is no energy stored in inductor and capacitor.At
View Solution
