State and explain Biot-Savart.s law.

Text Solution

Verified by Experts

Biot-Savart.s Law This law deals with the magnetic field induction at a point due to a small current element (a part of any conductor, carrying current). Let XY be current carrying conductor, I be current in the conductor, dl be infinitesimal small element of the conductor, let dB be magnetic field at point P at a distance r from the element. According to Biot-Savart.s law, the magnitude of magnetic field induction (dB) at a point P due to a current element depends on the following factors:
(i) $d B \propto l$ (i.e. magnetic field is directly proportional to the current flowing through the conductor). (ii) $d B \propto d l$ (i.e. magnetic field is directly proportional to the length of the element). (iii) $d B \propto sin θ$ (i.e. magnetic field is directly proportional to the sine of angle between the length of element and line joining the element to point (P). (iv) $d B \propto \frac{1}{r^{2}}$ (i.e. magnetic field is inversely proportional to the square of distance between the element and point P). Combining all the above relations,
$d B \propto \frac{I d l sin sin θ}{r^{2}}$
This relation is called Biot-Savart.s law.

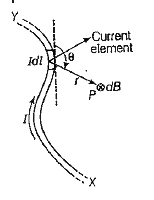
In vector form, Biot-Savart.s law can be written as
$d B \propto \frac{I d l \times r}{r^{3}} = \frac{μ_{0}}{4 π} . \frac{I d l \times r}{r^{3}}$

Updated on:21/07/2023

Which of the following relations represent Biot-Savart's law?
A $d \to B = \frac{μ_{0}}{4 π} I \frac{d \to l \times \to r}{r}$
B $d \to B = \frac{μ_{0}}{4 π} I \frac{d \to l \times \to r}{r^{2}}$
C $d \to B = \frac{μ_{0}}{4 π} I \frac{d \to l \times \to r}{r^{3}}$
D $d \to B = \frac{μ_{0}}{4 π} I \frac{d \to l \times \to r}{r^{4}}$
Which of the following gives the value of magnetic field according to, Biot-Savart’s law
A $\frac{i △ l sin θ}{r^{2}}$
B $\frac{μ_{0}}{4 π} \frac{i △ l sin θ}{r}$
C $\frac{μ_{0}}{4 π} \frac{i △ l sin θ}{r^{2}}$
D $\frac{μ_{0}}{4 π} i △ l sin θ$