(i) State Biot-Savart.s law.
(ii) Find magnetic flux density at a point on the axis of a long solenoid having 5000 turns m when it carrying a current of 2 A.
Step-by-Step Solution
1. Calculate B:
B=μ0nI=(4π×10−7T m/A)×(5000turns/m)×(2A)
2. Simplifying:
B=(4π×10−7)×10000=4π×10−3T
3. Approximating π as 3.14:
B≈4×3.14×10−3≈12.56×10−3T=0.01256T
Thus, the magnetic flux density at a point on the axis of the long solenoid is approximately 0.01256T or 12.56mT.
B=μ0nI=(4π×10−7T m/A)×(5000turns/m)×(2A)
B=(4π×10−7)×10000=4π×10−3T
B≈4×3.14×10−3≈12.56×10−3T=0.01256T
Similar Questions
Knowledge Check
- Question 1 - Select One
The torque required to hold a small circular coil of 10 turns, area 1mm2 and carrying a current of (2144)A in the middle of a long solenoid of 103turns/m carrying a current of 2.5A, with its axis perpendicular to the axis of the solenoid is
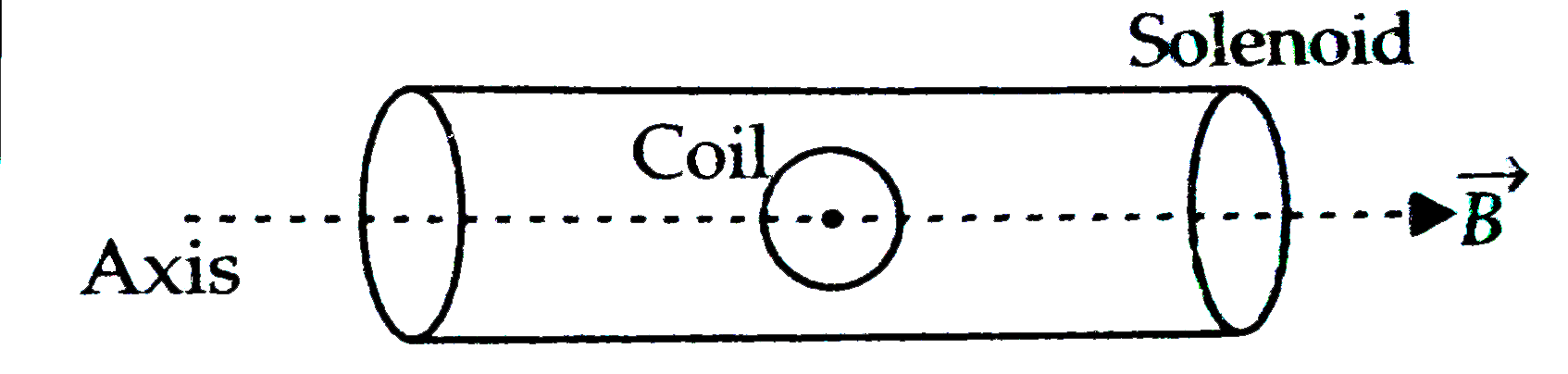 A1.5×10−6NmB1.5×10−8NmC1.5×106NmD1.5×108Nm
A1.5×10−6NmB1.5×10−8NmC1.5×106NmD1.5×108Nm
Obtain an expression for magnetic flux density B at the centre of a circular coil of radius R, having N turns and carrying a current I.
View SolutionObtain an expression for magnetic flux density B at the centre of a circular coil of radius R, having N turns and carrying a current I.
View SolutionThe magnetic flux density at a point distant d from a long straight current carrying conductor is B, then its value at distance d2 will be-
View Solution(a) Using Ampere.s circuital law or Biot Savart.s law, show that magnetic flux density .B. at a point P at a perpendicular distance.d from a long current carrying conductor is given by: B=(μ04π)2Ia (Statement of the law is not required)
(b) Define the terms (i) drift velocity, (ii) relaxation time. A conductor of length L is connected to a d.c. source of emf E. If this conductor is replaced by another conductor of same material and same area of cross-section but of length 3L, how will the drift velocity change?View SolutionUsing Ampere 's circuital law or Biot- Savart's a law, show that magnetic flux density B at point P at a perpendicular distance a from a long current carrying conductor is given by B=(μ4π)2Ia [ Statement of the laws not required]
View SolutionFind the magnetic intensity H at the centre of a long solenoid having n turns per unit length and carrying a current i (a) when no material is kept in it and (b) when a long copper rod is inserted in the solenoid.
View SolutionCalculate magnetic flux density of the magnetic field at the centre of a circular coil of 50 turns, having radius of 0.5 m and carrying a current of 5A.
View Solution