A conducting ring of radius r and resistance R is placed in region of uniform time varying magnetic field B which is perpendicular to the plane of the ring. It the magnetic field is changing at a rate α, then the current induced in the ring is
πr2α2R
2πrαR
πrαR
πr2αR
The correct Answer is:D
To find the induced current in a conducting ring placed in a time-varying magnetic field, we can follow these steps:
1. Identify the Given Information:
- Radius of the ring: r
- Resistance of the ring: R
- Rate of change of magnetic field: α (i.e., dBdt=α)
2. Understand the Concept of Induced EMF:
- According to Faraday's law of electromagnetic induction, the induced electromotive force (EMF) in a closed loop is equal to the negative rate of change of magnetic flux through the loop.
- The formula for induced EMF (E) is given by:
E=−dΦdt
- Where Φ is the magnetic flux given by Φ=B⋅A⋅cos(θ). Here, A is the area of the ring and θ is the angle between the magnetic field and the normal to the surface of the ring.
3. Calculate the Area of the Ring:
- The area A of the ring is given by:
A=πr2
4. Determine the Magnetic Flux:
- Since the magnetic field B is perpendicular to the plane of the ring, θ=0 and cos(0)=1.
- Thus, the magnetic flux becomes:
Φ=B⋅A=B⋅πr2
5. Calculate the Rate of Change of Magnetic Flux:
- The rate of change of magnetic flux is:
dΦdt=d(B⋅πr2)dt=πr2dBdt=πr2α
6. Find the Induced EMF:
- Substituting into the induced EMF formula:
E=−dΦdt=−πr2α
- The magnitude of the induced EMF is:
|E|=πr2α
7. Calculate the Induced Current:
- Using Ohm's law, the induced current I can be calculated as:
I=ER=πr2αR
Final Answer:
The induced current in the ring is:
I=πr2αR
- Radius of the ring: r
- Resistance of the ring: R
- Rate of change of magnetic field: α (i.e., dBdt=α)
- According to Faraday's law of electromagnetic induction, the induced electromotive force (EMF) in a closed loop is equal to the negative rate of change of magnetic flux through the loop.
- The formula for induced EMF (E) is given by:
E=−dΦdt
- Where Φ is the magnetic flux given by Φ=B⋅A⋅cos(θ). Here, A is the area of the ring and θ is the angle between the magnetic field and the normal to the surface of the ring.
- The area A of the ring is given by:
A=πr2
- Since the magnetic field B is perpendicular to the plane of the ring, θ=0 and cos(0)=1.
- Thus, the magnetic flux becomes:
Φ=B⋅A=B⋅πr2
- The rate of change of magnetic flux is:
dΦdt=d(B⋅πr2)dt=πr2dBdt=πr2α
- Substituting into the induced EMF formula:
E=−dΦdt=−πr2α
- The magnitude of the induced EMF is:
|E|=πr2α
- Using Ohm's law, the induced current I can be calculated as:
I=ER=πr2αR
The induced current in the ring is:
I=πr2αR
Similar Questions
The figure shows a conducting ring of radius R. A uniform steady magnetic field B lies perpendicular to the plane of the ring a circular region r(<R). If the resistance per unit length of the ring is λ, then the current induced in the ring when its radius gets doubled is

View Solutionthe uniform magnetic field perpendicular to the plane of a conducting ring of radius a change at the rate of α, then
View Solution
Knowledge Check
A conducting circular loop of radius r carries a constant current i. It is placed in a uniform magnetic field B such that B is perpendicular to the plane of loop. What is the magnetic force acting on the loop?
AI r BB2πrIBCzeroDπrIBA conducting ring is placed in a uniform magnetic field with its plane perpendicular to the field . An emf is induced in the ring if
Ait is rotated about its axis.Bit is rotated about its diameter.Cit is not moved.Dit is given translational motion in the field.
A vertical conducting ring of radius R falls vertically with a speed V in a horizontal uniform magnetic field B which is perpendicular to the plane of the ring. Which of the following statements is correct?
View Solutionthe uniform magnetic field perpendicualr to the plane of a conducting ring of radius a change at the rate of α, then
View SolutionA rectangular coil is placed in a region having a uniform magnetic field B perpendicular to the plane of the coil. An emf will not be induced ion the coil if the

View SolutionThe magnetic field perpendicular to the plane of a conducting ring of radius r changes at the rate.dBdt . Then
View SolutionA ring of mass m and radius r is rotated in uniform magnetic field B which is perpendicular to the plane of the loop with constant angular velocity ω0.Find the net ampere force on the ring and the tension developed in the ring if there is a current i in the ring.Current and rotation both are clockwise.
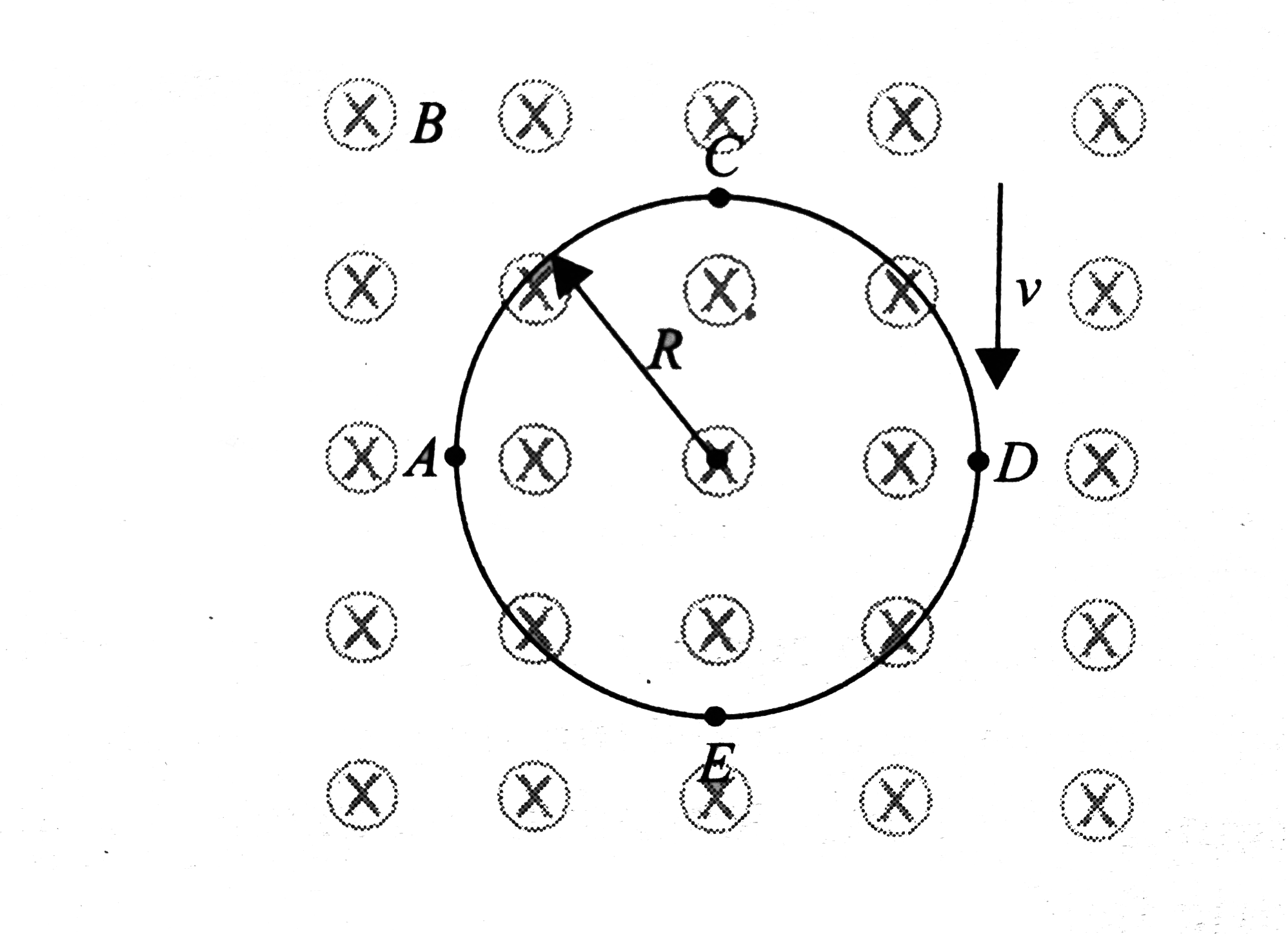
View SolutionA vertical conducting ring ogradius R falls vertically in a horizontal magnetic field of magnitude B. The direction of B is perpendicular to the plane of the ring. When the speed of the ring is v,
View Solution
