Two particles of equal masses have velocities →v1=4ˆims−1 and →v2=4ˆjms−1. First particle has an acceleration →a1=(5ˆi+5ˆj)ms−2, while the acceleration of the other particle is zero. The centre of mass of the two particles moves in a path of
Straight line
Parabola
Circle
Ellipse
The correct Answer is:A
To solve the problem, we need to find the path of the center of mass of two particles with given velocities and accelerations.
1. Identify Given Data:
- For Particle 1:
- Velocity: →v1=4^im/s
- Acceleration: →a1=5^i+5^jm/s2
- For Particle 2:
- Velocity: →v2=4^jm/s
- Acceleration: →a2=0m/s2
2. Calculate the Center of Mass Velocity (→vcm):
- Since both particles have equal masses, we can use the formula for the velocity of the center of mass:
→vcm=m1→v1+m2→v2m1+m2
- Let m1=m2=m:
→vcm=m→v1+m→v2m+m=→v1+→v22
- Substitute the values:
→vcm=4^i+4^j2=2^i+2^jm/s
3. Calculate the Center of Mass Acceleration (→acm):
- The acceleration of the center of mass is given by:
→acm=→a1+→a22
- Substitute the values:
→acm=(5^i+5^j)+02=5^i+5^j2=52^i+52^jm/s2
4. Determine the Path of the Center of Mass:
- Since the center of mass has a constant acceleration →acm and its velocity →vcm is also constant, the center of mass will move in a straight line.
- The direction of the motion can be determined from the acceleration vector, which is in the direction of ^i+^j.
5. Conclusion:
- The center of mass of the two particles moves in a straight line.
- For Particle 1:
- Velocity: →v1=4^im/s
- Acceleration: →a1=5^i+5^jm/s2
- For Particle 2:
- Velocity: →v2=4^jm/s
- Acceleration: →a2=0m/s2
- Since both particles have equal masses, we can use the formula for the velocity of the center of mass:
→vcm=m1→v1+m2→v2m1+m2
- Let m1=m2=m:
→vcm=m→v1+m→v2m+m=→v1+→v22
- Substitute the values:
→vcm=4^i+4^j2=2^i+2^jm/s
- The acceleration of the center of mass is given by:
→acm=→a1+→a22
- Substitute the values:
→acm=(5^i+5^j)+02=5^i+5^j2=52^i+52^jm/s2
- Since the center of mass has a constant acceleration →acm and its velocity →vcm is also constant, the center of mass will move in a straight line.
- The direction of the motion can be determined from the acceleration vector, which is in the direction of ^i+^j.
- The center of mass of the two particles moves in a straight line.
Similar Questions
Two particles of equal mass have velocities →v1=2ˆi=m/s−1 and →v2=2ˆjm/s−1. First particle has an acceleration →a1=(3ˆi+3ˆj)ms−2 while the acceleration of the other particle is zero. The centre of mass of the two particles moves in a path of.
View SolutionA particle moves with a tangential acceleration at=→a.ˆv where →a=(5ˆi)m/s2. If the speed of the particle is zero at x=0, then find v (in m/s) at x=4.9m.
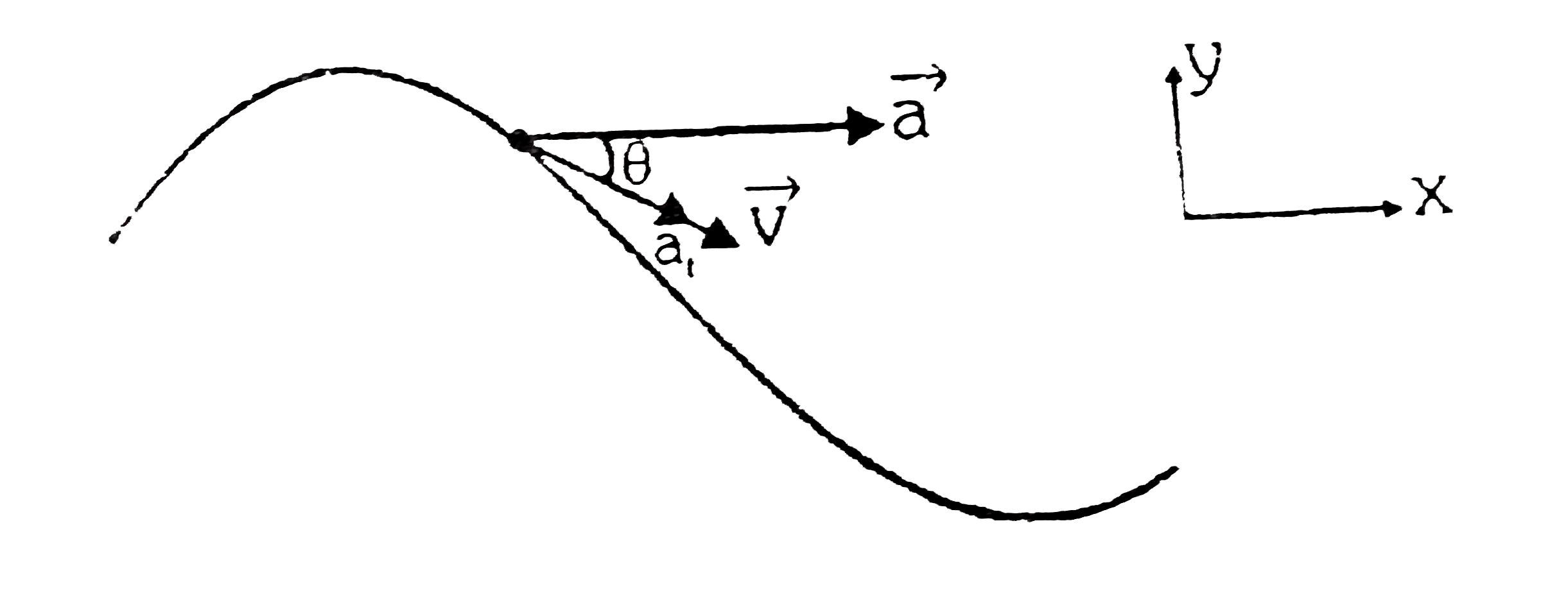
View Solution
Knowledge Check
Two particles of equal mass have velocities v1=2ims−1 and v2=2jms−1 First particle has an acceleration a=(3i+3j)ms−2 while the acceleration of the other particle is zero. The centre of mass of the two particles moves in a path of
Aa.straight lineBb.parabolaCc.circleDd.ellipse
A particle's velocity changes from (2ˆI+3ˆj)ms−1 in to (3ˆi−2ˆj)ms−1 in 2s. Its average acceleration in ms−2 is
View SolutionA charged particle has acceleration →a=2ˆi+xˆj in a megnetic field →B=−3ˆi+2ˆj−4ˆk. Find the value of x.
View SolutionTwo particles of masses m and 2 m has initial velocity →u1=2ˆi+3ˆjms and →u2=−4ˆi+3ˆjms Respectivley. These particles have constant acceleration →a1=4ˆi+3ˆj(ms2) and →a2=−4ˆi−2ˆj(ms2) Respectively. Path of the centre of the centre of mass of this two particle system will be:
View SolutionTwo particles of equal mass have coordinates (2m,4m,6m) and (6m,2m,8m). Of these one particle has a velocity v1=(2i)ms−1 and another particle has a velocity v2=(2j)ms−1 at time t=0. The coordinate of their center of mass at time t=1s will be
View SolutionA particle leaves the origin with an lintial veloity →u=(3ˆi) and a constant acceleration →a=(−1.0ˆi−05ˆj)ms−1. Its velocity →v and position vector →r when it reaches its maximum x-coordinate aer .
View SolutionA particle leaves the origin with initial velocity →v0=11ˆi+14ˆjm/s. It undergoes a constant acceleration given by →a=−225ˆi+215ˆjm/s2.
When does the particle cross the y-axis ?
View SolutionA particle of mass 2kg moves with an initial velocity of →v=4ˆi+4ˆjms−1. A constant force of →F=20ˆjN is applied on the particle. Initially, the particle was at (0,0). The x-coordinates of the particle when its y-coordinates again becomes zero is given by
View Solution
