Question

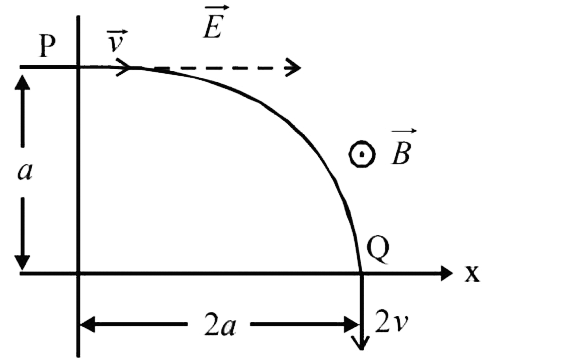
A particle ( mass = m and charge = q ) is moving in a region in which there exists a uniform electric field $E\overrightarrow{i}$ and a uniform magnetic field $B\hat{k}$. At t = 0 , the particle is at ( 0,a ) and is moving with $v\hat{i}$. After some time, the particle is located at ( 2a, 0 ) and has a velocity $-2v\hat{j}$ , then which of the following is true ?

Options

A. `E = (3)/( 2) (( mv^(2))/(qa))`
B. at the initial moment, the rate of work done by electric field is ` ( 3)/( 4) ((m v^(3))/( a))`
C. `E = ( 3)/( 8) ((mv^(2))/( qa))`
D. at the initial moment, the rate of work done by electric field is `( 3)/( 16) ((mv^(3))/( a ))`

Answer

B

Explanation

To solve the problem, we need to analyze the motion of the charged particle in the presence of both electric and magnetic fields.

1. Identify Initial and Final Conditions:
- Initial position: \( (0, a) \)
- Initial velocity: \( \vec{v_i} = v \hat{i} \)
- Final position: \( (2a, 0) \)
- Final velocity: \( \vec{v_f} = -2v \hat{j} \)

2. Determine the Displacement:
- Displacement \( \Delta \vec{r} = \vec{r_f} - \vec{r_i} = (2a, 0) - (0, a) = (2a, -a) \).

3. Calculate the Change in Kinetic Energy:
- Initial kinetic energy \( KE_i = \frac{1}{2} m v^2 \).
- Final kinetic energy \( KE_f = \frac{1}{2} m (-2v)^2 = \frac{1}{2} m (4v^2) = 2mv^2 \).
- Change in kinetic energy \( \Delta KE = KE_f - KE_i = 2mv^2 - \frac{1}{2} mv^2 = \frac{4mv^2}{2} - \frac{1}{2} mv^2 = \frac{3}{2} mv^2 \).

4. Work Done by Electric Field:
- The work done by the electric field \( W = \Delta KE \).
- Therefore, \( W = \frac{3}{2} mv^2 \).

5. Relate Work Done to Electric Field:
- The work done by the electric field can also be expressed as \( W = qE \cdot \Delta \vec{r} \).
- Since \( \Delta \vec{r} = (2a, -a) \) and \( \vec{E} = E \hat{i} \), we have:
\( W = qE \cdot (2a \hat{i} - a \hat{j}) = qE (2a). \)
- Therefore, equating the two expressions for work done:
\( qE (2a) = \frac{3}{2} mv^2. \)
- This gives us:
\( E = \frac{3mv^2}{2qa}. \)

6. Calculate Power:
- Power \( P \) is given by \( P = \vec{F} \cdot \vec{v} \).
- The force due to the electric field is \( \vec{F} = qE \hat{i} \).
- The velocity \( \vec{v} = v \hat{i} \).
- Therefore, the power is:
\( P = qE \cdot v = q \left(\frac{3mv^2}{2qa}\right) v = \frac{3mv^3}{2a}. \)

Conclusion:
The correct expression for the power is \( P = \frac{3}{2} \frac{mv^3}{a} \).