A man is standing between a stationary source and cliff. When he starts moving along line joining him and source, he hears 10 beats per second. The velocity of man is [frequency of source=600Hz, velocity of sound=330ms−1]
5.5 m/s
11m/s
16/5m/s
2.75m/s
The correct Answer is:D
To solve the problem, we will use the concept of the Doppler effect and the information given about beats. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Problem
We have a stationary sound source emitting a frequency fs=600Hz and a man moving towards the source. The speed of sound is v=330m/s. The man hears 10 beats per second, which indicates that there are two different frequencies being heard: one from the source and one reflected off the cliff.
Step 2: Identify the Frequencies
1. Frequency heard directly from the source (f1):
f1=fsv−vmv
where vm is the velocity of the man.
2. Frequency heard after reflecting off the cliff (f2):
f2=fsv+vmv
Step 3: Set Up the Beat Frequency Equation
The beat frequency fb is given by the difference between the two frequencies:
fb=|f2−f1|=10Hz
Substituting the expressions for f1 and f2:
10=(fsv+vmv−fsv−vmv)
Step 4: Simplify the Equation
Factoring out fs:
10=fs((v+vm)−(v−vm)v)
This simplifies to:
10=fs(2vmv)
Substituting fs=600Hz:
10=600(2vm330)
Step 5: Solve for the Velocity of the Man
Rearranging the equation gives:
10=1200vm330
Multiplying both sides by 330:
3300=1200vm
Now, solving for vm:
vm=33001200=114m/s
Calculating the numerical value:
vm=2.75m/s
Final Answer
The velocity of the man is 2.75m/s.
---
We have a stationary sound source emitting a frequency fs=600Hz and a man moving towards the source. The speed of sound is v=330m/s. The man hears 10 beats per second, which indicates that there are two different frequencies being heard: one from the source and one reflected off the cliff.
1. Frequency heard directly from the source (f1):
f1=fsv−vmv
where vm is the velocity of the man.
f2=fsv+vmv
The beat frequency fb is given by the difference between the two frequencies:
fb=|f2−f1|=10Hz
Substituting the expressions for f1 and f2:
10=(fsv+vmv−fsv−vmv)
Factoring out fs:
10=fs((v+vm)−(v−vm)v)
This simplifies to:
10=fs(2vmv)
Substituting fs=600Hz:
10=600(2vm330)
Rearranging the equation gives:
10=1200vm330
Multiplying both sides by 330:
3300=1200vm
Now, solving for vm:
vm=33001200=114m/s
Calculating the numerical value:
vm=2.75m/s
The velocity of the man is 2.75m/s.
Similar Questions
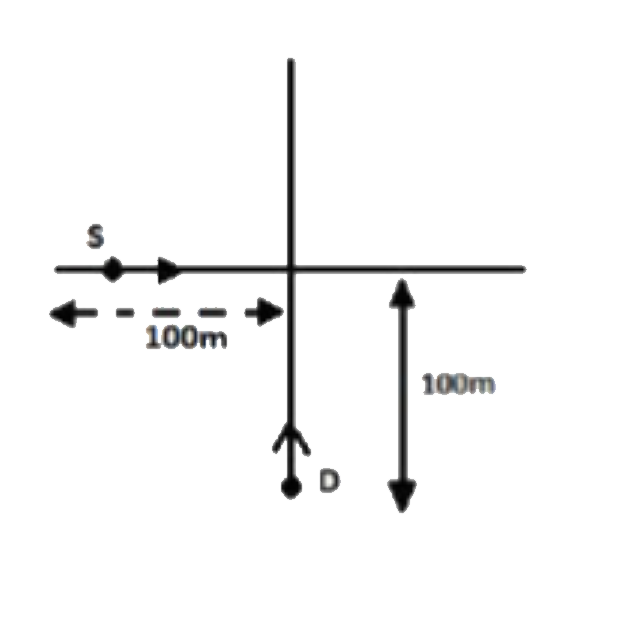
The figure shows the location of a source and detector at time t = 0. The source and detector are moving with velocities vs=5ˆims−1andvD=10ˆjms−1 respectively . The frequency of signals received by the detector at the moment when the source cros the origin is (the frequency of the source is 100 Hz . Velocity bof sound 330ms−1 .)
View SolutionA man standing on a inclined plain observes rain is falling verticaly. When he starts moving down the inclined plain with velocity v=6m/s observes rain hitting him horizontally.

The actual velocity of rain is
View SolutionAn observer travelling with a constant velocity of 16.5ms−1, passes closes to a stationary source of sound and notices that there is a change of frequency of 50 Hz as he passes the source. Calculate the frequency of the source. (Speed of sound in air = 330 ms−1).
View SolutionA man standing on a inclined plain observes rain is falling vertically. When he starts moving down the inclined plain with velocity v=6m/s observes rain hitting him horizontally.

The velocity of rain with respect to the man when he is moving down is
View SolutionA and B are two identical sound sources cachemitting a note of frequency 1000 Hz. A person moves from A to B along the line joining the two sources. How fast should be move to that he has 7 beats per second. velocity of sound 330 m/s ?
View SolutionA man standing between two cliffs produces a sound and hears two successive echoes at intervals of 3 s and 4 s respectively. Calculate the distance between the two cliffs.
The speed of sound in the air is 330ms−1.
View SolutionThe wave length of the sound produced by a source is 0.8m. If the source moves towards the stationary listener at 32ms−1 what is the apparent wave length of sound if the velocity of sound is 320ms−1
View SolutionWhen the observer moves towards the stationary source with velocity, v1, the apparent frequency of emitted note is f1. When the observer moves away from the source with velocity v1, the apparent frequency is f2. If v is the velocity of sound in air and f1f2 = 2,then vv1 = ?
View SolutionA source of sound with natural frequency f0=1800Hz moves uniformly along a straight line separated from a stationary observer by a distance l=250m. The velocity of the source is equal to η=0.80 fraction of the velocity of the sound.
Q. Find the frequency of osund received by the observer at the moment when the source gets closest to him.
View SolutionA source of sound with natural frequency f0=1800Hz moves uniformly along a straight line separated from a stationary observer by a distance l=250m. The velocity of the source is equal to η=0.80 fraction of the velocity of the sound.
Q. Find the frequency of osund received by the observer at the moment when the source gets closest to him.

View Solution
