If the density of a planet is constant, then the curve between value of g on its surface and its radius r will be-
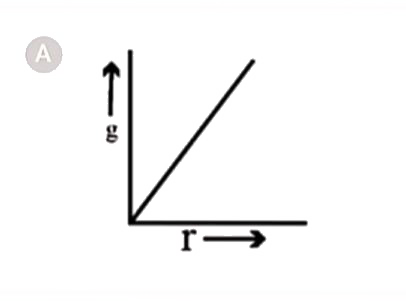
The correct Answer is:A
To solve the problem, we need to establish the relationship between the gravitational acceleration g at the surface of a planet and its radius r, given that the density of the planet is constant.
1. Understanding the relationship: The gravitational acceleration g at the surface of a planet is given by the formula:
g=G⋅MR2
where G is the gravitational constant, M is the mass of the planet, and R is the radius of the planet.
2. Expressing mass in terms of density: Since the density ρ of the planet is constant, we can express the mass M in terms of density and volume:
M=ρ⋅V
The volume V of a sphere is given by:
V=43πR3
Therefore, we can write:
M=ρ⋅43πR3
3. Substituting mass back into the equation for g: Now, substituting the expression for M into the equation for g:
g=G⋅(ρ⋅43πR3)R2
4. Simplifying the equation: This simplifies to:
g=G⋅ρ⋅43πR3R2=43πGρR
Here, we can see that g is directly proportional to R:
g∝R
5. Interpreting the relationship: Since g is directly proportional to R, if we plot g against R, we will get a straight line that passes through the origin.
Conclusion:
Thus, the curve between the value of g on the surface of the planet and its radius r will be a straight line passing through the origin.
Final Answer:
The answer is option A: A straight line passing through the origin.
---
g=G⋅MR2
where G is the gravitational constant, M is the mass of the planet, and R is the radius of the planet.
M=ρ⋅V
The volume V of a sphere is given by:
V=43πR3
Therefore, we can write:
M=ρ⋅43πR3
g=G⋅(ρ⋅43πR3)R2
g=G⋅ρ⋅43πR3R2=43πGρR
Here, we can see that g is directly proportional to R:
g∝R
Thus, the curve between the value of g on the surface of the planet and its radius r will be a straight line passing through the origin.
The answer is option A: A straight line passing through the origin.
Similar Questions
If the earth stops rotating, the apparent value of g on its surface will
View SolutionThe two metallic plates of radius r are placed at a distance d apart and its capacity is C. If a plate of radius r/2 and thickness dof dielectric constant 6 is placed between the plates of the condenser, then its capacity will be
View SolutionA planet has twice the density of earth but the acceleration due to gravity on its surface is exactly the same as that on the surface of earth. Its radius in terms of earth's radius R will be
View SolutionThe density of a newly discovered planet is twice that of earth. The acceleration due to gravity at the surface of the planet is equal to that at the surface of the earth. If the radius of the earth is R, the radius of the planet would be
View SolutionA spherical charged conductor has surface charge density σ.The intensity of electric field and potential on its surface are E and V, now radius is halved keeping surface charge density to be constant. Then the new values will be
View SolutionA planet has a mass of eight time the mass of earth and denisity is also equal to eight times a the average density of the earth. If g be the acceleration due to earth's gravity on its surface, then acceleration due to gravity on planet's surface will be
View SolutionA hypothetical planet in the shape of a sphere is completely made of an incompressible fluid and has a mass M and radius R. If the pressure at the surface of the planet is zero, then the pressure at the centre of the planet is [G = universal constant of gravitation]
View SolutionIf radius of earth is R, then the height h at which the value of g becomes (1/49)th of its value at the surface is
View SolutionAssertion : If the radius of earth is decreased keeping its mass constant, effective value of g may increase or decrease at pole.
Reason : Value of g on the surface of earth is given by g=GmR2.
View SolutionThe acceleration due to gravity on the surface of a planet is one - fourth of the value on Earth. When a brass ball is brought to this planet , its
View Solution
