While measuring the thermal conductivity of liquids the upper part is kept hot and lower cooled so that .
convection many be stopped
radiation may be stopped
heat conduction is easier downwards
it is easier and more convenient to do so
The correct Answer is:A
To solve the question regarding why the upper part of a liquid is kept hot and the lower part is kept cold while measuring thermal conductivity, we can break it down into the following steps:
1. Understanding Thermal Conductivity:
- Thermal conductivity is a measure of a material's ability to conduct heat. In liquids, this property is crucial for understanding how heat transfers through the liquid.
2. Setting Up the Experiment:
- In the experimental setup, the upper part of the liquid is heated while the lower part is cooled. This creates a temperature gradient within the liquid.
3. Analyzing Heat Transfer Mechanisms:
- There are three primary modes of heat transfer: conduction, convection, and radiation. In liquids, convection (the movement of liquid due to temperature differences) can significantly affect the measurement of thermal conductivity.
4. Role of Convection:
- When the upper part of the liquid is heated, the heated liquid becomes less dense and tends to rise, while the cooler, denser liquid at the bottom sinks. This movement can lead to convection currents, which can distort the measurement of thermal conductivity.
5. Avoiding Convection:
- To ensure that the measurement reflects only conduction (the transfer of heat through the material without the movement of the material itself), it is essential to minimize or eliminate convection currents. By keeping the upper part hot and the lower part cold, the experiment is designed to create a stable temperature gradient that promotes conduction.
6. Conclusion:
- Therefore, the reason for keeping the upper part hot and the lower part cold is to avoid convection, ensuring that the heat transfer observed is solely due to conduction.
Final Answer:
The upper part is kept hot and the lower part is kept cold to avoid convection, allowing for an accurate measurement of thermal conductivity.
- Thermal conductivity is a measure of a material's ability to conduct heat. In liquids, this property is crucial for understanding how heat transfers through the liquid.
- In the experimental setup, the upper part of the liquid is heated while the lower part is cooled. This creates a temperature gradient within the liquid.
- There are three primary modes of heat transfer: conduction, convection, and radiation. In liquids, convection (the movement of liquid due to temperature differences) can significantly affect the measurement of thermal conductivity.
- When the upper part of the liquid is heated, the heated liquid becomes less dense and tends to rise, while the cooler, denser liquid at the bottom sinks. This movement can lead to convection currents, which can distort the measurement of thermal conductivity.
- To ensure that the measurement reflects only conduction (the transfer of heat through the material without the movement of the material itself), it is essential to minimize or eliminate convection currents. By keeping the upper part hot and the lower part cold, the experiment is designed to create a stable temperature gradient that promotes conduction.
- Therefore, the reason for keeping the upper part hot and the lower part cold is to avoid convection, ensuring that the heat transfer observed is solely due to conduction.
The upper part is kept hot and the lower part is kept cold to avoid convection, allowing for an accurate measurement of thermal conductivity.
Similar Questions
Two identical plates with thermal conductivities K and 3K are joined together to form a single plate of double thickness. The equivalent thermal conductivity of one composite plate so formed for the flow of heat through its thickness is.
View SolutionA cylindrical block of length 0.4 m and area of cross-section 0.04m2 is placed coaxially on a thin metal disc of mass 0.4 kg and of the same cross - section. The upper face of the cylinder is maintained at a constant temperature of 400 K and the initial temperature of the disc is 300K. if the thermal conductivity of the material of the cylinder is 10watt/m−K and the specific heat of the material of the disc is 600J/kg−K, how long will it take for the temperature of the disc to increase to 350 K? Assume for purpose of calculation the thermal conductivity of the disc to be very high and the system to be thermally insulated except for the upper face of the cylinder.
View SolutionOne end of a uniform brass rod 20 cm long and 10cm2 cross-sectional area is kept at 100∘C. The other end is in perfect thermal contact with another rod of identical cross-section and length 10 cm. The free end of this rod is kept in melting ice and when the steady state has been reached, it is found that 360 g of ice melts per hour. Calculate the thermal conductivity of the rod, given that the thermal conductivity of brass is 0.25cal/scm∘CandL=80cal/g.
View SolutionTwo liquids of densities p1andp2(p2=6p1) are filled up behind a square wall of side 8 m as shown in figure. The liquids have height as shown. The ratio of the forces due to these liquids exerted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing)
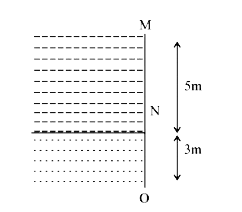
View SolutionTwo liquids of densities ρ1 and ρ2(ρ2=3ρ1) are filled up behind a square wall of side 6 m as shown in figure. Each liquid has a height of 3 m. The ratio of the forces due to these liquids exerted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing)2
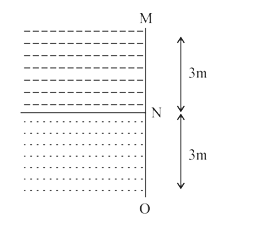
View SolutionTwo liquids of densities ρ1 and ρ2(ρ2=3ρ1) are filled up behind a square wall of side 6 m as shown in figure. Each liquid has a height of 3 m. The ratio of the forces due to these liquids exerted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing)2
View Solution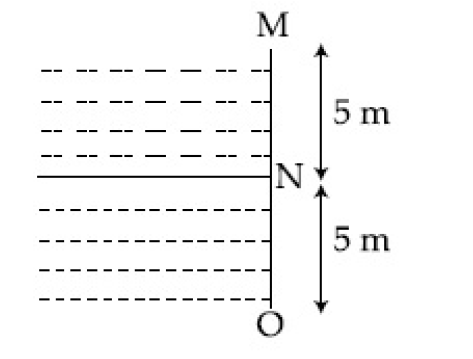
Two liquids of densities ρ1 and ρ2(ρ2=2ρ1) are filled up behind a square wall of side 10 m as shown in the figure. Each liquids has a height of 5m. The ratio of the forces due to these liqyuids exeted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing):View Solution
Equivalent thermal conductivity of figure given below will be given that length of eachh cylinder is l and area of cylinder having thermal conductivity K1 & K3 is A while middle cylinder is having K2.View SolutionCompare the structure of the crystal of diamond graphite with special reference to the reason for diamond being the hardest natural substance while graphite one of the softest. Compare the electrical & thermal conductivity of the two crystalline allotropes of carbon.
View SolutionIn steady state, rate of heat flow through rod of variable thermal conductivity shown in the figure is Q = 6000 W. Area of cross section of rod in m2 varies with x according as A(x)=(1−x), while temperature in Kelvin of rod is T(x)=300(1−4x−x3). Find thermal conductivity (in watt/m-K) of rod at x = 0. (Thermal conductivity depends only on x).
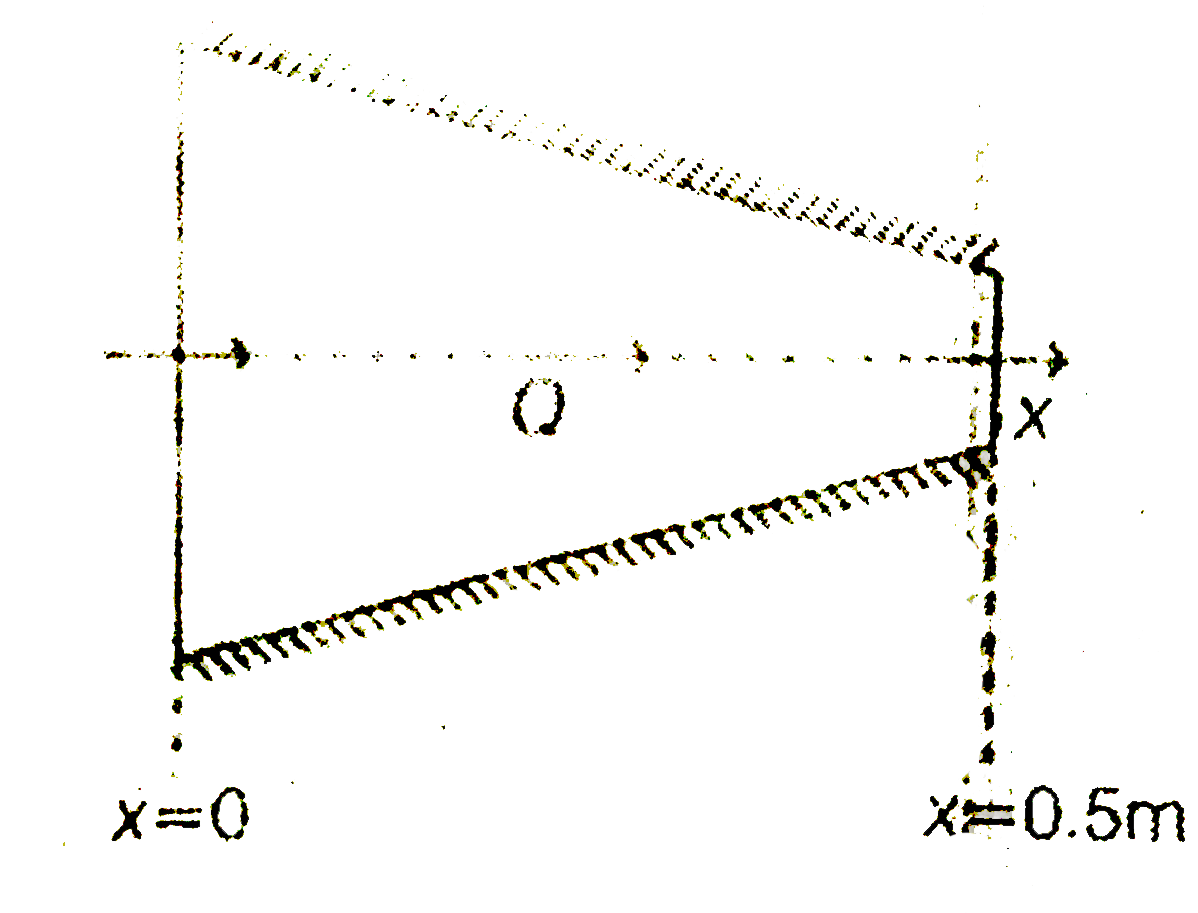
View Solution