Two identical plates with thermal conductivities K and 3K are joined together to form a single plate of double thickness. The equivalent thermal conductivity of one composite plate so formed for the flow of heat through its thickness is.
K
1.5 K
2.5 K
3 K
The correct Answer is:B
To find the equivalent thermal conductivity of the composite plate formed by two identical plates with thermal conductivities K and 3K, we can follow these steps:
Step 1: Understand the Configuration
We have two plates:
- Plate 1 with thermal conductivity K and thickness t.
- Plate 2 with thermal conductivity 3K and thickness t.
When combined, they form a single plate of double thickness 2t.
Step 2: Calculate the Thermal Resistance of Each Plate
The thermal resistance R for a plate is given by the formula:
R=LKA
where L is the thickness of the plate, K is the thermal conductivity, and A is the cross-sectional area.
For Plate 1:
R1=tK⋅A
For Plate 2:
R2=t3K⋅A
Step 3: Calculate the Total Resistance
Since the two plates are in series, the total thermal resistance Rtotal is the sum of the individual resistances:
Rtotal=R1+R2=tK⋅A+t3K⋅A
Step 4: Simplify the Expression
To combine the resistances, we need a common denominator:
Rtotal=3t3K⋅A+t3K⋅A=3t+t3K⋅A=4t3K⋅A
Step 5: Calculate the Equivalent Thermal Conductivity
The equivalent thermal resistance for the composite plate of thickness 2t can be expressed as:
Requivalent=2tKequivalent⋅A
Setting the total resistance equal to the equivalent resistance:
4t3K⋅A=2tKequivalent⋅A
Step 6: Solve for Kequivalent
Cancelling t and A from both sides:
43K=2Kequivalent
Cross-multiplying gives:
4Kequivalent=6K
Thus, we find:
Kequivalent=6K4=3K2=1.5K
Conclusion
The equivalent thermal conductivity of the composite plate is 1.5K.
We have two plates:
- Plate 1 with thermal conductivity K and thickness t.
- Plate 2 with thermal conductivity 3K and thickness t.
The thermal resistance R for a plate is given by the formula:
R=LKA
where L is the thickness of the plate, K is the thermal conductivity, and A is the cross-sectional area.
R1=tK⋅A
R2=t3K⋅A
Since the two plates are in series, the total thermal resistance Rtotal is the sum of the individual resistances:
Rtotal=R1+R2=tK⋅A+t3K⋅A
To combine the resistances, we need a common denominator:
Rtotal=3t3K⋅A+t3K⋅A=3t+t3K⋅A=4t3K⋅A
The equivalent thermal resistance for the composite plate of thickness 2t can be expressed as:
Requivalent=2tKequivalent⋅A
4t3K⋅A=2tKequivalent⋅A
Cancelling t and A from both sides:
43K=2Kequivalent
4Kequivalent=6K
Kequivalent=6K4=3K2=1.5K
The equivalent thermal conductivity of the composite plate is 1.5K.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.41
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.42
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.39
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
Two identical plates of different metals are joined to form a single plate whose thickness is double the thickness of each plate. If the coefficients of conductivity of each plate are 2 and 3 respectively, Then the conductivity of composite plate will be
View Solution
Statement I: Two solid cylindrical rods of identical size and different thermal conductivity K1 and K2 are connected in series. Then the equivalent thermal conductivity of two rods system is less than that value of thermal conductivity of either rod.
Statement II: For two cylindrical rods of identical size and different thermal conductivity K1 and K2 connected in series, the equivalent thermal conductivity K is given by 2K=1K1+1K2
View Solution
Knowledge Check
IF two metallic plates of equal thickness and thermal conductivities K1andK2 are put together face to face anda common plate is constructed, then the equivalent thermal conductivity of this plate will be
AK1K2K1+K2B2K1K2K1+K2C(K21_K22)3/2K1K2D(K21+K22)3/22K1K2Two rods of length l1andl2 and coefficients of thermal conductivities k1andK2 are kept touching each other. Both have the same area of cross-section. The equivalent of thermal conductivity is
AK1+K2BK1l1+K2l2CK1l1+K2l2l1+l2Dl1+l2(l1/K1)+(l2/K2)

Statement I: Two solid cylindrical rods of identical size and different thermal conductivity K1 and K2 are connected in series. Then the equivalent thermal conductivity of two rods system is less than that value of thermal conductivity of either rod.
Statement II: For two cylindrical rods of identical size and different thermal conductivity K1 and K2 connected in series, the equivalent thermal conductivity K is given by 2K=1K1+1K2
View SolutionTwo plates eachb of area A, thickness L1andL2 thermal conductivities K1andK2 respectively are joined to form a single plate of thickness (L1+L2). If the temperatures of the free surfaces are T1andT2. Calculate.
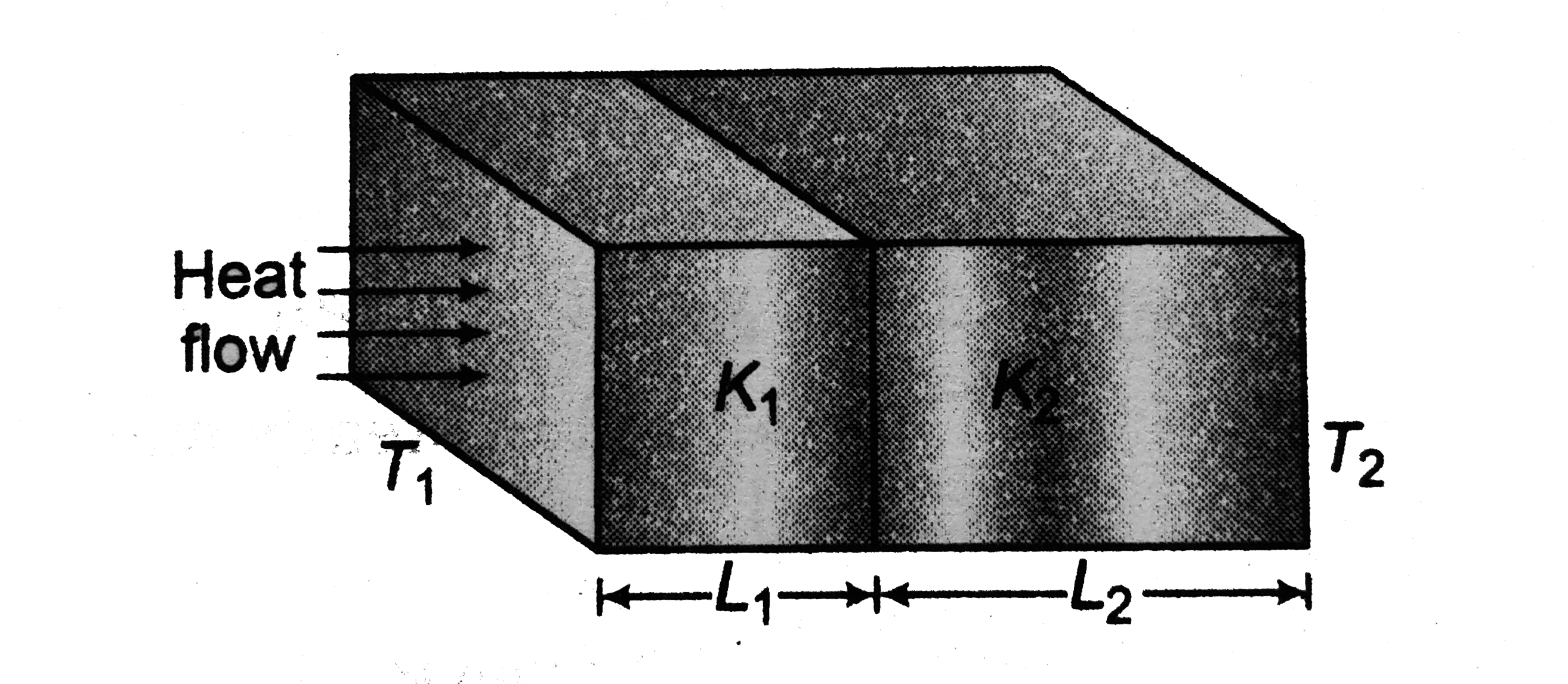
(a) rate of flow of heat
(b) temperature of interface and
(c) equivalent thermal conductivity.
View SolutionThree rods of same dimension are joined in series having thermal conductivity k1 , k2 and k3. The equivalent thermal conductivity is
View SolutionThermal conductivity of inner core of radius r is K and of the outer one of radius 2r is 2K. Find equivalent value of thermal conductivity between its two ends.
View SolutionTwo rods of length d1 and d2 and coefficients of thermal conductivites K1 and K2 are kept touching each other. Both have the same area of cross-section. The equivalent thermal conductivity.
View SolutionConsider a compound slab consisting of two different material having equal thickness and thermal conductivities K and 2K respectively. The equivalent thermal conductivity of the slab is
View Solution
