In an AC circuit the instantaneous values of emf and current are
volt and amp The average power consumed (in watts) is
200
100
50
400
The correct Answer is:B
To solve the problem, we need to calculate the average power consumed in the given AC circuit using the provided instantaneous values of emf (e) and current (i).
1. Identify the Given Values:
- The instantaneous emf is given by:
e=200sin(300t) volts
- The instantaneous current is given by:
i=2sin(300t+π3) amperes
2. Determine the Maximum Values:
- From the equation for emf, the maximum voltage Vmax is:
Vmax=200 volts
- From the equation for current, the maximum current Imax is:
Imax=2 amperes
3. Calculate the RMS Values:
- The RMS (Root Mean Square) value of voltage Vrms is calculated as:
Vrms=Vmax√2=200√2=100√2 volts
- The RMS value of current Irms is calculated as:
Irms=Imax√2=2√2=√2 amperes
4. Determine the Phase Difference:
- The phase difference ϕ between the voltage and current is given by the argument of the sine function in the current equation. Here, it is:
ϕ=π3
5. Calculate the Cosine of the Phase Difference:
- We need to find cos(ϕ):
cos(π3)=12
6. Calculate the Average Power:
- The average power P consumed in the circuit is given by the formula:
P=Vrms⋅Irms⋅cos(ϕ)
- Substituting the values we calculated:
P=(100√2)⋅(√2)⋅(12)
- Simplifying this:
P=100⋅2⋅12=100 watts
Final Answer:
The average power consumed in the circuit is 100 watts.
- The instantaneous emf is given by:
e=200sin(300t) volts
- The instantaneous current is given by:
i=2sin(300t+π3) amperes
- From the equation for emf, the maximum voltage Vmax is:
Vmax=200 volts
- From the equation for current, the maximum current Imax is:
Imax=2 amperes
- The RMS (Root Mean Square) value of voltage Vrms is calculated as:
Vrms=Vmax√2=200√2=100√2 volts
- The RMS value of current Irms is calculated as:
Irms=Imax√2=2√2=√2 amperes
- The phase difference ϕ between the voltage and current is given by the argument of the sine function in the current equation. Here, it is:
ϕ=π3
- We need to find cos(ϕ):
cos(π3)=12
- The average power P consumed in the circuit is given by the formula:
P=Vrms⋅Irms⋅cos(ϕ)
- Substituting the values we calculated:
P=(100√2)⋅(√2)⋅(12)
- Simplifying this:
P=100⋅2⋅12=100 watts
The average power consumed in the circuit is 100 watts.
Similar Questions
In an AC circuit, the instantaneous values of e.m.f and current are e=200sin314t volt and i=sin(314t+π3) ampere. The average power consumed in watt is
View SolutionIn an AC circuit, the instantaneous values of e.m.f and current are e=200sin314t volt and i=sin(314t+π3) ampere. The average power consumed in watt is
View SolutionIn an a.c. circuit, the instantaneous e.m.f. and current are given by e = 100 sin 30 t
i=20sin(30t−π4) In one cycle of a.c., the average power consumed by the circuit and the wattless current are, respectively :
View SolutionIn an a.c. circuit, the instantaneous e.m.f. and current are given by e = 100 sin 30 t
i=20sin(30t−π4) In one cycle of a.c., the average power consumed by the circuit and the wattless current are, respectively :
View SolutionIn an AC circuit, the applied potential difference and the current flowing are given by
V=20sin100t volt ,I=5sin(100t−π2) amp
The power consumption is equal to
View SolutionIn a circuit, the instantaneous values of alternating current and voltages in a circuit is given by
I=1√2sin(100πt)A and
E=1√2sin(100πt+π3)V.
The average power in watts consumed in the circui is
View SolutionIn an ac circuit the instantaneous values of current and applied voltage are respectively i=2(Amp)sin(250πs−1tandε=(10V)sin[(250πs−1)t+π3, Find the instantaneous power drawn from the source at t=23ms and its average value.
View SolutionIn an ac circuit the instantaneous values of current and applied voltage are respectively i=2(Amp)sin(250πs−1tandε=(10V)sin[(250πs−1)t+π3, Find the instantaneous power drawn from the source at t=23ms and its average value.
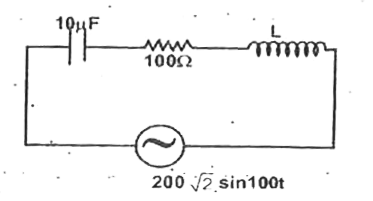
View SolutionA 10 muF capacitor, an inductor and a resistor of 100Omega are connected to an alternating source of emf 200sqrt2 sin 100t as shown in the figure. What is the r.m.s current in the circuit and the value of the inductance if the current and the source voltage attain their maxima simultaneously? What is the average power consumed in this case
View SolutionIn an a.c. circuit V and I are given by V=50 sin50t volt and I = 100 sin(50t+π/3) mA. The power dissipated in the circuit
View Solution
