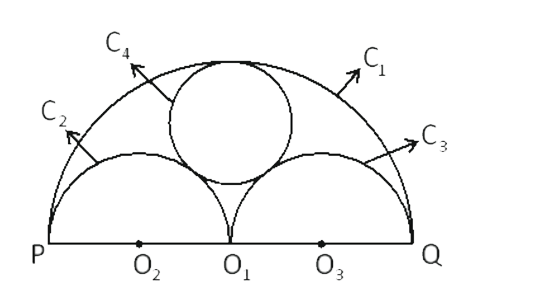
In the figure PQ PO1andO1Q are the diameters of semicircles C1,C2andC3 with centres at O1,O2andO3 respectively circle 4 touches the semicircle C1C2andC3 . If PQ = 24 units and the area of the circle 4 is A sq. units , then the value of 8πA is equal ot (here, PO1=O1Q)
The correct Answer is:0.5
Step by step video & image solution for In the figure PQ PO_1 and O_1Q are the diameters of semicircles C_1,C_2 and C_3 with centres at O_1 ,O_2 and O_3 respectively circle 4 touches the semicircle C_1 C_2 and C_3 . If PQ = 24 units and the area of the circle 4 is A sq. units , then the value of (8pi)/(A) is equal ot (here, PO_1 = O_1Q) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
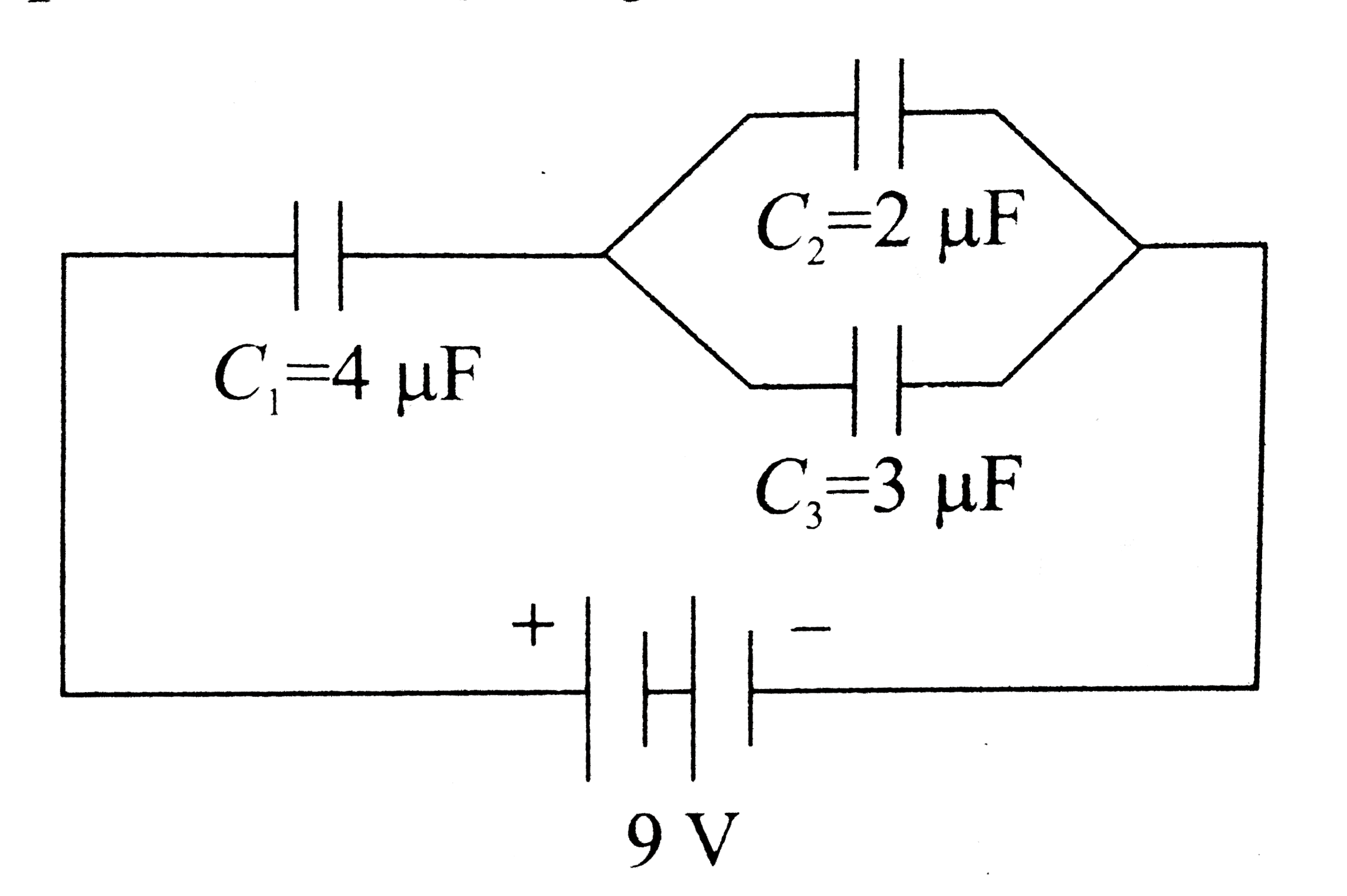
In figure, the charges on C1,C2, and C3, are Q1,Q2, and Q3, respectively.
 .
.
View SolutionIn Figure, O is the centre of the circle. Find ∠BAC
View SolutionLet circles C1,C2andC3 with centres O1,O2andO3 respectively touch each other externally, where
O1=(−36,7),O2=(20,7)andO3=(0,−8). The coordinates of the centre of a circle passing through the points of contact of circles C1,C2andC2,C3andC3,C1 are
View SolutionC1andC2 are fixed circles of radii r1andr2 touches each other externally. Circle 'C' touches both Circles C1andC2 extemelly. If r1r2=32 then the eccentricity of the locus of centre of circles C is
View SolutionIf C1 (1, 3) and C2(4, 3)are the centres of two circles whose radical axis is y-axis. If the radius of the 1st circle is 2√2 units, then the radius of the second circle is
View SolutionIn the figure, PO⊥QO. The tangents to the circle with centre O at P and Q intersect at a point T. Prove that PQ and OT are right bisectors of each other.

View SolutionFind the area of the shaded region in Figure, if PQ=24cm,PR=7cm and O is the centre of the circle.
View SolutionTwo circles with centres O and O' of radii 3 cm and 4 cm, respectively intersect at two points P and Q such that OP and O'P are tangents to the two circles. Find the length of common chord PQ.
View SolutionIf Figure, AOC is a diameter of the circle and arc AXB=12 arc BYC. Find ∠BOC
View SolutionFind the area of the shaded region in Figure, if AC=24 cm , BC=10 cm and O is the centre of the circle. (Use π=3.14)
View Solution
