If Figure, AOC is a diameter of the circle and arc AXB=12 arc BYC. Find ∠BOC
To solve the problem step by step, we will follow the reasoning provided in the video transcript.
1. Identify the Given Information:
- We have a circle with diameter AOC.
- It is given that arc AXY is half of arc BYC.
2. Understand the Relationship of Angles:
- Since AOC is a diameter, angle AOC is a straight angle, which measures 180∘.
3. Set Up the Ratio of the Arcs:
- Given that arc AXY is half of arc BYC, we can express this relationship as:
arc AXY:arc BYC=1:2
4. Define Angles Based on the Arcs:
- Let angle BOA=x and angle BOC=2x.
- This is because the angles subtended by the arcs at the center of the circle are proportional to the lengths of the arcs.
5. Use the Straight Angle Property:
- The sum of angles AOB and BOC must equal AOC:
angle AOB+angle BOC=angle AOC
- Therefore, we can write:
x+2x=180∘
6. Solve for x:
- Combine like terms:
3x=180∘
- Divide both sides by 3:
x=180∘3=60∘
7. Find Angle BOC:
- Since BOC=2x:
BOC=2×60∘=120∘
8. Conclusion:
- The measure of angle BOC is 120∘.
Final Answer:
∠BOC=120∘
- We have a circle with diameter AOC.
- It is given that arc AXY is half of arc BYC.
- Since AOC is a diameter, angle AOC is a straight angle, which measures 180∘.
- Given that arc AXY is half of arc BYC, we can express this relationship as:
arc AXY:arc BYC=1:2
- Let angle BOA=x and angle BOC=2x.
- This is because the angles subtended by the arcs at the center of the circle are proportional to the lengths of the arcs.
- The sum of angles AOB and BOC must equal AOC:
angle AOB+angle BOC=angle AOC
- Therefore, we can write:
x+2x=180∘
- Combine like terms:
3x=180∘
- Divide both sides by 3:
x=180∘3=60∘
- Since BOC=2x:
BOC=2×60∘=120∘
- The measure of angle BOC is 120∘.
∠BOC=120∘
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the figure, O is the center of the circle. What is average of the numbers a, b, c and d?
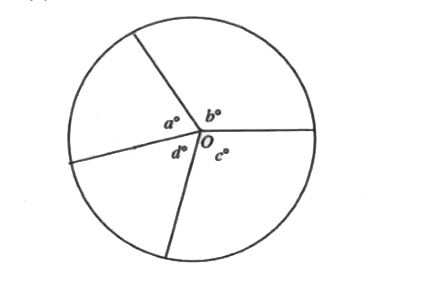 A45B60C90D180
A45B60C90D180
In Figure, O is the centre of the circle and the measure of arc ABC is 1000. Determine ∠ADC and ∠ABC
View SolutionIn Figure, it is given that O is the centre of the circle and ∠AOC=150∘. Find ∠ABC
View SolutionIn Figure, O is the centre of the circle and BCD is tangent to it at C. Prove that ∠BAC+∠ACD=900 .
View SolutionIn Figure, AB and CD are two diameters of a circle perpendicular to each other and OD is the diameter of the smaller circle. If OA=7 cm , find the area of the shaded region.
View SolutionIn the given figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC.
if ∠AOB=100∘ find ∠BOC
View SolutionIn Figure, AB=CB and O is the centre of the circle. Prove that BO bisects ∠ABC.
View SolutionAB and CD are diameters of a circle with centre O. If ∠OBD=500, find ∠AOC
View Solution
