The level of water in a tank is 5 m high . A hole of the area 10cm2 is made in the bottom of the tank . The rate of leakage of water from the hole is
10−2m3s−1
102m3s−1
10m3s−1
10−4m−3s−1
The correct Answer is:A
To solve the problem of finding the rate of leakage of water from a hole in a tank, we can follow these steps:
Step 1: Understand the given information
We have a tank filled with water to a height of h=5m. There is a hole at the bottom of the tank with an area A=10cm2.
Step 2: Convert the area into appropriate units
Since we need to work with SI units, we convert the area from cm2 to m2:
A=10cm2=10×10−4m2=10−3m2
Step 3: Use Torricelli's Law to find the velocity of water
According to Torricelli's Law, the velocity v of the water flowing out of the hole can be calculated using the formula:
v=√2gh
where g is the acceleration due to gravity (approximately 10m/s2) and h is the height of the water column.
Substituting the values:
v=√2×10m/s2×5m=√100=10m/s
Step 4: Calculate the flow rate (Q)
The flow rate Q (the volume of water leaking per second) can be calculated using the formula:
Q=A×v
Substituting the values we have:
Q=10−3m2×10m/s=10−2m3/s
Step 5: Conclusion
The rate of leakage of water from the hole is:
Q=10−2m3/s
Final Answer
The rate of leakage of water from the hole is 10−2m3/s.
---
We have a tank filled with water to a height of h=5m. There is a hole at the bottom of the tank with an area A=10cm2.
Since we need to work with SI units, we convert the area from cm2 to m2:
A=10cm2=10×10−4m2=10−3m2
According to Torricelli's Law, the velocity v of the water flowing out of the hole can be calculated using the formula:
v=√2gh
where g is the acceleration due to gravity (approximately 10m/s2) and h is the height of the water column.
v=√2×10m/s2×5m=√100=10m/s
The flow rate Q (the volume of water leaking per second) can be calculated using the formula:
Q=A×v
Substituting the values we have:
Q=10−3m2×10m/s=10−2m3/s
The rate of leakage of water from the hole is:
Q=10−2m3/s
The rate of leakage of water from the hole is 10−2m3/s.
Similar Questions
The level of water in a tank is 5 m high. A hole of area of cross section 1 cm2 is made at the bottom of the tank. The rate of leakage of water for the hole in m3s−1 is (g=10ms−2)
View SolutionWater is being poured in a vessel at a constant rate αm2/s. There is a small hole of area a at the bottom of the tank. The maximum level of water in the vessel is proportional to
View Solution
Knowledge Check
The height of the water in a tank is H. The range of the liquid emerging out from a hole in the wall of the tank at a depth 3H4 from the upper surface of water, will be
AHBH2C3H2D√3H2
The flat bottom of cylinder tank is silvered and water (μ=43) is filled in the tank upto a height h. A small bird is hovering at a height 3h from the bottom of the tank. When a small hole is opened near the bottom of the tank, the water level falls at the rate of 1 cm/s. The bird will perceive that his velocity of image is 1/x cm/sec (in downward directions) where x is
View SolutionWater flows into a large tank with flat bottom at the rate of 10−4m3s−1. Water is also leaking out of a hole of area 1 cm2 at its bottom. If the height of the water in the tank remains steady, then this height is :
View SolutionA rectangular tank is placed on a horizontal ground and is filled with water to a height H above the base. A small hole is made on one vertical side at a depth D below the level of the water in the tank. The distance x from the bottom of the tank at which the water jet from the tank will hit the ground is
View SolutionA tank of large base area is filled with water up to a height of 5m. A hole of 2cm2 cross section in the bottom allows the water to drain out in continuous streams. For this situation, mark out the correct statement(s) (take ρwater=1000kg/m3,g=10ms−12)
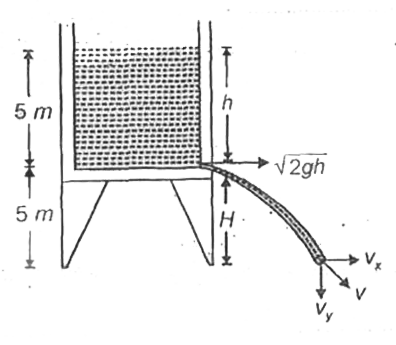
View SolutionA water tank is placed on a platform of height 5 m high and there is an orifice near the bottom in the wall of tank at 5 m below the level of water. Find the speed with which water will hit the ground [Take g = 10 m/s2 ]
View SolutionSuppose that water is emptied from a spherical tank of radius 10 cm. If the depth of the water in the tank is 4 cm and is decreasing at the rate of 2 cm/sec, then the radius of the top surface of water is decreasing at the rate of
View SolutionWater is filled in a container upto height 3m. A small hole of area 'a' is punched in the wall of the container at a height 52.5 cm from the bottom. The cross sectional area of the container is A. If a/A=0.1 then v2 is (where v is the velocity of water coming out of the hole)
View Solution
