A rectangular tank is placed on a horizontal ground and is filled with water to a height H above the base. A small hole is made on one vertical side at a depth D below the level of the water in the tank. The distance x from the bottom of the tank at which the water jet from the tank will hit the ground is
2[D(H−D)]]1/2
2(gD)12
2[D(H+D)]]1/2
12(DH)12
The correct Answer is:A
To solve the problem of determining the distance x from the bottom of the tank at which the water jet will hit the ground, we can follow these steps:
Step 1: Determine the height of the water above the hole
The height of the water above the hole is given by:
habove=H−D
where H is the total height of the water in the tank and D is the depth of the hole below the water level.
Step 2: Calculate the efflux speed of the water
Using Torricelli's theorem, the speed v of the water exiting the hole can be calculated using the formula:
v=√2ghabove=√2g(H−D)
where g is the acceleration due to gravity.
Step 3: Determine the time of flight of the water jet
The time t it takes for the water to fall from the height H−D to the ground can be calculated using the second equation of motion:
H−D=12gt2
Rearranging this gives:
t2=2(H−D)g
Taking the square root, we find:
t=√2(H−D)g
Step 4: Calculate the horizontal distance traveled by the water jet
The horizontal distance x that the water travels while falling can be calculated using the formula:
x=v⋅t
Substituting the expressions for v and t:
x=√2g(H−D)⋅√2(H−D)g
Simplifying this expression:
x=√2g(H−D)⋅√2(H−D)g=2(H−D)
Final Result
Thus, the distance x from the bottom of the tank at which the water jet will hit the ground is:
x=2√D(H−D)
The height of the water above the hole is given by:
habove=H−D
where H is the total height of the water in the tank and D is the depth of the hole below the water level.
Using Torricelli's theorem, the speed v of the water exiting the hole can be calculated using the formula:
v=√2ghabove=√2g(H−D)
where g is the acceleration due to gravity.
The time t it takes for the water to fall from the height H−D to the ground can be calculated using the second equation of motion:
H−D=12gt2
Rearranging this gives:
t2=2(H−D)g
Taking the square root, we find:
t=√2(H−D)g
The horizontal distance x that the water travels while falling can be calculated using the formula:
x=v⋅t
Substituting the expressions for v and t:
x=√2g(H−D)⋅√2(H−D)g
Simplifying this expression:
x=√2g(H−D)⋅√2(H−D)g=2(H−D)
Thus, the distance x from the bottom of the tank at which the water jet will hit the ground is:
x=2√D(H−D)
Similar Questions
A tank if filled with water upto height H. When a hole is made at a distance h below the level of water. What will be the horizontal range of water jet ?
View SolutionA large tank filled with water to a height h is to be emptied through a small hole at the bottom. The ratio of times taken for the level of water to fall from h to h2 and from h2 to zero is
View SolutionThe level of water in a tank is 5 m high . A hole of the area 10cm2 is made in the bottom of the tank . The rate of leakage of water from the hole is
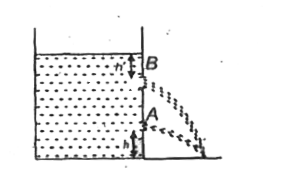
View SolutionA tank is filled with water and two holes A and B are made in it.- For getting same range, ratio of h' and h is
View SolutionThe flat bottom of cylinder tank is silvered and water (μ=43) is filled in the tank upto a height h. A small bird is hovering at a height 3h from the bottom of the tank. When a small hole is opened near the bottom of the tank, the water level falls at the rate of 1 cm/s. The bird will perceive that his velocity of image is 1/x cm/sec (in downward directions) where x is
View SolutionA rectangular water tank of base 11m×6m contains water upto a height of 5 m. If the water in the tank is transferred to a cylindrical tank of radius 3.5 m, find the height of the water level in the tank.
View SolutionP is the atmospheric pressure and the pressure at the bottom of a tank of water is 3P. If the water is drawn out to lower the level of water by one fifth, then the pressure at the bottom of the tank will be
View SolutionA large open tank is filled with water upto a height H. A small hole is made at the base of the tank. It takes T1 time to decrease the height of water to H/n(ngt1) and it takes T2 time to take out the remaining water. If T1=T2, then the value of n is
View Solution_E01_218_Q01.png)
A large tank is filled with two liquids of specific gravities 2σ and σ. Two holes are made on the wall of the tank as shown. Find the ratio of the distance from O of the points on the ground where the jets from holes A & B strike.
View SolutionA tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
View Solution
