Calculate the number of hours of service that can be derived at 1 atm, 300 K from an acetylene lamp containing 640 g calcium carbide. Given that the lamp requires 50 L acetylene gas at 1 atm 300 K for one hour. [Take 0.0821×300=25]
The correct Answer is:5
To solve the problem of calculating the number of hours of service that can be derived from an acetylene lamp containing 640 g of calcium carbide, we can follow these steps:
Step 1: Write the Reaction
The reaction for the decomposition of calcium carbide (CaC₂) to produce acetylene (C₂H₂) is as follows:
CaC2+H2O→C2H2+Ca(OH)2
This shows that one mole of calcium carbide produces one mole of acetylene.
Step 2: Calculate the Molar Mass of Calcium Carbide
To find the number of moles of calcium carbide, we first need to calculate its molar mass.
- Calcium (Ca) = 40 g/mol
- Carbon (C) = 12 g/mol (and there are 2 carbon atoms in calcium carbide)
Thus, the molar mass of calcium carbide (CaC₂) is:
Molar mass of CaC2=40+(2×12)=40+24=64 g/mol
Step 3: Calculate the Number of Moles of Calcium Carbide
Using the formula:
Number of moles=Given massMolar mass
Given mass of calcium carbide = 640 g, we can calculate:
Number of moles=640 g64 g/mol=10 moles
Step 4: Calculate the Volume of Acetylene Produced
Using the ideal gas equation PV=nRT, we can rearrange it to find the volume (V):
V=nRTP
Where:
- n=10 moles
- R=0.0821 L atm/(K mol)
- T=300 K
- P=1 atm
Substituting the values:
V=10×0.0821×3001
Calculating 0.0821×300=24.63:
V=10×24.63=246.3 L
Step 5: Determine the Hours of Service
The lamp requires 50 L of acetylene gas for one hour. To find the total hours of service, we can use a unitary method:
Hours of service=Total volume of acetyleneVolume required per hour
Hours of service=246.3 L50 L/hour=4.926 hours
Step 6: Round to the Nearest Whole Number
Rounding 4.926 to the nearest whole number gives us approximately 5 hours.
Final Answer
The number of hours of service that can be derived from the acetylene lamp containing 640 g of calcium carbide is approximately 5 hours.
---
The reaction for the decomposition of calcium carbide (CaC₂) to produce acetylene (C₂H₂) is as follows:
CaC2+H2O→C2H2+Ca(OH)2
This shows that one mole of calcium carbide produces one mole of acetylene.
To find the number of moles of calcium carbide, we first need to calculate its molar mass.
- Calcium (Ca) = 40 g/mol
- Carbon (C) = 12 g/mol (and there are 2 carbon atoms in calcium carbide)
Molar mass of CaC2=40+(2×12)=40+24=64 g/mol
Using the formula:
Number of moles=Given massMolar mass
Given mass of calcium carbide = 640 g, we can calculate:
Number of moles=640 g64 g/mol=10 moles
Using the ideal gas equation PV=nRT, we can rearrange it to find the volume (V):
V=nRTP
Where:
- n=10 moles
- R=0.0821 L atm/(K mol)
- T=300 K
- P=1 atm
V=10×0.0821×3001
Calculating 0.0821×300=24.63:
V=10×24.63=246.3 L
The lamp requires 50 L of acetylene gas for one hour. To find the total hours of service, we can use a unitary method:
Hours of service=Total volume of acetyleneVolume required per hour
Hours of service=246.3 L50 L/hour=4.926 hours
Rounding 4.926 to the nearest whole number gives us approximately 5 hours.
The number of hours of service that can be derived from the acetylene lamp containing 640 g of calcium carbide is approximately 5 hours.
Similar Questions
Knowledge Check
Under isothermal condition, a gas at 300 K expands from 0.1 L to 0.25 L against a constant external pressure of 2 bar. The work done by the gas is (Given that 1 L bar = 100 J)
A25 JB30 JC-30 JD5 kJ.
Calculate the work done when 1 mole of a gas expands reversibly and isothermally from 5 atm to 1 atm at 300 K. [Value of log 5 = 0.6989].
View SolutionCalculate the compressibility factor for SO2, if 1 mole of it occupies 0.35 litre at 300 K and 50 atm. Also comment on the result.
View SolutionCalculate the amount of heat required to raise the temperature of 13.5 g aluminium from 300 K to 400 K, if specific heat of aluminium is 0.9 J (.∘k)−1g−1.
View SolutionAt 300 K, 4 gm calcium is dissolved in hydrochloric acid in an open vessel at the atmoshphere pressure 0.821 atm. Calculate work done by the system.
View SolutionCalculate the values of σ, l (mean free path),Z1 and Z11 for oxygen at 300K at a pressure of 1atm. Given b=3.183×10−2dm3mol−1.
View SolutionCalculate the amount of heat required to raise the temperature of 13.9g aluminium from 300K to 400K, if specific heat of aluminium is 0.9J∘C−1g−1.
View SolutionThe figure given below shows three glass chambers that are connected by valves of negligible volume. At the outset of an experiment, the valves are closed and the chambers contain the gases as detailed in the diagram. All the chambers are at the temperature of 300K and external pressure of 1.0atm.
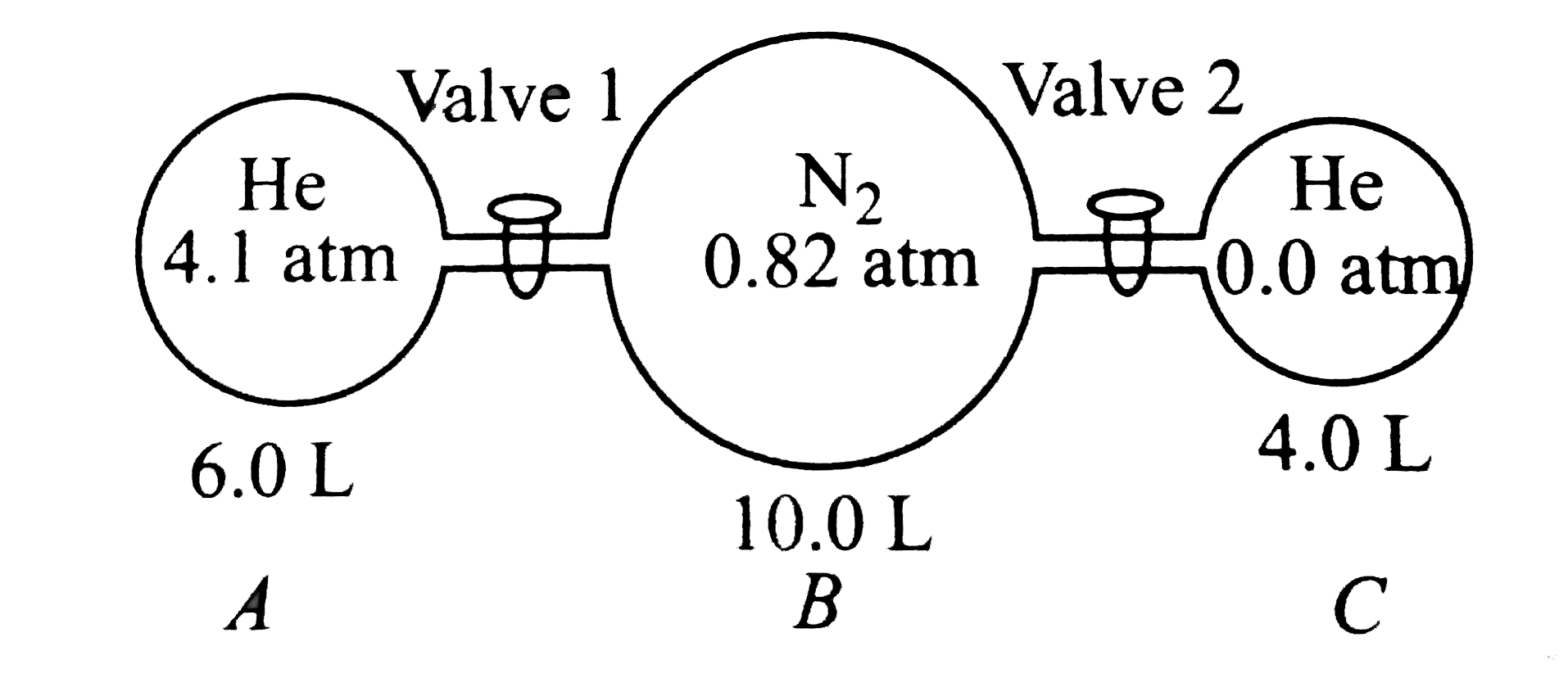
Which of the following represents the total kinetic energy of all the gas molecules after both valves are opened?
(R=0.082atmLK−1mol−1=8.314JK_1mol−1)
View Solution
