An ammeter, voltmeter and a resistor are connected in series to a cell and the readings are noted as I and V. If another resistor R is connected in parallel with a voltmeter, then
I and V increase
I increases
I and V will remain same
I decreases
The correct Answer is:B
To solve the problem step by step, we will analyze the situation where an ammeter, voltmeter, and a resistor are connected in series to a cell, and then we will consider the effect of connecting another resistor in parallel with the voltmeter.
Step 1: Understand the Initial Setup
We have an ammeter (A), a voltmeter (V), and a resistor (R1) connected in series to a cell. The readings of the ammeter and voltmeter are noted as I (current) and V (voltage across the voltmeter), respectively.
Step 2: Analyze the Circuit
In a series circuit, the total resistance (R_total) is the sum of the individual resistances. Therefore, the total resistance in the circuit is:
Rtotal=R1+RV
where RV is the internal resistance of the voltmeter.
Step 3: Apply Ohm's Law
According to Ohm's Law, the current (I) flowing through the circuit can be expressed as:
I=VcellRtotal=VcellR1+RV
Step 4: Introduce the New Resistor
Now, when another resistor (R2) is connected in parallel with the voltmeter, the effective resistance of the voltmeter and the new resistor in parallel (R2) is given by:
1Reff=1RV+1R2
This means that the effective resistance (R_eff) of the voltmeter and the resistor R2 will be less than the resistance of the voltmeter alone.
Step 5: Calculate the New Total Resistance
The new total resistance in the circuit becomes:
Rnew=R1+Reff
Since Reff<RV, it follows that:
Rnew<R1+RV
Step 6: Determine the Effect on Current
Since the total resistance in the circuit has decreased, the current (I) flowing through the circuit will increase. According to Ohm's Law:
Inew=VcellRnew
Since Rnew<Rtotal, we have:
Inew>I
Conclusion
Thus, when the resistor R2 is connected in parallel with the voltmeter, the current I increases. The voltage across the voltmeter remains the same because it is still connected across the same cell.
Final Answer
When another resistor R is connected in parallel with the voltmeter, the current (I) in the circuit increases.
---
We have an ammeter (A), a voltmeter (V), and a resistor (R1) connected in series to a cell. The readings of the ammeter and voltmeter are noted as I (current) and V (voltage across the voltmeter), respectively.
In a series circuit, the total resistance (R_total) is the sum of the individual resistances. Therefore, the total resistance in the circuit is:
Rtotal=R1+RV
where RV is the internal resistance of the voltmeter.
According to Ohm's Law, the current (I) flowing through the circuit can be expressed as:
I=VcellRtotal=VcellR1+RV
Now, when another resistor (R2) is connected in parallel with the voltmeter, the effective resistance of the voltmeter and the new resistor in parallel (R2) is given by:
1Reff=1RV+1R2
This means that the effective resistance (R_eff) of the voltmeter and the resistor R2 will be less than the resistance of the voltmeter alone.
The new total resistance in the circuit becomes:
Rnew=R1+Reff
Since Reff<RV, it follows that:
Rnew<R1+RV
Since the total resistance in the circuit has decreased, the current (I) flowing through the circuit will increase. According to Ohm's Law:
Inew=VcellRnew
Since Rnew<Rtotal, we have:
Inew>I
Thus, when the resistor R2 is connected in parallel with the voltmeter, the current I increases. The voltage across the voltmeter remains the same because it is still connected across the same cell.
When another resistor R is connected in parallel with the voltmeter, the current (I) in the circuit increases.
Similar Questions
An ammeter and a voltmeter are joined in series to a cell. Their readings are A and V respectively. If a resistance is now joining parallel with the voltmeter. Then
View SolutionAn ammeter and a voltmeter are joined in sereis to a cell. Their readings are A and V respectively. If a resistance is now joinding parallel with the voltmeter. Then
View SolutionAn ammeter and a voltmeter are connected in series to a battery with an emf ξ=6.0V. When a certain resistance is connected in parallel with the voltmeter, the readings of the latter decreases η=2.0 times, whereas the readings of the ammeter increase the same number of times, Find the voltmeter readings after the connection of the resistance.
View SolutionAn ammeter and a voltmeter of resistance R connected in seires to an electric cell of negligible internal resistance. Their readings are A and V respecitvely. If another resistance R is connected in parallel with the voltmeter
View SolutionAn ammeter and a voltmeter of resistance R connected in seires to an electric cell of negligible internal resistance. Their readings are A and V respecitvely. If another resistance R is connected in parallel with the voltmeter
View SolutionA voltmeter of resistance R1 and an ammeter of resistance R2 are connected in series across a battery oif negligible internal resistance. When as resistance R is connected in parallel to voltmeter reading of ammeter increases three times white that of voltmeter reduces to one third. Find the ratio of R1 and R2.
View SolutionAn ammeter and a voltmeter are connected in series to an ideal battery of emf E. If the ammeter reads I and voltmeter reading V, then
View SolutionA battery of electro motive force E is connected in series with a resistance R and a voltmeter. An ammeter is connected in parallel with the battery.
View SolutionA voltmeter and an ammeter are connected in series to an ideal cell of emfE. The voltmeter reading is V, and the ammeter readings is I. Then
(i) V<E (ii) the voltmeter resistance is V/I
(iii) the potential difference across the ammeter is E−V
(iv) Voltmeter resistance + ammeter resistance = E//I
Correct statements are
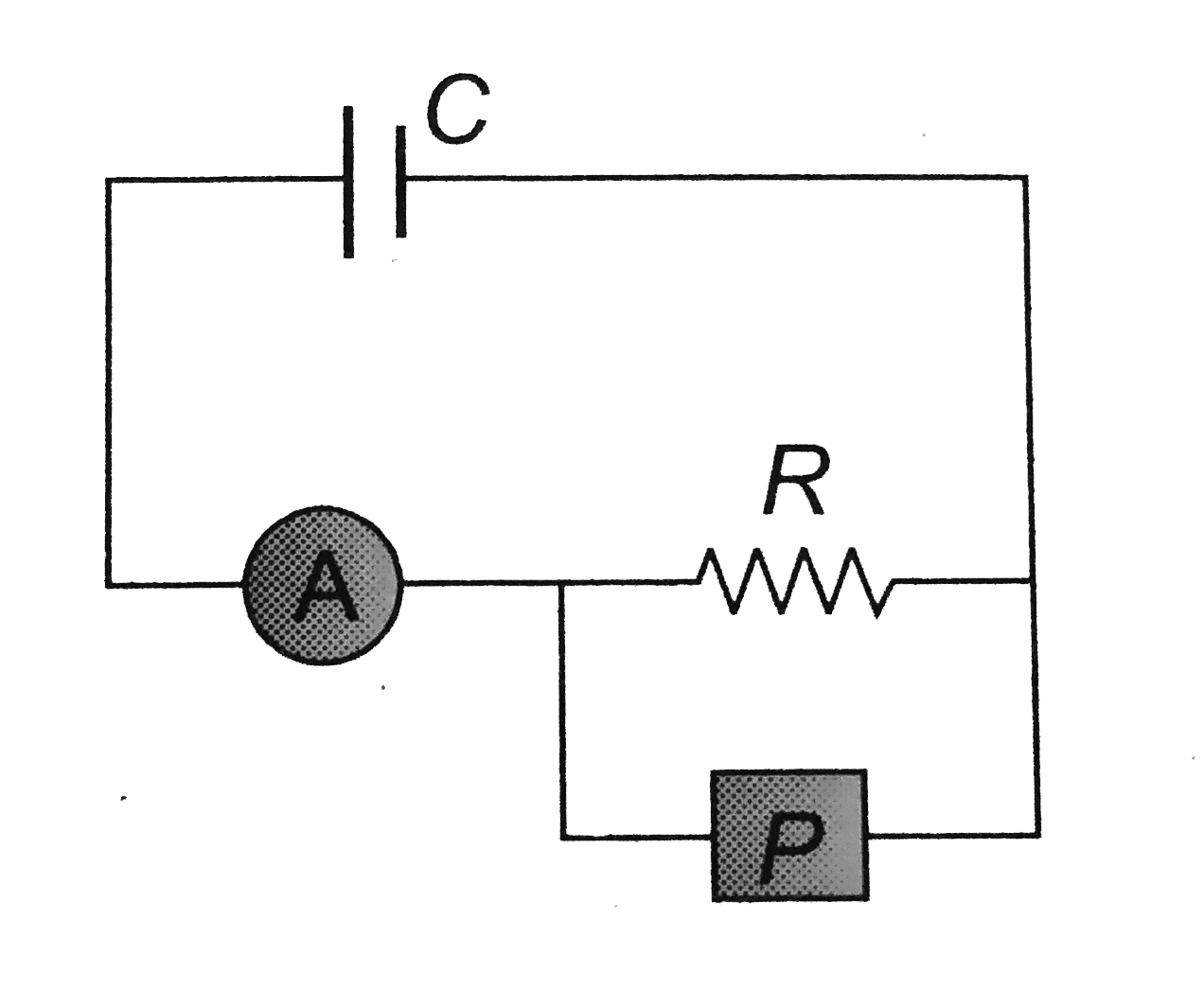
View SolutionAn ammeter A of finite resistance, and a resistor R are joined in series to an ideal cell C. A potentiometer P is joined in parallel to R. The ammeter reading is I0 and the potentiometer reading is V0. P is now replaced by a voltmeter of finite resistance. The ammeter reading now is I and the voltmeter reading is V.
View Solution
