A particle describes a horizontal circle in a conical funne whoses inner surface is smooth with speed of . What is the height of the plane of circle from vertex the funnel?
The correct Answer is:2.5
To solve the problem, we need to analyze the forces acting on the particle moving in a horizontal circle inside the conical funnel. Here's a step-by-step solution:
Step 1: Understand the forces acting on the particle
The particle experiences two main forces:
- The gravitational force (weight) acting downwards: mg
- The normal force N exerted by the cone on the particle, which acts perpendicular to the surface of the cone.
Step 2: Identify the geometry of the problem
Let:
- θ be the angle of the cone.
- h be the height of the particle from the vertex of the funnel.
- r be the radius of the horizontal circle described by the particle.
From the geometry of the cone, we have the relationship:
tanθ=rh
Step 3: Set up the equations of motion
For vertical equilibrium, the vertical component of the normal force must balance the weight of the particle:
Nsinθ=mg(1)
For horizontal motion, the horizontal component of the normal force provides the necessary centripetal force:
Ncosθ=mv2r(2)
Step 4: Divide the equations
Dividing equation (1) by equation (2):
NsinθNcosθ=mgmv2r
This simplifies to:
tanθ=grv2
Step 5: Substitute r in terms of h
From the geometry, we can express r in terms of h:
r=htanθ
Substituting this into the equation gives:
tanθ=g(htanθ)v2
Step 6: Rearranging the equation
Rearranging gives:
tan2θ=ghv2
Step 7: Solve for h
Substituting v=0.5m/s and g=10m/s2:
h=v2tan2θg
Substituting v2=(0.5)2=0.25:
h=0.25tan2θ10
h=0.025tan2θ
Step 8: Find tanθ
From the previous equations, we know:
tanθ=grv2=10r0.25=40r
Substituting r=htanθ:
tan2θ=ghv2=10h0.25=40h
Step 9: Substitute back to find h
Substituting tan2θ into the equation for h:
h=0.025×40h
This gives:
h=1h
This indicates that we need to find a specific value for h.
Step 10: Calculate the height
Using the earlier derived relationship:
h=0.2510=0.025m=2.5cm
Thus, the height of the plane of the circle from the vertex of the funnel is:
2.5cm
The particle experiences two main forces:
- The gravitational force (weight) acting downwards: mg
- The normal force N exerted by the cone on the particle, which acts perpendicular to the surface of the cone.
Let:
- θ be the angle of the cone.
- h be the height of the particle from the vertex of the funnel.
- r be the radius of the horizontal circle described by the particle.
tanθ=rh
For vertical equilibrium, the vertical component of the normal force must balance the weight of the particle:
Nsinθ=mg(1)
Ncosθ=mv2r(2)
Dividing equation (1) by equation (2):
NsinθNcosθ=mgmv2r
This simplifies to:
tanθ=grv2
From the geometry, we can express r in terms of h:
r=htanθ
tanθ=g(htanθ)v2
Rearranging gives:
tan2θ=ghv2
Substituting v=0.5m/s and g=10m/s2:
h=v2tan2θg
Substituting v2=(0.5)2=0.25:
h=0.25tan2θ10
h=0.025tan2θ
From the previous equations, we know:
tanθ=grv2=10r0.25=40r
Substituting r=htanθ:
tan2θ=ghv2=10h0.25=40h
Substituting tan2θ into the equation for h:
h=0.025×40h
This gives:
h=1h
This indicates that we need to find a specific value for h.
Using the earlier derived relationship:
h=0.2510=0.025m=2.5cm
2.5cm
Similar Questions
A particle describes a horizontal circle of radius r on the smooth surface of an inverted cone as shown. The height of plane of circle above vertex is h. The speed of particle should be
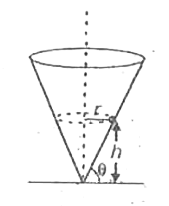 View Solution
View SolutionA particle describes a horizontal circle on the smooth surface of an inverted cone, the height of the plane of the circle above the vertex is 9.8 cm. Find the speed of the particle (g= 9.8m/s2) [See Fig.] (a) given in the answer section]
View SolutionA particle of mass m describes a circle of radius r . The centripetal acceleration of the particle is 4r2 . What will be the momentum of the particle ?
View SolutionA cylinder rolls on a horizontal plane surface. If the speed of the centre is 25 m/s, what is the speed of the highest point?
View SolutionA particle is describing circular motion in a horizontal plane in contact with the smooth surface of a fixed rigid circular cone with its axis vertical and vertex down. The heigh of the plane of motion above the vertex is hh and the semi-vertical angle of the cone is α The period of revolution of the particle
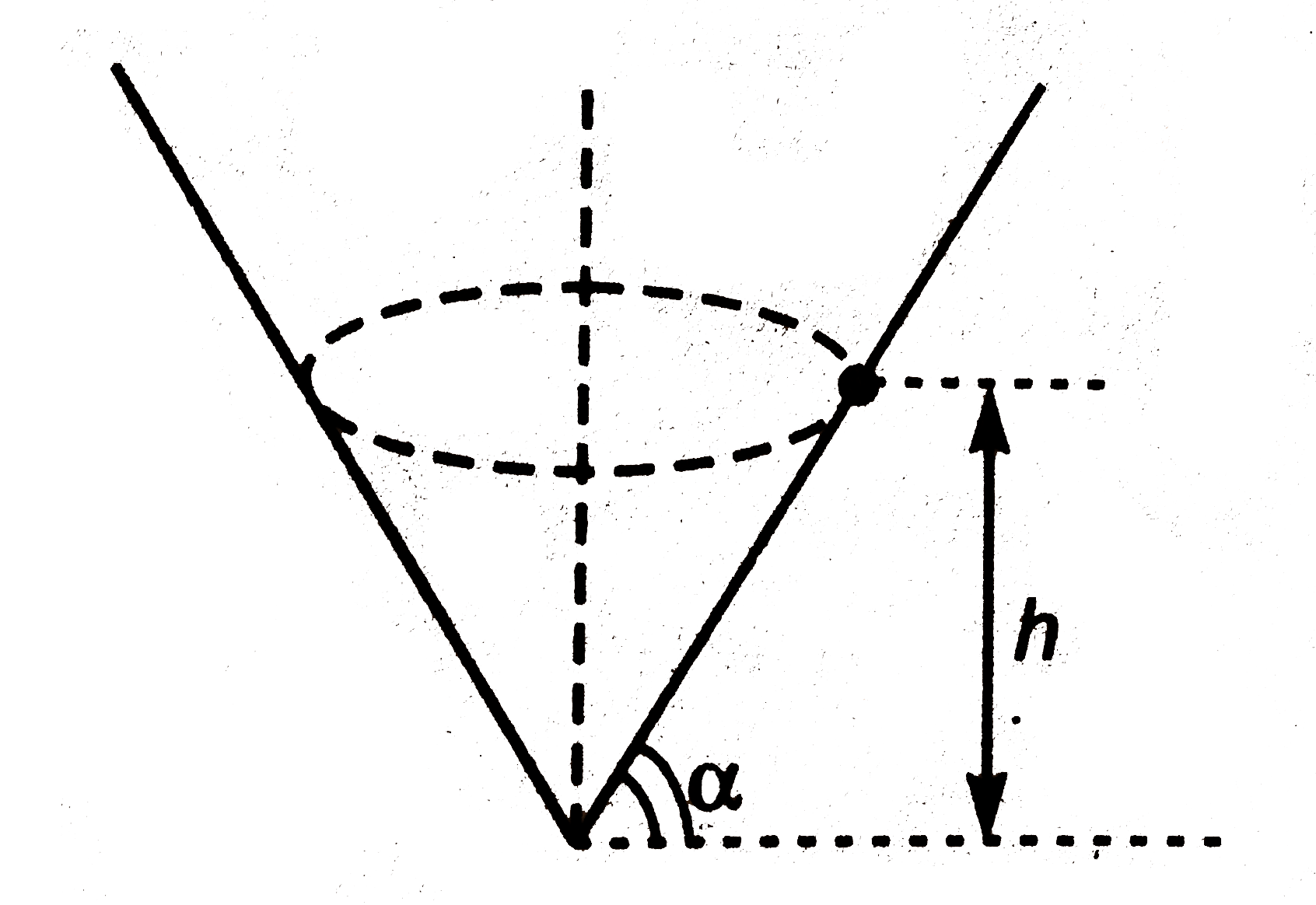 View Solution
View SolutionA metal bob of mass 0.6 kg is attached to the end of a string of length 1 m and the bob is whirled in a horizontal circle with a uniform speed of 12 m/s. What is the centripetal force acting on the bob ? If the speed of the bob is 5 m/s calculate the tension in the string.
View SolutionA particle of mass 1kg is moving about a circle of radius 1m with a speed of 1m/s. Calculate the angular momentum of the particle.
View SolutionA particle of mass 1kg is moving about a circle of radius 1m with a speed of 1m/s. Calculate the angular momentum of the particle.
View SolutionA particle of mass m is thrown horizontally from point P , as shown in the figure, with speed 5m/s. It strikes an inclined plane perpendicularly as shown. The inclination of the plane is 30∘ with the horizontal. Then, the height h shown in the figure is ( Take g=10m/s2 ) .
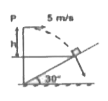 View Solution
View SolutionA particle moves in a circle of radius 5 cm with constant speed and time period 0.2πs. The acceleration of the particle is
View Solution

