The wave function orbital of H-like atoms is given as onder
ψ2s=14√2πZ3/2(2−Zr)Zr/2
Given that the radius is in Å then which of the following is the radius for nodal surface for HeΘ ion ?
The correct Answer is:1
To find the radius for the nodal surface for the He+ ion using the given wave function orbital, we can follow these steps:
1. Understand the Wave Function: The wave function for the 2s orbital of H-like atoms is given by:
ψ2s=14√2πZ3/2(2−Zr)Zr/2
where Z is the atomic number and r is the radius in Ångströms.
2. Identify the Condition for Nodal Surfaces: For nodal surfaces, the wave function must equal zero. Therefore, we set:
ψ2s=0
3. Set the Wave Function to Zero: From the wave function, we have:
2−Zr=0
This implies:
2=Zr
4. Substitute the Atomic Number for Helium: The atomic number Z for helium (He) is 2. Substituting this value into the equation gives:
2=2r
5. Solve for r: Rearranging the equation to find r:
r=22=1 Å
6. Conclusion: The radius for the nodal surface for the He+ ion is:
r=1 Å
Final Answer:
The radius for the nodal surface for the He+ ion is 1 Å.
ψ2s=14√2πZ3/2(2−Zr)Zr/2
where Z is the atomic number and r is the radius in Ångströms.
ψ2s=0
2−Zr=0
This implies:
2=Zr
2=2r
r=22=1 Å
r=1 Å
The radius for the nodal surface for the He+ ion is 1 Å.
Similar Questions
The wave function orbital of H-like atoms is given as order
ψ2s=14√2πZ3/2(2−Zr)eZr/2
Given that the radius is in Å then which of the following is the radius for nodal surface for HeΘ ion ?
View SolutionA wave function for an atomic orbital is given as Ψ2,1,0 Recogine the orbital
View SolutionConsider ψ (wave function) of 2s atomic orbital of H-atom is-
ψ2s=14√2πa3/20[2−ra0]e.r2a0
Find distance of radial node from nucleous in terms of a0
View SolutionConsider ψ (wave function) of 2s atomic orbital of H-atom is-
ψ2s=14√2πa3/20[2−ra0]e.r2a0
Find distance of radial node from nucleous in terms of a0
View Solution(a) The wave function of an electron in 2s orbital in hydrogen atom is given below:
ψ2s=14(2π)1/2(za0)3/2(2−ra0)exp(−r/2a0)
where a0 is the radius. This wave function has a radial node at r=r0. Express r0 in terms of a0.
(b) Calculate the wavelength of a ball of mass 100g moving with a velocity of 100ms−1.
View SolutionThe Schrodinger wave equation for hydrogen atom is Ψ2s=14√2π(1a0)3/2(2−ra0)e−r/a0 , where a0 is Bohr's radius . If the radial node in 2s be at r0 , then r0 would be equal to :
View SolutionIf 2cosθ+2√2=3secθ where θ∈(0,2π) then which of the following can be correct ?
View SolutionOrbitals are the pictorial representationofΨ or Ψ2

Ψ2 tell about the probability of finding electron.
Which of the following graph is correct for 3p ?
View SolutionIf the radius of the second orbit of hydrogen atom is a Å then radius of the first orbit of Li2+ ion (in Å ) will be
View SolutionWhich of the following plots of radial - probability function 4π2ψ2 is /are correct
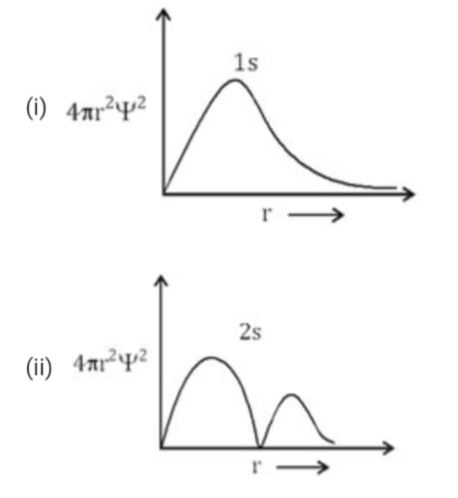
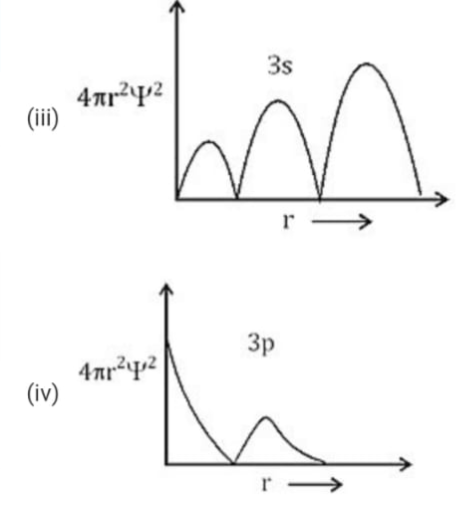
View Solution
