A wave function for an atomic orbital is given as Ψ2,1,0 Recogine the orbital
The correct Answer is:Ψn,l,m=Ψ2,1,0
n=2,l=1,m=0
l=1psubshelln=2Lshellm=0pzorbital
To recognize the orbital represented by the wave function Ψ2,1,0, we will analyze the quantum numbers provided in the notation.
1. Identify the Quantum Numbers:
The wave function Ψ2,1,0 corresponds to three quantum numbers:
- n=2 (Principal quantum number)
- l=1 (Azimuthal quantum number)
- m=0 (Magnetic quantum number)
2. Determine the Principal Quantum Number n:
The principal quantum number n indicates the energy level or shell of the electron. Here, n=2 means the electron is in the second energy level.
3. Determine the Azimuthal Quantum Number l:
The azimuthal quantum number l defines the shape of the orbital:
- l=0 corresponds to an s orbital
- l=1 corresponds to a p orbital
- l=2 corresponds to a d orbital
Since l=1, we conclude that the orbital is a p orbital.
4. Determine the Magnetic Quantum Number m:
The magnetic quantum number m specifies the orientation of the orbital:
- For l=1 (p orbital), m can take the values -1, 0, or +1.
Here, m=0 indicates the specific orientation of the p orbital along the z-axis, which is commonly referred to as the 2pz orbital.
5. Final Recognition of the Orbital:
Based on the values of n, l, and m, we can identify the orbital as 2pz.
Conclusion:
The wave function Ψ2,1,0 corresponds to the 2pz orbital.
---
The wave function Ψ2,1,0 corresponds to three quantum numbers:
- n=2 (Principal quantum number)
- l=1 (Azimuthal quantum number)
- m=0 (Magnetic quantum number)
The principal quantum number n indicates the energy level or shell of the electron. Here, n=2 means the electron is in the second energy level.
The azimuthal quantum number l defines the shape of the orbital:
- l=0 corresponds to an s orbital
- l=1 corresponds to a p orbital
- l=2 corresponds to a d orbital
Since l=1, we conclude that the orbital is a p orbital.
The magnetic quantum number m specifies the orientation of the orbital:
- For l=1 (p orbital), m can take the values -1, 0, or +1.
Here, m=0 indicates the specific orientation of the p orbital along the z-axis, which is commonly referred to as the 2pz orbital.
Based on the values of n, l, and m, we can identify the orbital as 2pz.
The wave function Ψ2,1,0 corresponds to the 2pz orbital.
Topper's Solved these Questions
Similar Questions
Knowledge Check
2s and 2p- atomic orbitals combine to give how many molecular orbitals ?
A2B4C8D6
In a hydrogen atom, which orbital is higher in energy than a 3s-orbital?
View Solution(a) The wave function of an electron in 2s orbital in hydrogen atom is given below:
ψ2s=14(2π)1/2(za0)3/2(2−ra0)exp(−r/2a0)
where a0 is the radius. This wave function has a radial node at r=r0. Express r0 in terms of a0.
(b) Calculate the wavelength of a ball of mass 100g moving with a velocity of 100ms−1.
View SolutionConsider ψ (wave function) of 2s atomic orbital of H-atom is-
ψ2s=14√2πa3/20[2−ra0]e.r2a0
Find distance of radial node from nucleous in terms of a0
View SolutionConsider ψ (wave function) of 2s atomic orbital of H-atom is-
ψ2s=14√2πa3/20[2−ra0]e.r2a0
Find distance of radial node from nucleous in terms of a0
View SolutionThe wave function of 3s and 3pz orbitals are given by :
Ψ3s=19√3(14π)1/2(Zσ0)3/2(6−6σ+σ)e−σ/2
Ψ3pz=19√6(34π)1/2(Zσ0)3/2(4−σ)σe−σ/2cos0,
σ=2Zrnα0
whereα0=1st Bohr radius , Z= charge number of nucleus, r= distance from nucleus.
From this we can conclude:
View SolutionWave function of an orbital is plotted against the distance from nucleus. The graphical representation is of :
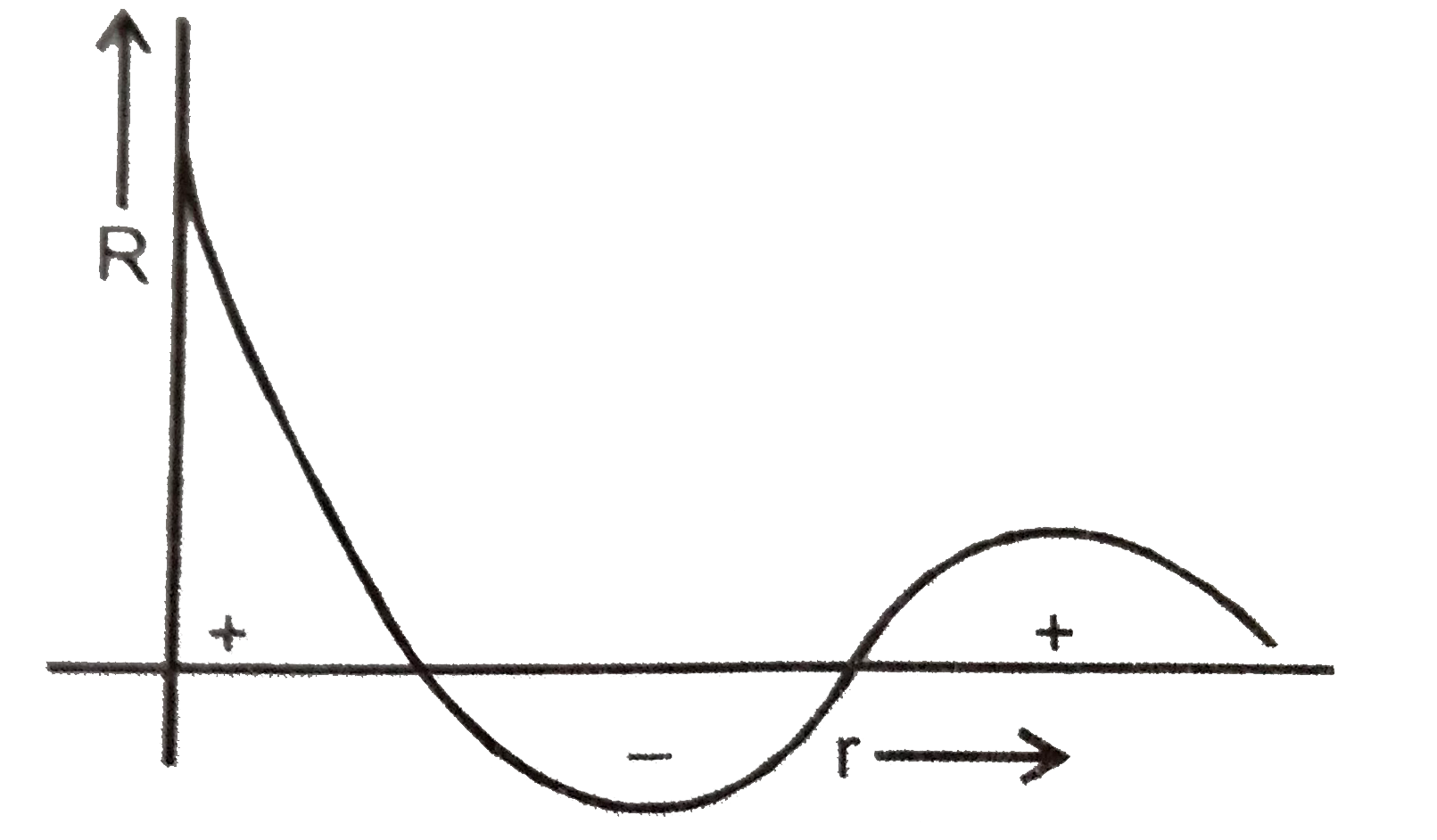
View SolutionWave function of an orbital is plotted against the distance from nucleus
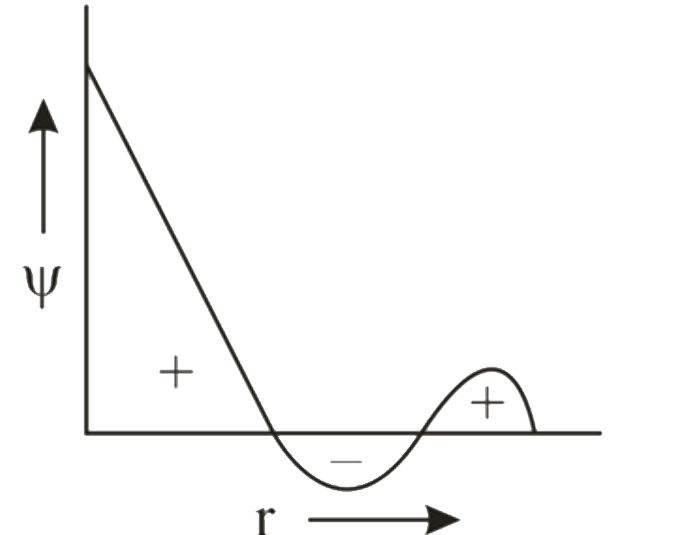
The graphical representation is of
View Solution
