A sample of .210Po which is α -emitter with T12=138 days is observed by a student to have 200 disintegration (2000 Bq) . The activity in μCi for this source is
0.050μCi
0.051μCi
0.055μCi
0.054μCi
The correct Answer is:D
To solve the problem of converting the activity of a sample of Polonium-210 from Becquerels to microcuries, we can follow these steps:
1. Understand the Given Information:
- The half-life of Polonium-210 (T₁/₂) is 138 days.
- The activity of the sample is given as 2000 disintegrations per second (2000 Bq).
2. Convert Becquerels to Curie:
- We know that 1 Curie (Ci) is equivalent to 3.7×1010 disintegrations per second (Bq).
- To convert from Becquerels to Curie, we use the formula:
Activity in Curie=Activity in Bq3.7×1010
3. Calculate the Activity in Curie:
- Substitute the given activity into the formula:
Activity in Curie=2000 Bq3.7×1010
- Performing the calculation:
Activity in Curie=20003.7×1010≈5.405×10−8 Ci
4. Convert Curie to Microcurie:
- Since 1 microcurie (µCi) is 10−6 Curie, we can convert the activity from Curie to microcurie:
\text{Activity in µCi} = \text{Activity in Ci} \times 10^{6}
- Substitute the value we calculated:
Activity in µCi=5.405×10−8 Ci×106≈0.05405 µCi
5. Final Result:
- Rounding to three significant figures, the activity of the sample in microcuries is approximately:
Activity≈0.054 µCi
Conclusion:
The activity of the Polonium-210 sample is approximately 0.054 µCi.
---
- The half-life of Polonium-210 (T₁/₂) is 138 days.
- The activity of the sample is given as 2000 disintegrations per second (2000 Bq).
- We know that 1 Curie (Ci) is equivalent to 3.7×1010 disintegrations per second (Bq).
- To convert from Becquerels to Curie, we use the formula:
Activity in Curie=Activity in Bq3.7×1010
- Substitute the given activity into the formula:
Activity in Curie=2000 Bq3.7×1010
- Performing the calculation:
Activity in Curie=20003.7×1010≈5.405×10−8 Ci
- Since 1 microcurie (µCi) is 10−6 Curie, we can convert the activity from Curie to microcurie:
\text{Activity in µCi} = \text{Activity in Ci} \times 10^{6}
- Substitute the value we calculated:
Activity in µCi=5.405×10−8 Ci×106≈0.05405 µCi
- Rounding to three significant figures, the activity of the sample in microcuries is approximately:
Activity≈0.054 µCi
The activity of the Polonium-210 sample is approximately 0.054 µCi.
Similar Questions
A radioactive sample is α−emitter with half life 138.6 days is observed by a student to have 2000 disintegration/sec. The number of radioactive nuclei for given activity are.
View SolutionThere are two radio nuceli A and B.A is an α emitter and B a β emitter. Their disintegration constant are in the ratio of 1:2 What should be the ratio of number of atoms of A and B at any time t so that probabilities of getting alpha and beta particles are same at that instant?
View SolutionA long, cylindrical iron core of cross-sectional area 5.00cm2 is inserted into a long solenoid having 2000 tunres m−1 and carrying a current 2.00A. The magnetic field inside the core is found to be 1.57T. Neglecting the end effects, find the magnetization I of the core and the pole strength developed.
View Solution(a) Calculate the activity of one mg sample .28Sr90 whose half life period is 28 years.
(b) An experiment is performed to determine the half-life of a radioactive substance which emits one beta particle for each decay process. Observation shown that an average of 8.4β−particles are emitted each second by 2.5mg of the substance. the atomic weight of the substance is 230. calculate the half0life of the substance.
(c) Determine the quantity of .84Po210 necessary to provide a source of alpha particle of 5mCi strength (T1/2 for Po =138 day).
View SolutionThe radioactivity of a sample is R1 at a time T1 and R2 at time T2. If the half-life of the specimen is T, the number of atoms that have disintegrated in the time (T2−T1) is proporational to
View SolutionThe radioactive of a sample is R1 at a time T1 and R2 at a time T2. If the half-life of the specimen is T, the number of atoms that have disintegrated in the time (T2−T1) is equal ton(R1−R2)Tln4. Here n is some integral number. What is the value of n?
View SolutionRutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
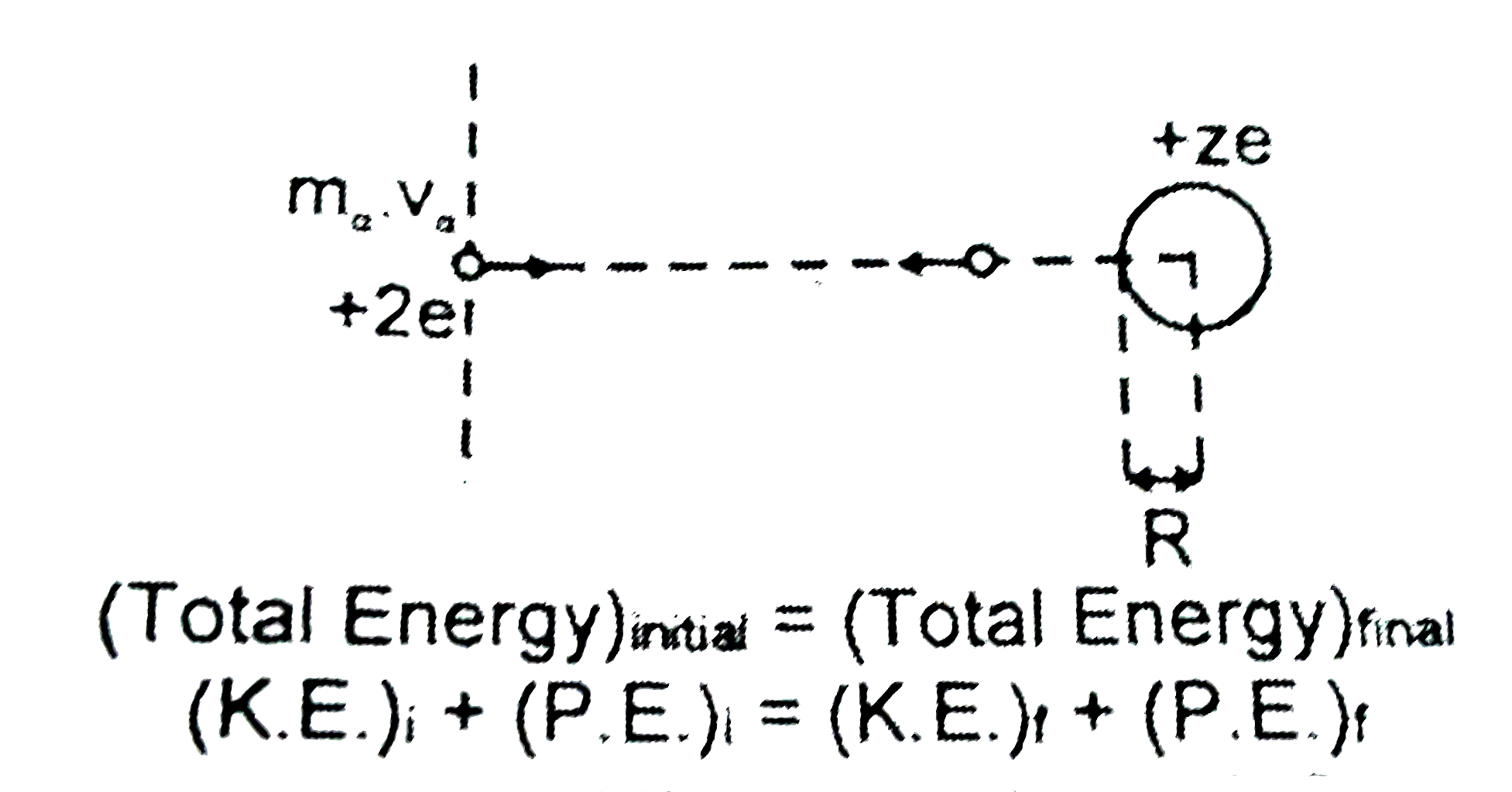
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
An α-particle with initial speed v0 is projected from infinity and it approaches up to r0 distance from a nuclie. Then, the initial speed of α-particle, which approaches upto 2r0 distance from the nucleus is :
View SolutionA student is running at her top speed of 5.0ms−1, to catch a bus, which is stopped at the bus stop. When the student is still 40.0m from the bus, it starts to pull away, moving with a constant acceleration of 0.2ms−2.
a For how much time and what distance does the student have to run at 5.0ms−1 before she overtakes the bus?
b. When she reached the bus, how fast was the bus travelling?
c. Sketch an x−t graph for bothe the student and the bus.
d. Teh equations uou used in part (a)to find the time have a second solution, corresponding to a later time for which the student and the bus are again at thesame place if they continue their specified motions. Explain the significance of this second solution. How fast is the bus travelling at this point?
e. If the students⊤speedis3.5 m s^(-1), will she catch the bus?
f. What is the minimum speed the student must have to just catch up with the bus?For what time and what distance dies she have to run in that case?
View SolutionThe path of photoelectrons emitted due to electromagnetic radiation incident on a sample of material A, is found to have a maximum bending radius of 0.1 m in a magnetic field of (√23)×10−4T. When the radiation is incident normally on a double slit having a slit separation of 0.1mm, it is observed that there are 10 fringes in a width of 3.1 cm on a screen placed at a distance of 1 m from the double slit. Find the work function of the material. and the corresponding threshold wavelength. What sould be the wavelength of the incident light so that the bending radius is one-half of what it was before? Given that mass of the electron=0.5MeVc2,hc=12400eV−A
View SolutionUranium 23892U is an unstable nucleaus. It decays to Thorium 23490Th, which is again an unstable nuclei which further decays to 23491Pa. Such decay is called successive dlsntegration. Let 23892U is called as A of decay constant λ1 and 23490Th is called as B of decay constant λ2 and stable nuclei 23491Pa is named as C. Here A is called parent nucleus and B is called daughter nucleus of A. Any two adjacent nuclei may be consider parem daughter nuclei. Suppose N1, N2 and N3 are number of nuclei A, B and C respectively at time t which undergoes decay. Then we can write
Aλ2−−→Bλ2−−→C
Rate of disintegration of A=dN1dt=−λ1N1
Rate of disintegration of B=−λ2N2 and net rate of formation of B=dN2dt=λ1N1−λ2N2
Rate of formation of nuclei C is equal to dN3dt=λ2N2
If at t=0, there are N0 number of nuclei of A, where as nuclei B and C are absent in the sample answer the following questions
The graph between N1, N2 & N3 with time can be best represented by
View Solution
