A man is running on the ground .It is known that the coefficient of friction between the man and the ground is . Then which of the following statements is correct
Normal reaction between man and ground is equal to weight of man
The direction of friction on man is in the direction of normal reaction on the man
Direction of friction on man is opposite to the direction of motion of man
Maximum acceleration of man can be
The correct Answer is:A
To solve the problem, we need to analyze the forces acting on the man who is running on the ground, considering the coefficient of friction (μ) between him and the ground.
1. Identify the Forces Acting on the Man:
- The weight of the man (W) acts downward and is equal to mg, where m is the mass of the man and g is the acceleration due to gravity.
- The normal force (N) acts upward, balancing the weight of the man.
- Since the man is running, there is a frictional force (F_friction) acting on him. This frictional force is what allows him to run without slipping.
2. Establish the Relationship Between Forces:
- In the vertical direction, the forces must balance. Thus, we have:
N=mg
- The maximum static frictional force can be expressed as:
Ffriction=μN=μmg
- This frictional force is what enables the man to accelerate forward.
3. Determine the Direction of the Frictional Force:
- The direction of the frictional force on the man is opposite to the direction of his motion. When he pushes backward against the ground, the ground pushes forward on him due to friction.
4. Analyze the Maximum Acceleration:
- The maximum acceleration (a_max) that the man can achieve without slipping can be calculated using Newton's second law:
amax=Ffrictionm=μmgm=μg
- Therefore, the maximum acceleration is not 2μg but simply μg.
5. Conclusion:
- Based on the analysis, we can conclude that:
- The normal reaction force is equal to the weight of the man (correct).
- The direction of friction is opposite to the direction of motion (correct).
- The maximum acceleration is μg (not 2μg).
Final Answer:
The correct statement is that the normal reaction force between the ground and the man is equal to the weight of the man (N=mg).
- The weight of the man (W) acts downward and is equal to mg, where m is the mass of the man and g is the acceleration due to gravity.
- The normal force (N) acts upward, balancing the weight of the man.
- Since the man is running, there is a frictional force (F_friction) acting on him. This frictional force is what allows him to run without slipping.
- In the vertical direction, the forces must balance. Thus, we have:
N=mg
- The maximum static frictional force can be expressed as:
Ffriction=μN=μmg
- This frictional force is what enables the man to accelerate forward.
- The direction of the frictional force on the man is opposite to the direction of his motion. When he pushes backward against the ground, the ground pushes forward on him due to friction.
- The maximum acceleration (a_max) that the man can achieve without slipping can be calculated using Newton's second law:
amax=Ffrictionm=μmgm=μg
- Therefore, the maximum acceleration is not 2μg but simply μg.
- Based on the analysis, we can conclude that:
- The normal reaction force is equal to the weight of the man (correct).
- The direction of friction is opposite to the direction of motion (correct).
- The maximum acceleration is μg (not 2μg).
The correct statement is that the normal reaction force between the ground and the man is equal to the weight of the man (N=mg).
Similar Questions
Which of the following statement is not true for
man?
View SolutionA car moves along a horizontal circular road of radius r with velocity u -The coefficient of friction between the wheels and the road is μ. Which of the following statement is not true?
View SolutionWhich of the following statement is correct about Neanderthal man?
View SolutionWhich of the following statement is correct about Cro-Magnon Man ?
View SolutionAn aeroplane requires a speed of 72 kmph for a take off with 150m run on the ground. The mass of the plane is 1500kg and the coefficient of friction between the aeroplane and the ground is 0.4. Assuming that the aeroplane acceleration uniformly during take off, find the minimum force exerted by the engine of the aeroplane for take off.
View SolutionA ladder of length l and mass m is placed against a smooth vertical wall, but the ground is not smooth. Coefficient of friction between the ground and the ladder is μ. The angle θ at which the ladder will stay in equilibrium is
View SolutionThe block each of mass 1kg are placed as shown .They are connected by a string which passes over a smooth (massless) pulley.
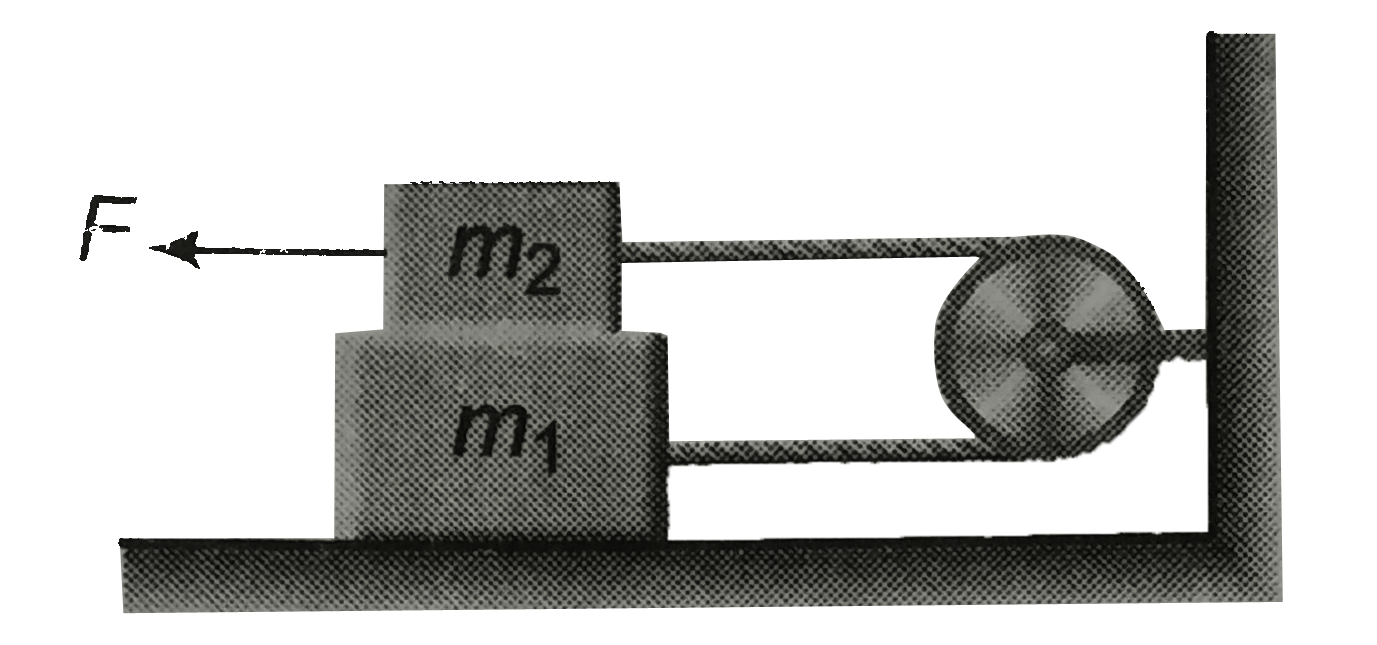
There is no friction between m1 and the ground.The coefficient of friction between m1 andm2 is 0.2A force F is applied to m2. Which of the fpllowing statement is/are correct?
View SolutionWhich of the following statement is correct w.r.t. Neanderthal man?
View SolutionA force F is applied on the top of a cube as shown in the figure. The coefficient of friction between the cube and the ground is μ. If F is gradually increased, find the value of μ for which the cube will topple before sliding.
View SolutionA block of mass m is moving with a constant acceleration a on a rough horizontal plane. If the coefficient of friction between the block and ground is μ, the power delivered by the external agent in a time interval t from the beginning is equal to:
View Solution
