An object is floating in a liquid , kept in a container . The container is placed in a lift . Choose the correct option .
Buoyant force increases as the lift accelerates up
Buoyant force decreases as the lift accelerates up
Buoyant force remains constant as the lift accelerates
The fraction of solid submerged into liquid decreases
The correct Answer is:A
To solve the problem, we need to analyze the situation of an object floating in a liquid inside a container that is placed in a lift. The key concepts involved here are buoyancy and the effects of acceleration on the effective gravitational force.
1. Understanding Floating Condition:
- An object floats in a liquid when the weight of the object (mg) is equal to the buoyant force acting on it. The buoyant force (B) can be expressed as:
B=V⋅ρl⋅g
where V is the volume of the liquid displaced, ρl is the density of the liquid, and g is the acceleration due to gravity.
2. Lift Moving Upward:
- If the lift is moving upward with a constant acceleration a, the effective gravitational force acting on the object becomes:
geffective=g+a
- The buoyant force in this case will be:
B=V⋅ρl⋅(g+a)
3. Lift Moving Downward:
- If the lift is moving downward with a constant acceleration a, the effective gravitational force acting on the object becomes:
geffective=g−a
- The buoyant force in this case will be:
B=V⋅ρl⋅(g−a)
4. Conclusion on Buoyant Force:
- Regardless of whether the lift is moving upward or downward, the buoyant force is dependent on the density of the liquid and the volume of the liquid displaced, which remains constant as long as the object is floating.
- Thus, the buoyant force remains constant even when the lift accelerates, because the volume of the liquid displaced does not change.
Final Answer:
The buoyant force acting on the object remains constant regardless of the lift's motion (upward or downward with constant acceleration).
- An object floats in a liquid when the weight of the object (mg) is equal to the buoyant force acting on it. The buoyant force (B) can be expressed as:
B=V⋅ρl⋅g
where V is the volume of the liquid displaced, ρl is the density of the liquid, and g is the acceleration due to gravity.
- If the lift is moving upward with a constant acceleration a, the effective gravitational force acting on the object becomes:
geffective=g+a
- The buoyant force in this case will be:
B=V⋅ρl⋅(g+a)
- If the lift is moving downward with a constant acceleration a, the effective gravitational force acting on the object becomes:
geffective=g−a
- The buoyant force in this case will be:
B=V⋅ρl⋅(g−a)
- Regardless of whether the lift is moving upward or downward, the buoyant force is dependent on the density of the liquid and the volume of the liquid displaced, which remains constant as long as the object is floating.
- Thus, the buoyant force remains constant even when the lift accelerates, because the volume of the liquid displaced does not change.
The buoyant force acting on the object remains constant regardless of the lift's motion (upward or downward with constant acceleration).
Similar Questions
Knowledge Check
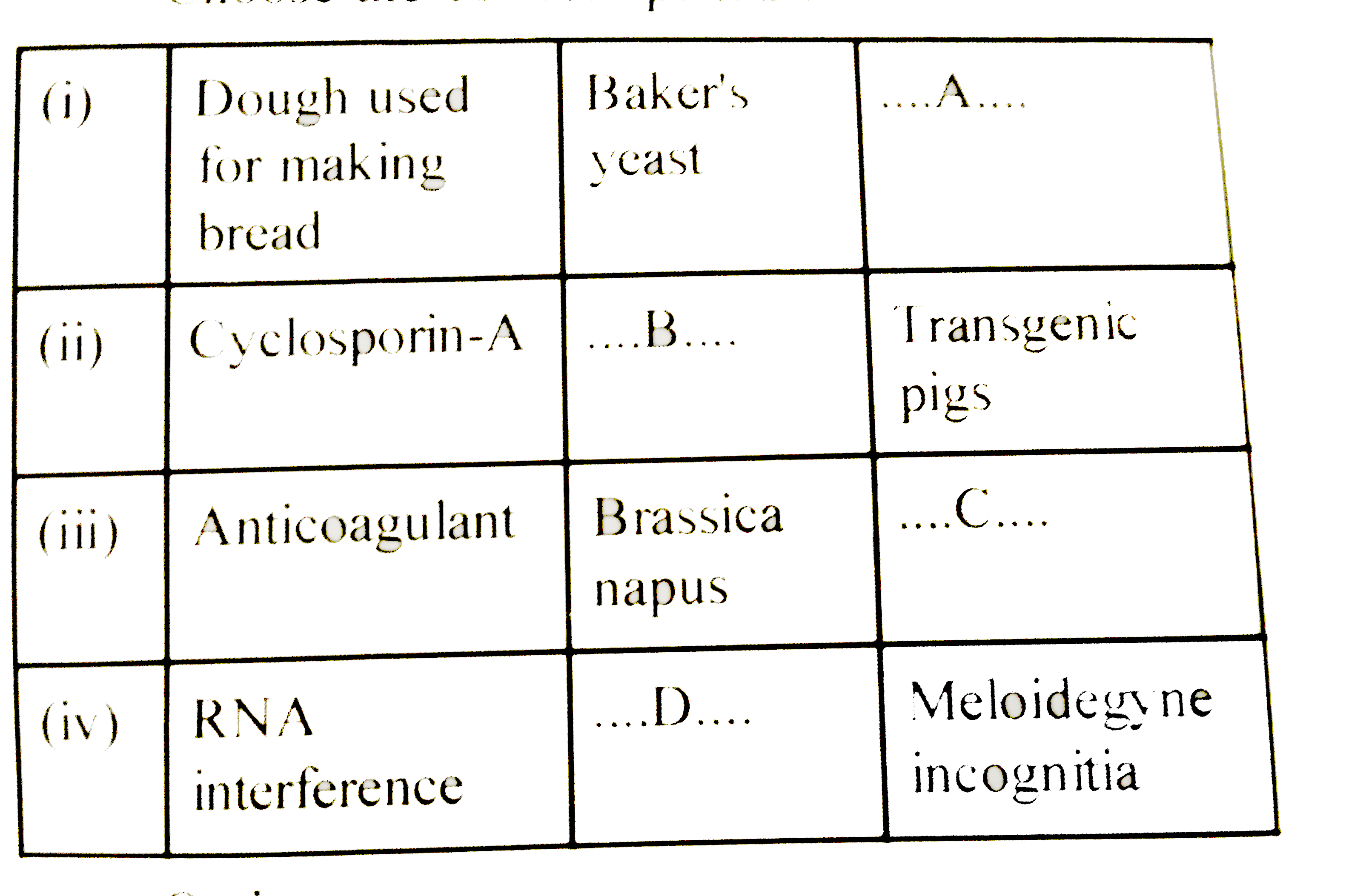
Choose the correct option as directed.
ACsH>KH>NaH>LiH (Order of stability)BH2O<NH3<CH4 (Order of dipole moment)CPH3<AsH3<NH3<SbH3 (Order of boiling point)DX−−−H−X,X=O>F>N>S>Cl (Order of strength of H-bonding)